A comparison of different methods for fitting the self-thinning equation
-
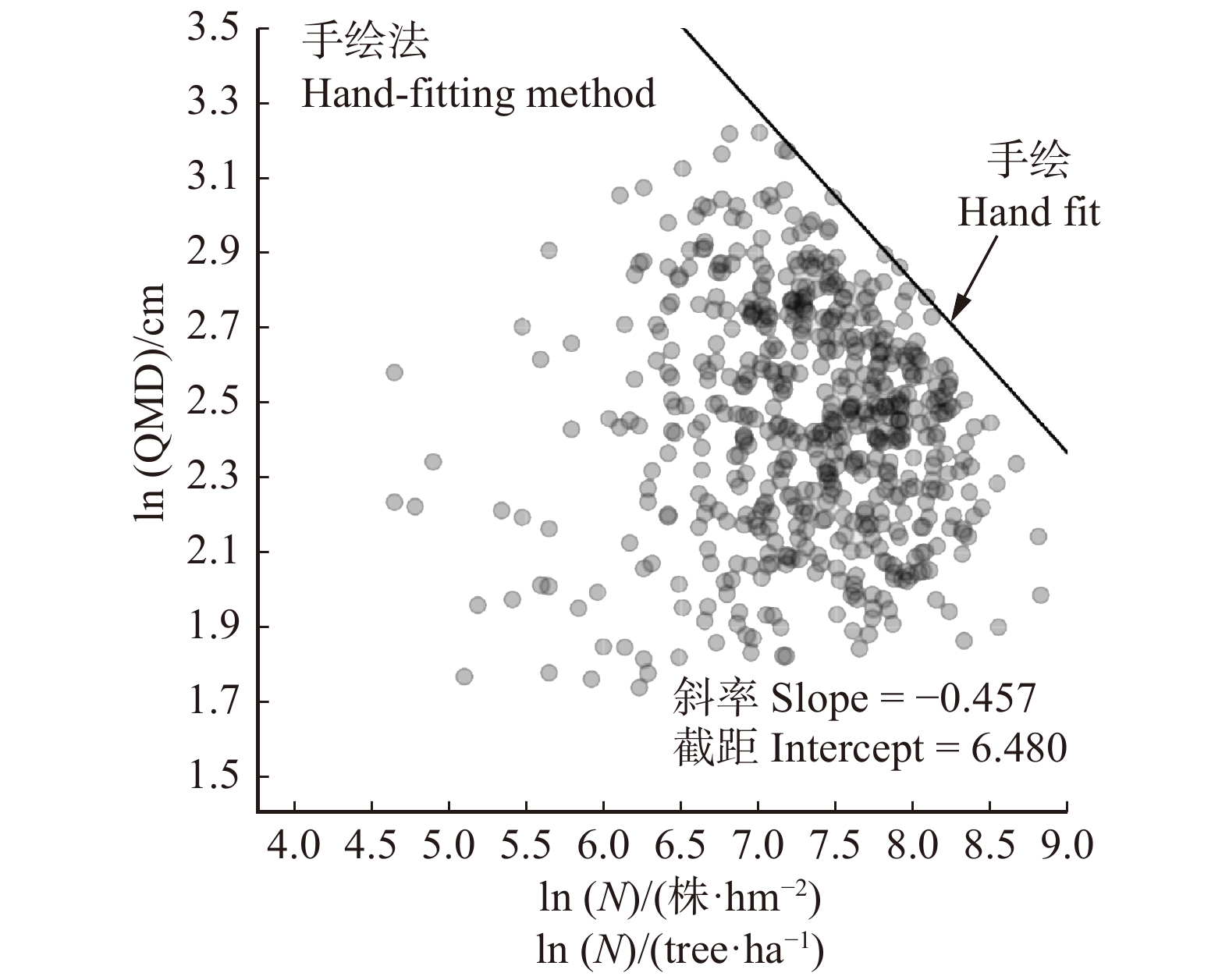
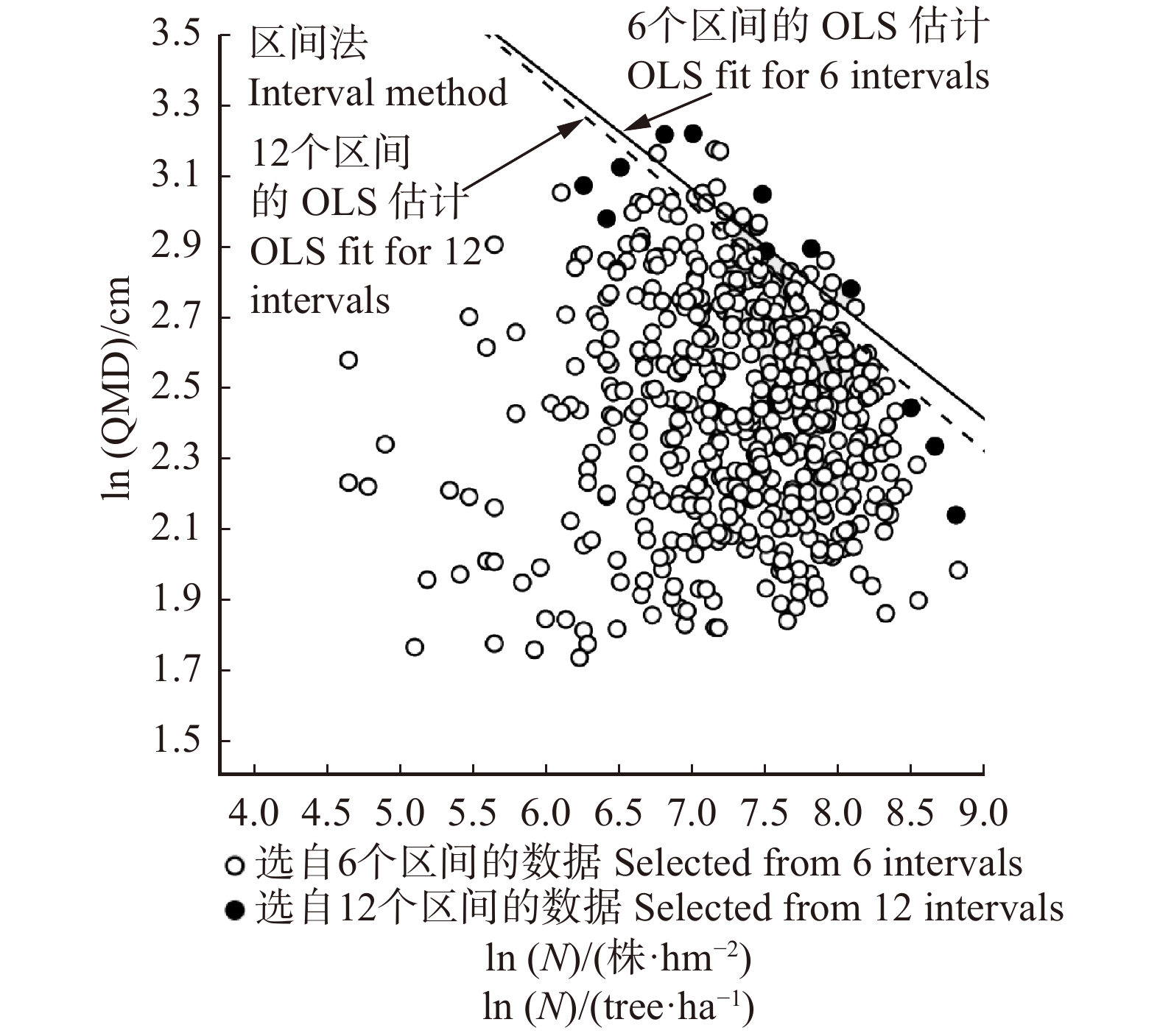
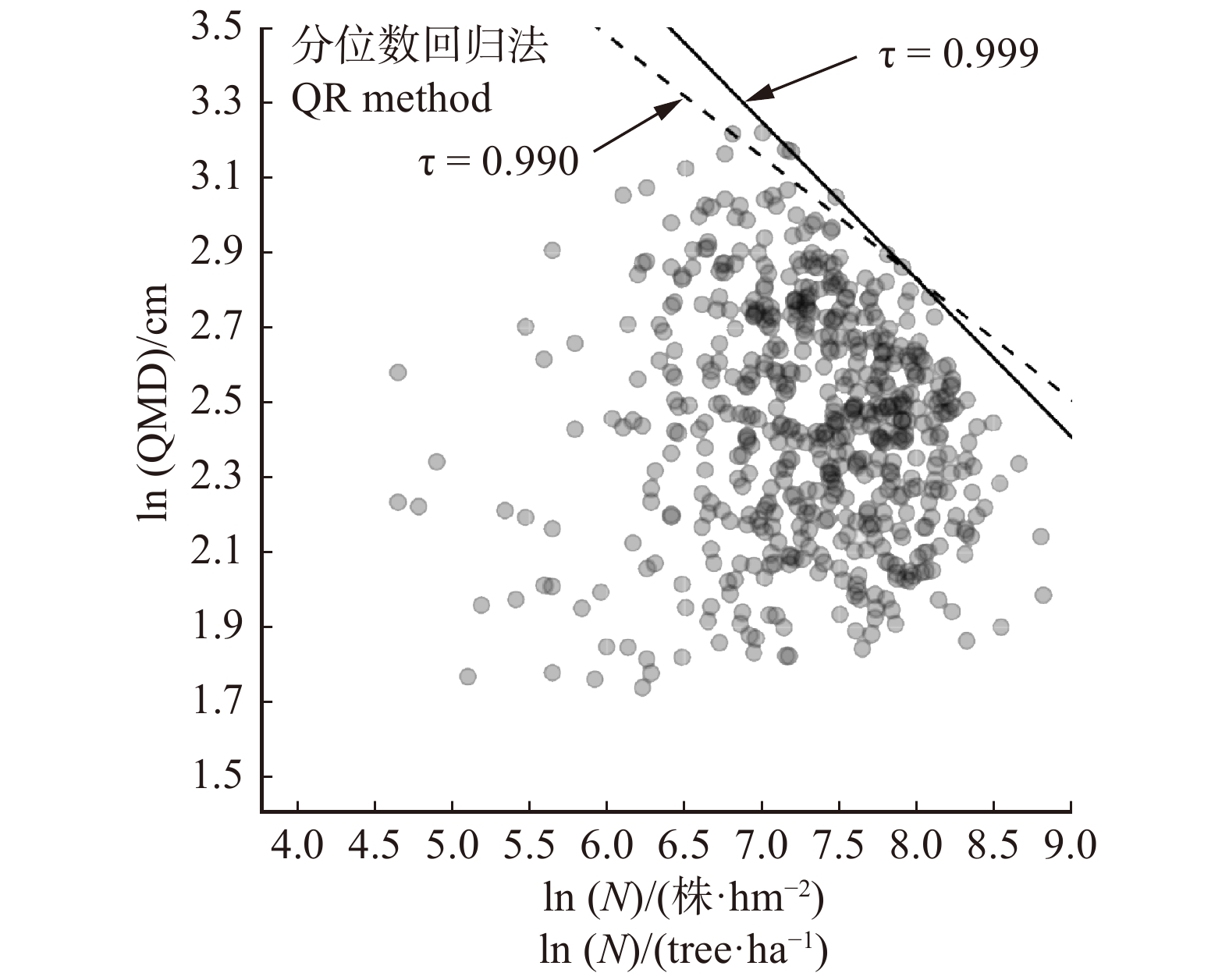
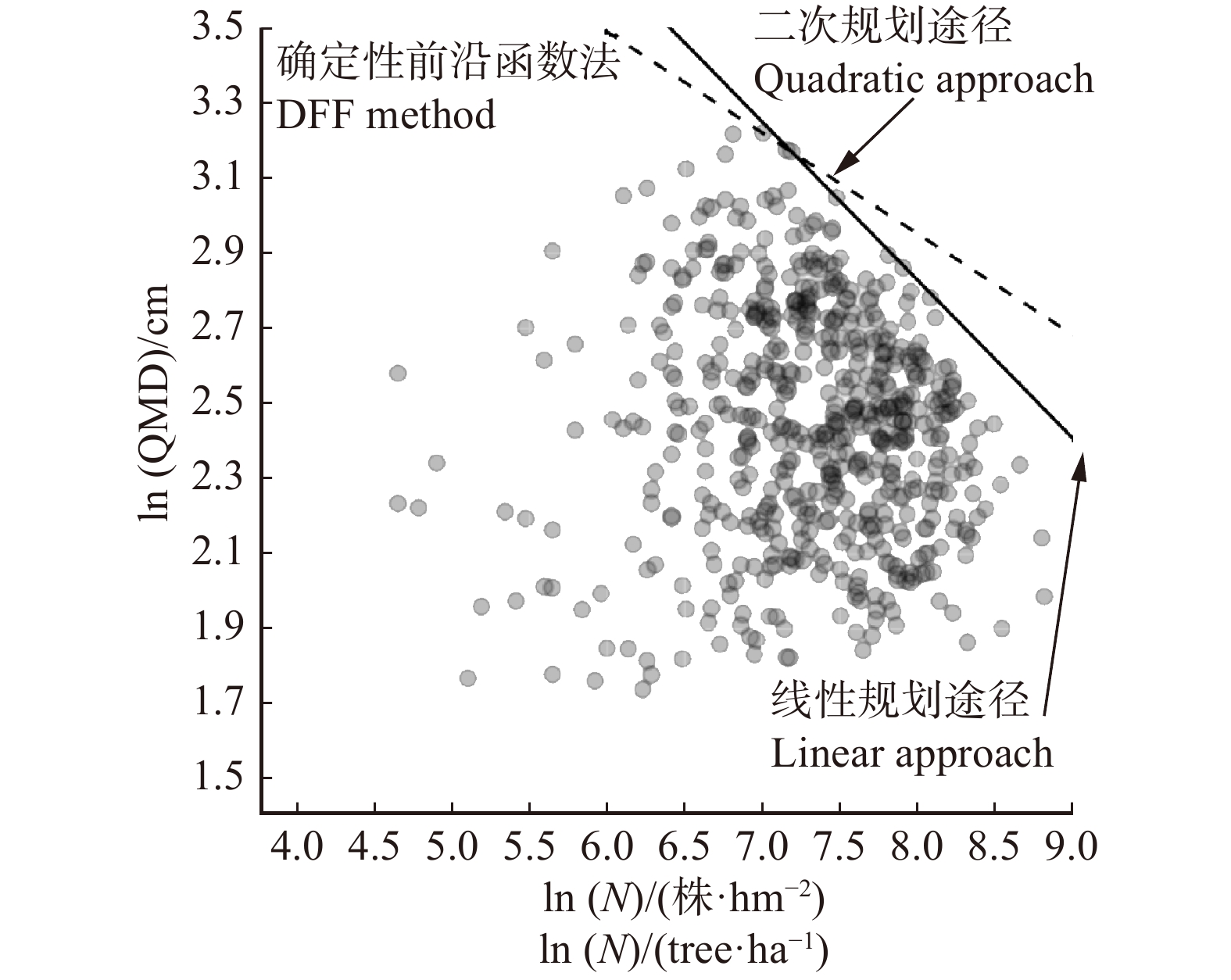
摘要:目的选择能够真实反映种群自疏动态的拟合方法是评估自然稀疏法则的难点和热点问题。方法本研究基于福建省553块杉木同龄林样地数据，对前人用于估计自然稀疏方程的手绘法、区间法和相对密度法3种传统方法，普通最小二乘法回归（OLS）、简化主轴回归（RMA）和分位数回归（QR）3种以回归为基础的方法，修正最小二乘法（COLS）、确定性前沿函数（DFF）和随机前沿函数（SFF）3种边界模型构建方法进行对比分析，得出最大密度线的适宜拟合方法。结果手绘法简单易行，但主观性较强；区间法拟合结果会受到区间长度的显著影响，得到的最大密度线斜率往往比真实斜率平缓；相对密度法能排除非密度依赖死亡的干扰，但会受选点过程中预设斜率理论值的影响；OLS法、COLS法、RMA法拟合的直线容易出现与实际的数据点边界不吻合的问题，与自然稀疏直线为数据点上边界线的定义不相符；当分位数值越接近100%，QR法拟合得到的直线就越接近林分自疏上边界线；DFF法中，采用线性规划途径拟合直线优于非线性规划途径，但QR法和DFF法进行统计推断比较困难。SFF法拟合结果比较客观，但只有随机误差项的方差足够小且趋近于零时，拟合所得直线才能真实反映种群自疏过程。结论本文最后筛选得出福建省杉木人工林最优自然稀疏方程为ln（QMD） = 7.795 − 0.620ln（N），可为当地杉木经营实践中制定有效密度调控措施提供参考。Abstract:ObjectiveFor evaluating the self-thinning theory, selecting the optimum fitting method that can truly describe self-thinning dynamics is a hot topic and a difficult task.MethodIn this study, we compared different approaches for fitting self-thinning equations, including three traditional methods, i.e., hand-fitting method, interval method and relative density (RD) method; three regression methods, i.e., ordinary least squares regression (OLS) method, reduced major axis regression (RMA) method and quantile regression (QR) method; and three frontier model methods, i.e., corrected OLS (COLS) method, deterministic frontier function (DFF) method and stochastic frontier function (SFF) method. The data from 553 sample plots of even-aged Chinese fir (Cunninghamia lanceolata) plantations in Fujian Province, eastern China was employed.ResultThe results indicated that hand-fitting method was easy but subjective. The coefficients estimated by interval method can be influenced significantly by interval length, and the estimated slope tended to be flatter than the real slope. The RD method can avoid influence of independent-density mortality, but the result would be affected by the predetermined theoretical constant for the slope. The maximum size-density lines fitted by the OLS, RMA and COLS methods tended to inaccurately match the actual boundaries of data points, and differed from the stand self-thinning upper boundary line. The maximum size-density line fitted by QR method can be close to the stand self-thinning upper boundary line when the quantile value approached 100%. The maximum size-density line fitted by linear programming approach was more suitable than the line fitted by quadratic programming approach. However, statistical inference was very difficult with the DFF and QR methods. SFF method was relatively objective, however, the fitted maximum size-density line can truly describe self-thinning process only when variance of stochastic error terms was small enough and close to zero.ConclusionFinally, the optimum self-thinning equation for Chinese fir plantations in Fujian Provence is determined as ln(QMD) = 7.795 − 0.620ln(N), which can provide a reference for developing efficient measures of stand density control.
-
Keywords:
- self-thinning theory /
- Chinese fir /
- stand density /
- comparison of different methods
-
城市生态文明建设是国家生态文明战略的重要组成部分。随着城市生态文明建设事业的快速发展，建成区绿地在绿量和绿视率方面成效显著，大众对城市绿地景观的要求和审美水平也在不断提高。植物及其形成的景观是城市生态文明的重要体现，当前城市生态系统中，植物占据极其重要地位，承担着改善城市人居环境、丰富植物多样性[1-2]、维持城市生态系统稳定等功能。现如今，城市绿地建设已不仅单纯满足于对绿量追求，而且更加重视植物景观质量的提升，植物景观的色彩变化和特色主题营造已成为当前城市绿地建设发展的新趋势[3]。
我国植物资源丰富，可作为园林应用的潜在树木种类就达8 000种以上，而草本植物资源更加丰富，对提高我国城市绿地生物多样性具有先天的资源优势[3-4]。近年来，我国相关专家学者对植物资源调查和开发利用愈发关注[5-12]，特色珍稀植物资源的开发[13]和推广应用的力度不断加大[5,14-19]，对特色植物资源调查、筛选、评价体系构建[20-21]，以及资源开发和园林应用一直是学者们和园林行业从业者的研究热点和关注焦点[22-28]。随着国家生态文明战略的确立和不断深入发展，城市绿地的彩化、香化、特色化等高品质化的诉求不断增强。在众多植物资源中，具有鲜明特色[29-31]尤其是具有较高彩化价值的本地特色植物资源颇受关注[32]。舟山群岛因其独特于内陆的海岛特征、地理位置和气候差异，分布着丰富的海岛特色彩化植物资源，有待开发生产和推广应用[33]。因此，对舟山海岛彩化植物资源进行全面调查、筛选、客观评价，为其推广应用奠定良好的基础，可为舟山的城市绿地彩化建设，以及舟山海岛特色的园林植物资源开发利用提供基础资料和理论评价依据，为我国植物资源开发和应用提供参考。
1. 研究地概况和研究对象界定
1.1 研究地概况
舟山群岛位于长江口南测、杭州湾外缘的东海海域，由嵊泗列岛、马鞍列岛、崎岖列岛、川湖列岛、中街山列岛、浪岗山列岛、七姊八妹列岛、火山列岛和梅散列岛组成。地理坐标介于121°30′ ~ 123°25′ E，29°32′ ~ 31°04′ N。东濒太平洋，南接象山县海界，西临杭州湾，北与上海市海界相接。境域东西长182 km，南北宽169 km，总面积2.22万 km2，其中海域面积2.08万 km2。舟山群岛是由3 190余个海岛组成的我国第一大群岛[34]，是我国重点海洋旅游区域和国家旅游综合改革试点城市[35]，同时确立了打造“海上花园城市”的生态发展目标 [36]。
1.2 研究对象界定
植物景观彩化是指根据植物生物学特性，利用不同植物的花、叶、果、皮等色相差异、季相变化、空间结构、视觉效果的变化，通过植物配置，产生美感的活动过程[37]。根据《城市园林绿化评价标准》的定义，本地植物是指：原有天然分布或长期生长于本地，适应本地自然条件并融入本地自然生态系统，对本地原生生物物种和生物环境不产生威胁的植物。主要包括：本地自然生长的野生植物及其衍生品种、归化种（非本地原生，但已逸为野生）及其衍生品种、驯化种（非本地原生，但在本地正常生长，并且完成其生活史的植物种类）及其衍生品种[38]。因此，舟山本地彩化植物是指原产舟山本地或者已成为舟山归化、驯化的植物种类或品种，是花、叶、果、枝（皮）长年具有除绿色外的长年或季节性的色彩变化的一类植物统称。
2. 研究方法
2.1 调查范围以及筛选方法
本研究对舟山市域范围内的城市建成区绿地和主要海岛天然植被进行实地调查，通过采集标本、拍照等方法记录植物的相关信息，并查阅《浙江植物志》以及相关文献进行考证[39]，结合以下6个原则筛选出具有园林应用前景的66种舟山本地彩化植物。
2.2 筛选原则
2.2.1 舟山本地植物且有色彩或色彩变化
原有自然分布或长期生长于舟山本地，适应本地自然条件并融入本地自然生态系统，对本地原生环境不产生威胁的植物。这类植物的花、叶、果或枝等部位常年或者在特定的季节呈现出绿色以外的色彩。
2.2.2 有一定的苗木资源或具有开发价值
筛选出的植物在舟山市内外有较丰富的苗木资源，或者目前虽尚未有苗圃生产，但其本身的观赏价值及生态习性具备舟山城市园林绿地的应用潜力，且具有较大的开发价值的种类，如芫花（Daphne genkwa）、普陀狗娃花（Aster arenarius）、长萼瞿麦（Dianthus longicalyx）等。
2.2.3 适应性强且观赏价值较高
具有较好的适应性，如耐盐碱、抗海风、抗海雾、耐瘠薄、抗病虫害、耐水湿等特点。此外具有独特的观赏价值，如花色、叶色或果色鲜艳奇特，如黄连木（Pistacia chinensis）等。
2.2.4 具有舟山的历史文化寓意和特色
舟山历史悠久，作为我国重要的佛教圣地，宗教文化底蕴深厚，部分植物具有典型的佛教文化寓意，如南京椴（Tilia miqueliana）、石蒜（Lycoris radiate）等植物。
2.2.5 有一定数量的古树资源分布
古树指在舟山本地生长百年以上的树木，能在舟山生长百年以上，说明其已适应了舟山的气候和环境，如银杏（Ginkgo biloba）等。
2.2.6 舟山海岛地域特色、特有种类
特有植物是舟山地域植物景观特色的重要体现，如舟山新木姜子（Neolitsea sericea）、匙叶紫菀（Aster spathulifolius）等，这类植物的规模化生产和应用有助于形成舟山的地域植物景观特色。
2.3 AHP层次评价法
本研究运用AHP综合评价法对筛选出的66种本地彩化植物进行评价。根据舟山本地彩化植物的特点，征求风景园林、林学等方面的专家以及园林行业的工作者的意见和建议的基础上，通过调查问卷和专家打分的形式，确定了基本能够全面衡量和评价彩化植物在舟山应用的18个评价指标，根据其隶属关系，建立客观、合理的层次评价模型。
2.3.1 构建层次模型
模型包括目标层OB（舟山本地彩化植物综合评价）、准测层A（美学价值、生态适应性、栽培管护特性、生态价值）、指标层B（树形、叶形、花形数等18个综合评价舟山本地彩化植物应用的因素）、方案层C（待评价的彩化植物），各层次之间互不相交（图1）。
![]()
图 1 舟山本地彩化植物综合评价模型Figure 1. Comprehensive evaluation model of local colourful plants in Zhoushan Archipelago2.3.2 判断矩阵的构建及权重的确定
根据总目标的要求，在参考有经验的园林专业人士意见的基础上做出判断。本模型以1~9标度法构造判断矩阵，由此得出OB-A（第二层因素相对于第一层的比较判断）、A-B（第三层因素相对于第二层的比较判断）共5个矩阵。相关公式如下：
CI=(λmax (1) {\rm{CR = CI/RI}} (2) 式中：CI为一致性指标，λmax为判断矩阵相应行列式的非零最大特征根，CR为随机一致性比率，RI为判断矩阵的平均随机一致性指标。
其中，1~9阶的判断矩阵的RI值分别为0、0、0.52、0.89、1.12、1.26、1.36、1.41和1.46。作一致性的检验，需计算CI然后将CI与RI进行相互比较计算CR，若CR < 0.1，则判断该矩阵具有满意的一致性[40]。根据迈实软件（Version1.82）完成相应的计算与检验，该软件可生成相应的专家调查表格，并可实现数据的批量处理，具有较强的实用性与数据可靠性。
2.3.3 评分标准建立
本研究的评价人员均为有相关知识背景和实践经验的专业人员，对舟山本地彩化植物具有良好的认知和评判能力[40]。采用5分评分标准邀请参评人员评价标准层指标，计算得出总分，从而进行分级评价（表1）。最终得出各植物18个指标的得分Ci（i = 1, 2, ···, 18）。同时采用层次分析法和加权平均法获得各影响因素的平均权重（Wi），运用以下公式计算各植物的综合评价值（Tj）。
表 1 舟山本地彩化植物评价指标和评价标准Table 1. Evaluation indexes and standards of local colourful plants in Zhoushan Archipelago评价指标 Evaluation index 分值（0~5） Score（0−5） A1 B1
B2树形差、松散至树形美、紧凑
From poor tree shape, loose to beautiful tree shape, compact叶小、形差、松散至叶大、形美、紧密
From small leaf, poorly shaped, loose to large, beautiful and compactB3 花小、花少、花色单一至花大、花多、花色丰富
From small flower, few flower, single color to large flower, many flower, and rich colorB4 叶色变化单一至叶色变化丰富
Leaf color changes from single to richB5 15 d以下、16 ~ 20 d、21 ~ 25 d、25 ~ 30 d、30 d以上
Less than 15 d, 16−20 d, 21−25 d, 25−30 d and more than 30 dA2 B6 差、较差、中等、较强、强
Poor, inferior, middle, stronger, strongestB7 B8 B9 B10 B11 B12 B13 A3 B14 差、较差、中等、较强、强
Poor, inferior, middle, stronger, strongestB15 A4 B16 差、较差、中等、较强、强
Poor, inferior, middle, stronger, strongestB17 B18 {\rm{Tj}} = \sum {{C_i}{W_i}} (3) 2.3.4 权重分析及一致性检验
对4个判断矩阵进行一致性检验。当判断矩阵的CR < 0.1时或CI = 0时，认为判断矩阵具有满意的一致性，否则需调整矩阵中的元素以使其具有满意的一致性。
3. 结果和分析
3.1 评价模型权重设置及评价结果分析
评价因子的权重体现出该指标在评价中的相对重要性，确定各指标权重是评价的前提[40]。此处采用1~9比率标度法，对层次模型构造判断矩阵，并进行层次单类别和一致性检验以及层次总类别和一致性检验[40]。由表2、3、4、5可知：乔木、灌木、草本、藤本等4类植物CR值分别为0.031、0.087、0.013、0.066，均小于0.1一致性检验均通过。对其他3类评价模型依次计算检验，均得到满意的一致性，最终确定不同的评价指标权重[40]。在各项评分的基础上按各项得分与其权重进行计算，求得各自的综合得分，根据不同的生活型将66种彩化植物划分为3个等级：Ⅰ类（Tj ≥ 3.5）、Ⅱ类（3.5 > Tj ≥ 3.0）、Ⅲ类（Tj < 3.0）。
表 2 乔木评价模型判断矩阵及一致性检验Table 2. Judgment matrix and consistency test of tree evaluation model项目 Item A1 A2 A3 A4 Wi A1 1.000 1.000 5.000 7.000 0.452 A2 1.000 1.000 3.000 5.000 0.366 A3 0.200 0.333 1.000 3.000 0.124 A4 0.143 0.200 0.333 1.000 0.058 注：乔木评价模型中，舟山本地彩化植物综合评价OB-Ai，其中λmax = 4.082，CR = 0.031, CI = 0.027。Notes: in the arbor evaluation model, the comprehensive evaluation of Zhoushan Archipelago local colorful plants is OB-Ai, in which, λmax = 4.082, CR = 0.031, CI = 0.027. 表 3 灌木评价模型判断矩阵及一致性检验Table 3. Judgment matrix and consistency test of shrub evaluation model项目 Item A1 A2 A3 A4 Wi A1 1.000 1.000 3.000 7.000 0.417 A2 1.000 1.000 5.000 3.000 0.383 A3 0.333 0.200 1.000 3.000 0.130 A4 0.143 0.333 0.333 1.000 0.069 注：灌木评价模型中，舟山本地彩化植物综合评价OB-Ai，其中λmax = 4.233，CR = 0.087, CI = 0.078。Notes: in the shrub evaluation model, the comprehensive evaluation of Zhoushan Archipelago local colorful plants is OB-Ai, in which, λmax = 4.233, CR = 0.087, CI = 0.078. 表 4 藤本评价模型判断矩阵及一致性检验Table 4. Judgment matrix and consistency test of liana evaluation model项目 Item A1 A2 A3 A4 Wi A1 1.000 1.000 5.000 7.000 0.343 A2 1.000 1.000 1.000 9.000 0.294 A3 0.200 1.000 1.000 7.000 0.218 A4 0.143 0.111 0.143 1.000 0.145 注：藤本植物评价模型中，舟山本地彩化植物综合评价OB-Ai，其中λmax = 4.343，CR = 0.013，CI = 0.114。Notes: in the liana evaluation model, the comprehensive evaluation of Zhoushan Archipelago local colorful plants is OB-Ai, in which, λmax = 4.343, CR = 0.013, CI = 0.114. 表 5 草本评价模型判断矩阵及一致性检验Table 5. Judgment matrix and consistency test of herb evaluation model项目 Item A1 A2 A3 A4 Wi A1 1.000 0.333 1.000 9.000 0.239 A2 1.000 1.000 3.000 7.000 0.512 A3 1.000 0.333 1.000 5.000 0.206 A4 0.111 0.143 0.200 1.000 0.043 注：草本植物评价模型中，舟山本地彩化植物综合评价OB-Ai，其中λmax = 4.175，CR = 0.066，CI = 0.058。Notes: in the herbaceous plant evaluation model, the comprehensive evaluation of Zhoushan Archipelago local colorful plants is OB-Ai, where λmax = 4.175, CR = 0.066, CI = 0.058. 3.2 乔木综合评价结果
30种舟山本地彩化乔木综合评价结果如表6所示：30种彩化乔木中评价为Ⅰ类的有黄连木（Pistacia chinensis）、舟山新木姜子（Neolitsea sericea）2种，占比6.7%；评价为Ⅱ类的有海滨木槿（Hibiscus hamabo）、枫香（Liquidambar formosana）、白杜（Euonymus maackii）、全缘冬青（Ilex integra）、海州常山（Clerodendrum trichotomum）、野鸦椿（Euscaphis japonica）、乌桕（Sapium sebiferum）、檫木（Sassafras tzumu）、铁冬青（Ilex rotunda）、南川柳（Salix rosthornii）、七叶树（Aesculus chinensis）等11种，占比36.7%。综合评价为Ⅰ类和Ⅱ类的乔木，建议作为舟山园林绿地的基调植物，并作为主要苗木产品在舟山本地苗圃进行生产和应用。
表 6 舟山本地彩化乔木综合评价值Table 6. Comprehensive evaluation values of local colorful trees in Zhoushan Archipelago类别 Category 植物名
Plant name分值 Score 类别 Category 植物名
Plant name分值 Score Ⅰ 黄连木 Pistacia chinensis 3.848 Ⅲ 冬青 Ilex chinensis 2.874 Ⅰ 舟山新木姜子 Neolitsea sericea 3.530 Ⅲ 金银木 Lonicera maackii 2.755 Ⅱ 海滨木槿 Hibiscus hamabo 3.422 Ⅲ 无患子 Sapindus mukorossi 2.712 Ⅱ 枫香 Liquidambar formosana 3.372 Ⅲ 榔榆 Ulmus parvifolia 2.699 Ⅱ 白杜 Euonymus maackii 3.372 Ⅲ 豆梨 Pyrus calleryana 2.681 Ⅱ 全缘冬青 Ilex integra 3.329 Ⅲ 合欢 Albizzia julibrissin 2.681 Ⅱ 海州常山 Clerodendrum trichotomum 3.221 Ⅲ 小叶石楠 Photinia parvifolia 2.666 Ⅱ 野鸦椿 Euscaphis japonica 3.199 Ⅲ 柿树 Diospyros kaki 2.653 Ⅱ 乌桕 Sapium sebiferum 3.159 Ⅲ 南京椴 Tilia miqueliana 2.639 Ⅱ 檫木 Sassafras tzumu 3.151 Ⅲ 山茱萸 Cornus officinale 2.615 Ⅱ 铁冬青 Ilex rotunda 3.132 Ⅲ 朴树 Celtis sinensis 2.545 Ⅱ 南川柳 Salix rosthornii 3.076 Ⅲ 榉树 Zelkova schneideriana 2.503 Ⅱ 七叶树 Aesculus chinensis 3.062 Ⅲ 金钱松 Pseudolarix amabilis 2.453 Ⅲ 三角枫 Acer buergerianum 2.947 Ⅲ 银杏 Ginkgo biloba 2.326 Ⅲ 红楠 Machilus thunbergii 2.946 Ⅲ 红椿 Toona ciliata 2.324 3.3 灌木综合评价结果
对筛选出的16种备选灌木进行综合评价，结果显示（表7）：综合评价为Ⅰ类的彩化植物有白棠子树（Callicarpa dichotoma）、芫花（Daphne genkwa）、蜡梅（Chimonanthus praecox）、美丽胡枝子（Lespedeza formosa）、老鸦糊（Callicarpa giraldii）等，占比31.3%；评价为Ⅱ类的有浙江红山茶（Camellia chekiangoleosa）、中华绣线菊（Spiraea chinensis）、河北木蓝（Indigofera bungeana）、紫珠（Callicarpa bodinieri）、紫金牛（Ardisia japonica）、卫矛（Euonymus alatus）、金钟花（Forsyfhia viridissima）、溲疏（Deutzia crenata）、臭牡丹（Clerodendrum bungei）等9种，占比56.3%。这2大类植物建议在舟山园林绿地中推广应用，尤其是第Ⅰ类建议扩大苗木生产。
表 7 舟山本地彩化灌木综合评价值Table 7. Comprehensive evaluation values of local colorful shrubs in Zhoushan Archipelago类别 Category 植物名
Plant name分值 Score 类别 Category 植物名
Plant name分值 Score Ⅰ 白棠子树 Callicarpa dichotoma 3.789 Ⅱ 中华绣线菊 Spiraea chinensis 3.272 Ⅰ 芫花 Daphne genkwa 3.669 Ⅱ 河北木蓝 Indigofera bungeana 3.133 Ⅰ 蜡梅 Chimonanthus praecox 3.651 Ⅱ 紫珠 Callicarpa bodinieri 3.115 Ⅰ 美丽胡枝子 Lespedeza formosa 3.532 Ⅱ 紫金牛 Ardisia japonica 3.082 Ⅰ 老鸦糊 Callicarpa giraldii 3.504 Ⅱ 卫矛 Euonymus alatus 3.078 Ⅱ 浙江红山茶 Camellia Chekiangoleosa 3.449 Ⅱ 金钟花 Forsyfhia viridissima 3.061 Ⅱ 溲疏 Deutzia crenata 3.346 Ⅲ 紫荆 Cercis chinensis 2.888 Ⅱ 臭牡丹 Clerodendrum bungei 3.279 Ⅲ 朱砂根 Ardisia crenata 2.885 3.4 藤本综合评价结果
对筛选出的舟山本地5种本地藤本植物进行综合评价，结果如表8所示：评价为Ⅰ类的有单叶蔓荆（Vitex trifolia）和地锦（Parthenocissus tricuspidata）2种，占比40%；综合评价为Ⅱ类的有紫藤（Wisteria sinensis）和云实（Caesalpinia decapetala）2种，占比40%。这2类藤本植物均具有较好的开发应用潜力，可加大其在舟山城市园林绿地的推广和应用。
表 8 舟山本地彩化藤本综合评价值Table 8. Comprehensive evaluation values of local colorful vines in Zhoushan Archipelago类别 Category 植物名
Plant name分值 Score Ⅰ 单叶蔓荆 Vitex trifolia 3.872 Ⅰ 地锦 Parthenocissus tricuspidata 3.545 Ⅱ 紫藤 Wisteria sinensis 3.218 Ⅱ 云实 Caesalpinia decapetala 3.081 Ⅲ 忍冬 Lonicera japonica 2.908 3.5 草本综合评价结果
舟山本地草本植物评价结果显示（表9）：在15种舟山本地多年生草本中，匙叶紫菀（Aster spathulifolius）、芙蓉菊（Crossostephium chinense）、普陀狗娃花（Heteropappus arenarius）、八宝景天（Hylotelephium erythrostichum）、大吴风草（Farfugium japonicum）、佛甲景天（Sedum lineara）综合评价最好，占比40.0%；长萼瞿麦（Dianthus chinensis）、赤胫散（Polygonum runcinatum var. sinense）评价次之，占比13.3%。综合评价表明，这2类植物具有较高的开发和应用价值，建议在舟山对其加以重点推广和开发应用。
表 9 舟山本地彩化草本综合评价值Table 9. Comprehensive evaluation values of local colorful herbs in Zhoushan Archipelago类别 Category 植物名
Plant name分值
Score类别 Category 植物名
Plant name分值 Score Ⅰ 匙叶紫菀 Aster spathulifolius 4.353 Ⅲ 虎耳草 Saxifraga stolonifera 2.926 Ⅰ 芙蓉菊 Crossostephium chinense 4.327 Ⅲ 石蒜 Lycoris radiate 2.715 Ⅰ 普陀狗娃花 Heteropappus arenarius 3.852 Ⅲ 换锦花 Lycoris sprengeri 2.715 Ⅰ 八宝景天 Hylotelephium erythrostichum 3.804 Ⅲ 普陀水仙 Narcissus tazetta 2.579 Ⅰ 大吴风草 Farfugium japonicum 3.617 Ⅲ 射干 Belamcanda chinensis 2.526 Ⅰ 佛甲景天 Sedum lineara 3.611 Ⅲ 白及 Bletilla striata 2.490 Ⅱ 长萼瞿麦 Dianthus chinensis 3.337 Ⅲ 桔梗 Platycodon grandiflorus 2.244 Ⅱ 赤胫散 Polygonum runcinatum var. sinense 3.334 4. 结论和讨论
4.1 评价结果
通过调查可知,舟山群岛本地彩化植物资源丰富，地域特色鲜明，彩化植物资源推广应用和开发空间和潜力巨大[30]。根据评价结果可知：综合评分为Ⅰ类的彩化植物共15种，乔木如黄连木、舟山新木姜子；灌木如芫花、蜡梅、白棠子树、美丽胡枝子、老鸦糊等；藤本植物如单叶蔓荆和地锦；草本植物如匙叶紫菀、芙蓉菊、普陀狗娃草、八宝景天、大吴风草、佛甲景天等。这15种本地彩化植物在美学价值、生态适应性、栽培管护特性、生态价值（效益）方面综合评价最高，具有极高的开发价值，是舟山城市彩化建设中值得推广应用的本地彩化植物资源，建议对该类植物加强繁育，扩大苗木资源。
综合评价为Ⅱ类的彩化植物共24种，乔木如海滨木槿、枫香、白杜、全缘冬青、海州常山、野鸦椿、乌桕、檫木、铁冬青、南川柳、七叶树等；灌木如中华绣线菊、河北木蓝、紫珠、紫金牛、卫矛、金钟花、浙江红山茶、溲疏、臭牡丹等；藤本植物如紫藤和云实；草本植物如长萼瞿麦和赤胫散。这些彩化植物在在美学价值、生态适应性、栽培管护特性、生态价值（效益）方面综合评价相对较高，具有较好的开发前景，建议以开发和推广应用，以丰富城市植物景观色彩和彩化植物多样性。
综合评价为Ⅲ类的彩化植物共27种，乔木15种，如三角枫、冬青、金银木、无患子、榔榆等；灌木如紫荆、朱砂根；藤本植物如有忍冬；草本植物如虎耳草、石蒜、换锦花等，这类彩化植物综合评价相对较低，但在丰富城市色彩和彩化植物多样性方面亦可以发挥一定的作用。
因此，综合评价为Ⅰ类的15种彩化植物和Ⅱ类的24种彩化植物，可作为丰富舟山城市色彩或彩化植物多样性的补充彩化植物资源加以重点开发和利用。由于舟山土地资源紧张，本研究评价结果可作为生产和应用提供参考和借鉴，向长三角土地资源丰富的地区推广生产和应用。
4.2 舟山本地彩化植物应用存在的问题
通过与内陆地区彩色植物资源比较[29,31-32,41-42]可知：舟山群岛彩化植物资源的海岛特征明显，与内陆地区彩化植物资源有一定的差异，尤其是舟山特有植物资源，如舟山新木姜子、匙叶紫菀、普陀狗娃花、普陀水仙（Narcissus tazetta）、芙蓉菊等，还分布有海滨木槿、全缘冬青、长萼瞿麦、单叶蔓荆等典型的海岛植物资源。但当前舟山建成区城市绿地中，道路绿化主要行道树为香樟（Cinnamomum camphora）、广玉兰（Magnolia grandiflora）、红楠等常绿植物，其中香樟行道树占比95%以上[1]，植物景观色彩单一，缺乏季相色彩变化。悬铃木（Platanus acerifolia）、朴树、紫薇（Lagerstroemia indica）、无患子、银杏等，具有一定的色彩变化的植物占比较少，约5%[1]。基于现状的调研，城市公园绿地中常见本地彩化植物约20余种，主要有舟山新木姜子、乌桕、黄连木、海滨木槿、红楠、合欢、全缘冬青、朴树、榉树、枫香、铁冬青、大叶冬青、冬青、紫金牛、朱砂根、大吴风草、榔榆、丝棉木（白杜）、三角枫，约占本次筛选数量的30%。需要说明的是，调查中发现以上彩化植物大多只是零星应用在公园绿地中，体量较小，并未形成植物景观彩化基调，尚不能体现舟山城市绿地的植物景观特色。通过调研发现主要有以下原因：（1）舟山土地资源紧张，难以大规模生产本地彩化植物资源，致使本地特色鲜明的彩化植物难以得到较好的生产推广；（2）随着舟山城市建设的快速推进，城市园林绿地建设周期短、工期紧，在舟山本地绿地建设中，外来园林景观规划设计单位居多，对舟山本地彩化植物苗木资源的了解尚不够深入，在设计阶段主要还是以外来的常规苗木为主，进一步导致本地植物的生产开发和园林应用推广受到限制；（3）本地彩化植物资源的研究、苗木生产和推广力度总体偏弱，尚需加强。
4.3 建议和对策
目前，舟山市城市园林绿地“绿化有余、彩化不足”的问题也日渐显现，单一传统的绿化形式以及单纯的对绿量的追求已很难体现作为旅游型城市建设水平，难以满足市民和游客的审美需求[37]。园林植物作为舟山城市园林绿地建设的核心要素，在本地彩化植物资源中发挥着关键作用。微观层面上是对本地彩化植物资源进行评价和开发，并进行资源调查和综合评价，为舟山市本地彩化植物资源引种驯化研究、苗木生产和推广应用工作建立提供了研究基础，有利于促进本地彩化植物在舟山城市绿地建设中应用的良性循环。宏观层面上建议加强对建成区的绿地本地彩化植物景观的总体顶层规划研究，对城市绿地加强顶层的植物景观总体规划，目的是为对今后的城市园林彩化建设起到宏观层面的系统指导作用。一方面，城市绿地的品质提高有利于促进舟山群岛新区旅游业及其他行业的可持续发展；另一方面，由过去的“城市绿化”升级为的“城市彩化”的建设目标，不断将舟山城市生态文明建设和“海上花园城市”建设推向新高度，有利于提升舟山城市的品味，增强市民的获得感和幸福感。
致谢 本研究得到浙江农林大学风景园林与建筑学院吴仁武博士，以及李上善、张明月、朱怀真等硕士研究生的大力支持和帮助，在此一并表示感谢。
-
![]()
图 1 清查样地杉木纯林的地理分布
Figure 1. Geographical distribution of the inventory sample plots within pure Chinese fir stands
![]()
图 2 由手绘法得到的最大密度线
Figure 2. The maximum size-density line obtained from the hand-fitting method
![]()
图 3 由区间法得到的最大密度线
Figure 3. The maximum size-density lines obtained from the interval method
![]()
图 4 由相对密度（RD）法得到的最大密度线
Figure 4. The maximum size-density lines obtained from the relative density (RD) method
![]()
图 5 6种拟合方法得到的最大密度线
Figure 5. The maximum size-density lines obtained from the six fitting methods
![]()
图 6 由分位数回归（QR）法得到的最大密度线
Figure 6. The maximum size-density lines obtained from the quantile regression (QR) method
![]()
图 7 由确定性前沿分析（DFF）法得到的最大密度线
Figure 7. The maximum size-density lines obtained from the deterministic frontier function (DFF) method
表 1 杉木人工纯林林分和立地变量统计（样地数为553个）
Table 1 Descriptive statistics of stand and site variables for pure Chinese fir plantations (n = 553)
变量
Variable平均值 Mean 标准差 SD 最小值 Min. 最大值 Max. 林分年龄/a
Stand age/year19 10 4 46 林分密度/（株·hm− 2）
Stand density/(tree·ha− 1)1 801 1 047 104 6 791 平方平均胸径
Quadratic mean DBH/cm12.1 3.8 5.7 25.0 海拔
Elevation/m483 222 43 1350 土壤厚度
Soil depth/cm93.8 18.8 9.0 160.0 腐殖层厚度
Humus depth/cm9.5 5.2 0 42.0 枯落物厚度
Litter depth/cm3.8 5.5 0 30.0 坡度
Slope/(°)25.9 7.1 3.0 46.0 方位角
Azimuth/(°)194.8 104.4 45.0 360.0 
下载: 导出CSV
表 2 6种拟合方法的回归系数和检验结果
Table 2 Regression coefficients of the six fitting methods
回归方法
Regression method所选数据点
Selected data斜率
Slope截距
Intercept普通最小二乘法
Ordinary least squares regression (OLS)RD ≥ 0.70 − 0.582 7.345 简化主轴回归
Reduced major axis regression (RMA)RD ≥ 0.70 − 0.600 7.490 分位数回归
Quantile regression (QR)全部
All plots− 0.420 6.193 修正最小二乘法
Corrected OLS (COLS)RD ≥ 0.70 − 0.582 7.489 确定性前沿函数
Deterministic frontier function (DFF)全部
All plots− 0.420 6.193 随机前沿函数
Stochastic frontier function (SFF)RD ≥ 0.70 − 0.620 7.795 注：RD，相对密度。Note: RD, relative density.
下载: 导出CSV
表 3 基于RD ≥ 0.70数据点拟合的OLS和RMA模型统计量和验证结果
Table 3 Fitting statistics and validation results of the OLS and RMA models fitted by RD ≥ 0.70 data
回归方法
Regression method回归模型的拟合统计量
Fitting statistics of the regression models交叉验证
Cross-validationdf AIC RMSE R2 NMSEte PRESS OLS − 99.830 (3) − 93.830 0.058 0.940 0.133 0.096 RMA − 100.250 (5) − 90.251 0.059 0.985 0.135 0.090
下载: 导出CSV
-
[1] Cubbage F, Harou P, Sills E. Policy instruments to enhance multi-functional forest management[J]. Forest Policy and Economics, 2007, 9(7): 833−851. doi: 10.1016/j.forpol.2006.03.010
[2] Meng J H, Lu Y C, Zeng J. Transformation of a degraded Pinus massoniana plantation into a mixed-species irregular forest: impacts on stand structure and growth in southern China[J]. Forests, 2014, 5(12): 3199−3221. doi: 10.3390/f5123199
[3] Kanninen M. Plantation forests: Global perspectives//Ecosystem goods and services from plantation forests[M]. Bauhus J, van der Meer P, Kanninen M, Eds. London: Earthscan Press, 2010: 1–15.
[4] 国家林业和草原局. 中国森林资源报告(2014—2018)[M]. 北京: 中国林业出版社, 2009. National Forestry and Grassland Administration (NFGA). China forest resources report (2014–2018)[M]. Beijing: China Forestry Publishing House, 2019.
[5] 徐济德. 我国第八次森林资源清查结果及分析[J]. 林业经济, 2014(3):6−8. Xu J D. The 8th forest resources inventory results and analysis in China[J]. Forestry Economics, 2014(3): 6−8.
[6] 彭舜磊, 王得祥, 赵辉, 等. 我国人工林现状与近自然经营途径探讨[J]. 西北林学院学报, 2008, 23(2):184−188. Peng S L, Wang D X, Zhao H, et al. Discussing the status quality of plantation and near nature forestry management in China[J]. Journal Northwest Forestry University, 2008, 23(2): 184−188.
[7] 陆元昌, 张守攻, 雷相东, 等. 人工林近自然化改造的理论基础和实施技术[J]. 世界林业研究, 2009, 22(1):20−27. Lu Y C, Zhang S G, Lei X D, et al. Theoretical basis and implementation techniques on close-to-nature transformation of plantations[J]. World Forestry Research, 2009, 22(1): 20−27.
[8] Drew T J, Flewelling J W. Stand density management: an alternative approach and its application to Douglas-Fir plantations[J]. Forest Science, 1979, 25(3): 518−532.
[9] Bormann B T, Gordon J C. Stand density effects in young red alder plantations: productivity, photosynthate partitioning, and nitrogen fixation[J]. Ecology, 1984, 65(2): 394−402. doi: 10.2307/1941402
[10] Castano S J, Barrio A M, Alvarez A P. Regional-scale stand density management diagrams for Pyrenean oak (Quercus pyrenaica Willd.) stands in north-west Spain[J]. Iforest, 2013, 6(2): 113−122.
[11] Hibbs D E. The self-thinning rule and red alder management[J]. Forest Ecology and Management, 1987, 18(4): 273−281. doi: 10.1016/0378-1127(87)90131-9
[12] Long J N. A practical approach to density management[J]. The Forestry Chronicle, 1985, 61(1): 23−27. doi: 10.5558/tfc61023-1
[13] Newton P. Evaluating the ecological integrity of structural stand density management models developed for boreal conifers[J]. Forests, 2015, 6(4): 992−1030.
[14] Mohler C L, Sprugel D G. Stand structure and allometry of trees during self-thinning of pure stands[J]. Journal of Ecology, 1978, 66(2): 599−614. doi: 10.2307/2259153
[15] Enquist B J, Brown J H, West G B. Allometric scaling of plant energetics and population density[J]. Nature, 1998, 395: 163−165. doi: 10.1038/25977
[16] Xue L, Ogawa K, Hagihara, et al. Self-thinning exponents based on the allometric model in Chinese pine (Pinus tabulaeformis Carr.) and Prince Rupprecht’s larch (Larix principis-rupprechtii Mayr) stands[J]. Forest Ecology and Management, 1999, 117(1): 87−93.
[17] Pretzsch H. A unified law of spatial allometry for woody and herbaceous plants[J]. Plant Biology, 2002, 4(2): 159−166. doi: 10.1055/s-2002-25732
[18] Inoue A, Miyake M, Nishizono T. Allometric model of the Reineke equation for Japanese cypress (Chamaecyparis obtusa) and red pine (Pinus densiflora) stands[J]. Journal of Forest Research, 2004, 9(4): 319−324. doi: 10.1007/s10310-004-0084-0
[19] Reineke L H. Perfecting a stand-density index for even-aged forests[J]. Journal of Agricultural Research, 1933, 46(7): 627−638.
[20] Yoda K, Kira T, Ogawa H, et al. Self-thinning in overcrowded pure stands under cultivated and natural conditions[J]. Journal of Biology, 1963, 14: 107−129.
[21] Westoby M. The self-thinning rule[J]. Advances in Ecological Research, 1984, 14(2): 167−225.
[22] Zhang L, Bi H, Gove J H, et al. A comparison of alternative methods for estimating the self-thinning boundary line[J]. Canadian Journal of Forest Research, 2005, 35(6): 1507−1514. doi: 10.1139/x05-070
[23] Vospernik S, Sterba H. Do competition-density rule and self-thinning rule agree?[J]. Annals of Forest Science, 2005, 72(3): 379−390.
[24] White J, Happer J L. Correlated changes in plant size and number in plant populations[J]. Journal of Ecology, 1970, 58(2): 467−485. doi: 10.2307/2258284
[25] Gorham E. Shoot height, weight and standing crop in relation to density of monospecific plant stands[J]. Nature, 1979, 279: 148−150. doi: 10.1038/279148a0
[26] White J. Demographic factors in populations of plants//Demography and evolution in plant populations[M]. Solbrig O T, Ed. Oxford: Blackwell Publishing, 1980: 21–48.
[27] Jack S B, Long J N. Linkages between silviculture and ecology: an analysis of density management diagrams[J]. Forest Ecology and Management, 1996, 86(1–3): 205−220.
[28] Luis J F S, Fonseca T F. The allometric model in the stand density management of Pinus pinaster Ait. in Portugal[J]. Annals of Forest Science, 2004, 61(8): 807−814. doi: 10.1051/forest:2004077
[29] Lonsdale W M, Watkinson A R. Tiller dynamics and self-thinning in grassland habitats[J]. Oecologia, 1983, 60(3): 390−395. doi: 10.1007/BF00376857
[30] Dunhan J B, Vinyard G L. Relationships between body mass, population density, and the self-thinning rule in stream-living salmonids[J]. Canadian Journal of Fisheries and Aquatic Sciences, 1997, 54(5): 1025−1030. doi: 10.1139/f97-012
[31] Pretzsch H. Stand density and growth of Norway spruce (Picea abies (L.) Karst.) and European beech (Fagus sylvatica L.): evidence from long-term experimental plots[J]. European Journal of Forest Research, 2005, 124(3): 193−205. doi: 10.1007/s10342-005-0068-4
[32] Solomon D S, Zhang L. Maximum size–density relationships for mixed softwoods in the northeastern USA[J]. Forest Ecology and Management, 2002, 155(1–3): 163−170.
[33] Zeide B. Tolerance and self-tolerance of trees[J]. Forest Ecology and Management, 1985, 13(3–4): 149−166.
[34] Weller D E. Will the real self-thinning rule please stand up? A reply to Osawa and Sugita[J]. Ecology, 1990, 71: 1204−1207. doi: 10.2307/1937389
[35] Puettmann K J, Hann D W, Hibbs D E. Evaluation of the size-density relationships for pure red alder and Douglas-fir stands[J]. Forest Science, 1993, 39(1): 7−27.
[36] Morris E C. Self-thinning lines differ with fertility level[J]. Ecological Research, 2002, 17(1): 17−28. doi: 10.1046/j.1440-1703.2002.00459.x
[37] Pretzsch H. Species-specific allometric scaling under self-thinning: evidence from long-term plots in forest stands[J]. Oecologia, 2006, 146(6): 572−583.
[38] Ge F, Zeng W, Ma W, et al. Does the slope of the self-thinning line remain a constant value across different site qualities? An implication for plantation density management[J/OL]. Forests, 2017, 8(10): f8100355 [2019−09−11]. http://www.doc88.com/p-1476329079105.html.
[39] West G B, Brown J H, Enquist B J. A general model for the origin of allometric scaling laws in biology[J]. Science, 1997, 276: 122−126. doi: 10.1126/science.276.5309.122
[40] Enquist B J, West G B, Charnov E L, et al. Allometric scaling of production and life history variation in vascular plants[J]. Nature, 1997, 65(9): 3529−3538.
[41] West G B, Brown J H, Enquist B J. A general model for the structure and allometry of plant vascular systems[J]. Nature, 1999, 400: 664−667. doi: 10.1038/23251
[42] Brown J H, West G B, Enquist B J. Yes, West, Brown and Enquist’s model of allometric scaling is both mathematically correct and biologically relevant[J]. Functional Ecology, 2005, 19(4): 735−738. doi: 10.1111/j.1365-2435.2005.01022.x
[43] Deng J M, Wang G X, Morris E C, et al. Plant mass-density relationship along a moisture gradient in north-west China[J]. Journal of Ecology, 2006, 94(5): 953−958. doi: 10.1111/j.1365-2745.2006.01141.x
[44] Franco M, Kelly C K. The interspecific mass–density relationship and plant geometry[J]. Proceedings of the National Academy of Sciences, 1998, 95(13): 7830−7835. doi: 10.1073/pnas.95.13.7830
[45] Belgrano A, Allen A P, Enquist B J, et al. Allometric scaling of maximum population density: a common rule for marine phytoplankton and terrestrial plants[J]. Ecology Letters, 2002, 5(5): 611−613. doi: 10.1046/j.1461-0248.2002.00364.x
[46] Zeide B. Analysis of the 3/2 power law of self-thinning[J]. Forest Science, 1987, 33(2): 517−537.
[47] Cao W V, Dean T J, Vcjr B. Modeling the size-density relationship in direct seeded slash pine stands[J]. Forest Science, 2000, 46(3): 317−321.
[48] Lonsdale W. The self-thinning rule: dead or alive?[J]. Ecology, 1990, 71(4): 1373−1388. doi: 10.2307/1938275
[49] Hamilton N S, Matthew C, Lemaire G. In defence of the −3/2 boundary rule: a reevaluation of self-thinning concepts and status[J]. Annals of Botany, 1995, 76(6): 569−577. doi: 10.1006/anbo.1995.1134
[50] Weller D E. A reevaluation of the −3/2 power rule of plant self-thinning[J]. Ecological monographs, 1987, 57(1): 23−43. doi: 10.2307/1942637
[51] Weller D E. The interspecific size–density relationship among crowded plant stands and its implications for the −3/2 power rule of self-thinning[J]. American Naturalist, 1989, 133(1): 20−41. doi: 10.1086/284899
[52] Bi H, Turvey N D. A method of selecting data points for fitting the maximum biomass-density line for stands undergoing self-thinning[J]. Australian Journal of Ecology, 1997, 22(3): 356−359. doi: 10.1111/j.1442-9993.1997.tb00683.x
[53] 付立华, 张建国, 段爱国, 等. 最大密度法则研究进展[J]. 植物生态学报, 2008, 32(2):501−511. doi: 10.3773/j.issn.1005-264x.2008.02.030 Fu L H, Zhang J G, Duan A G, et al. Review of studies on maximum size–density rules[J]. Journal of Plant Ecology, 2008, 32(2): 501−511. doi: 10.3773/j.issn.1005-264x.2008.02.030
[54] Osawa A, Allen R B. Allometric theory explains self-thinning relationships of mountain beech and red pine[J]. Ecology, 1993, 74(4): 1020−1032. doi: 10.2307/1940472
[55] Osawa A. Inverse relationship of crown fractal dimension to self-thinning exponent of tree populations: a hypothesis[J]. Canadian Journal of Forest Research, 1995, 25(10): 1608−1617. doi: 10.1139/x95-175
[56] Osawa A, Sugita S. The self-thinning rule: another interpretation of Weller’s results[J]. Ecology, 1989, 70(1): 279−283. doi: 10.2307/1938435
[57] Wilson D S, Seymour R S, Maguire D A. Density management diagram for northeastern red spruce and balsam fir forests[J]. Northern Journal of Applied Forestry, 1999, 16(1): 48−56. doi: 10.1093/njaf/16.1.48
[58] Newton P F. Forest production model for upland black spruce stands: optimal site occupancy levels for maximizing net production[J]. Ecological Modelling, 2006, 190: 190−204. doi: 10.1016/j.ecolmodel.2005.02.022
[59] Blackburn T M, Lawton J H, Perry J N. A method of estimating the slope of upper bounds of plots of body size and abundance in natural animal assemblages[J]. Oikos, 1992, 65(1): 107−112. doi: 10.2307/3544892
[60] Scharf F S, Juanes F, Sutherlang M. Inferring ecological relationships from the edges of scatter diagrams: comparison of regression techniques[J]. Ecology, 1998, 79(2): 448−460. doi: 10.1890/0012-9658(1998)079[0448:IERFTE]2.0.CO;2
[61] 孙洪刚, 张建国, 段爱国. 数据点选择与参数估计方法对杉木人工林自疏边界线的影响[J]. 植物生态学报, 2010, 34(4):56−63. Sun H G, Zhang J G, Duan A G. A comparison of selecting data points and fitting coefficients methods for estimating self-thinning boundary line[J]. Chinese Journal of Plant Ecology, 2010, 34(4): 56−63.
[62] Weisberg S. Applied linear regression (4th Edition)[M]. Hoboken: John Wiley & Sons Press, 2014.
[63] Niklas K J. Plant allometry: the scaling of form and process[M]. Chicago: University of Chicago Press, 1994.
[64] Leduc D J. A comparative analysis of the reduced major axis technique of fitting lines to bivariate data[J]. Canadian Journal of Forest Research, 1987, 17(7): 654−659. doi: 10.1139/x87-107
[65] Bohonak A J. RMA: software for reduced major axis regression, Version 1.17[Z/OL]. San Diego: San Diego State University, 2004 [2019−10−12]. http://www.bio.sdsu.edu/pub/andy/rma.html.
[66] Weller D E. Self-thinning exponent correlated with allometric measures of plant geometry[J]. Ecology, 1987, 68(4): 813−821. doi: 10.2307/1938352
[67] Begin E, Begin J, Belanger L, et al. Balsam fir self-thinning relationship and its constancy among different ecological regions[J]. Canadian Journal of Forest Research, 2001, 31(6): 950−959. doi: 10.1139/x01-026
[68] Li H T, Han X G, Wu J G. Variant scaling relationship for mass-density across tree-dominated communities[J]. Journal of Integrative Plant Biology, 2006, 48(3): 268−277. doi: 10.1111/j.1744-7909.2006.00222.x
[69] Koenker R, Bassett G. Regression quantiles[J]. Econometrica, 1978, 46(1): 33−50. doi: 10.2307/1913643
[70] Cade B S, Noon B R. A gentle introduction to quantile regression for ecologists[J]. Frontiers in Ecology and the Environment, 2003, 1(8): 412−420. doi: 10.1890/1540-9295(2003)001[0412:AGITQR]2.0.CO;2
[71] Greene W H. The econometric approach to efficiency analysis// Fried H O, Lovell C A K, Schmidt S S. The measurement of productive efficiency: techniques and applications[M]. New York: Oxford University Press, 1993: 68–119.
[72] Kopp R J, Mullahy J. Least squares estimation of econometric frontier models: consistent estimation and inference[J]. The Scandinavian Journal of Economics, 1993, 95(1): 125−132. doi: 10.2307/3440140
[73] Aigner D J, Chu S F. On estimating the industry production function[J]. American Economic Review, 1968, 58(4): 826−839.
[74] Greene W H. Maximum likelihood estimation of econometric frontier functions[J]. Journal of Econometrics, 1980, 13(1): 27−56. doi: 10.1016/0304-4076(80)90041-X
[75] Aigner D, Lovell C A K, Schmidt P. Formulation and estimation of stochastic frontier production function models[J]. European Journal of Clinical Pharmacology, 1977, 6(1): 21−37.
[76] Stevenson R. Likelihood functions for generalized stochastic frontier estimation[J]. Journal of Econometrics, 1980, 13(1): 58−66.
[77] Bi H, Wan G, Turvey N D. Estimating the self-thinning boundaey line as a density-dependent stochastic biomass frontier[J]. Ecology, 2000, 81(6): 1477−1483. doi: 10.1890/0012-9658(2000)081[1477:ETSTBL]2.0.CO;2
[78] Ogawa K. Relationships between mean shoot and root masses and density in an overcrowded population of hinoki (Chamaecyparis obtusa (Sieb. et Zucc.) Endl.) seedlings[J]. Forest Ecology and Management, 2005, 213(1): 391−398.
[79] Ogawa K. Time-trajectory of mean phytomass and density during a course of self-thinning in a sugi (Cryptomeria japonica D. Don) plantation[J]. Forest Ecology and Management, 2005, 214(1): 104−110.
[80] Del río M, Montero G, Bravo F. Analysis of diameter-density relationships and self-thinning in non-thinned even-aged Scots pine stands[J]. Forest Ecology and Management, 2001, 142(1): 79−87.
[81] Zeide B. How to measure stand density?[J]. Trees, 2005, 19(1): 1−14. doi: 10.1007/s00468-004-0343-x
[82] R Core Team. R: a language and environment for statistical computing[Z/OL]. 2015 [2018–11–19]. https://www.r–project.org/.
[83] Legendre P. lmodel2: Model II regression//R package, version 1.7–2[Z/OL]. 2014 [2018–12–05]. http://CRAN.R–project.org/package=lmodel2.
[84] Koenker R. Quantreg: quantile regression and related methods [Z/OL]. 2013 [2018–11–21]. http://cran.r–project.org/web/packages/quantreg/quantreg.
[85] Lindo Systems Inc. LINGO user’s guide[M]. Chicago: Lindo Systems Inc., 2013.
[86] Econometric Software Inc. LIMDEP 7.0 user’s manual (computer Manual)[M]. New York: Econometric Software Inc., 1998.
[87] Drew T J, Fleweling J W. Some recent japanese theories of yield–density relationships and their application to monterey pine plantations[J]. Forest Science, 1977, 23(4): 517−534.
[88] Bi H. The self-thinning surface[J]. Forest Science, 2001, 47(3): 361−370.
[89] Guo Q, Rundel P W. Self-thinning in early postfire chaparral succession: mechanisms, implications, and a combined approach[J]. Ecology, 1998, 79(2): 579−586. doi: 10.1890/0012-9658(1998)079[0579:STIEPC]2.0.CO;2
[90] Bi H. Stochastic frontier analysis of a classic self-thinning experiment[J]. Austral Ecology, 2004, 29(4): 408−417. doi: 10.1111/j.1442-9993.2004.01379.x
-
期刊类型引用(2)
1. 朱莉，王猛，孟兆新，李博，乔际冰. 基于强化学习的木工送料平台误差控制研究. 林产工业. 2023(11): 38-45 . 
百度学术
2. 孟兆新，郭骐瑞，邢鑫，殷鑫，宋绪秋. 基于数字孪生的并联式曲线送料平台误差分析. 林业机械与木工设备. 2022(02): 28-33 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 7326
- HTML全文浏览量: 890
- PDF下载量: 118
- 被引次数: 3
