Modeling variable exponential taper function for Cunninghamia lanceolata based on quantile regression
-
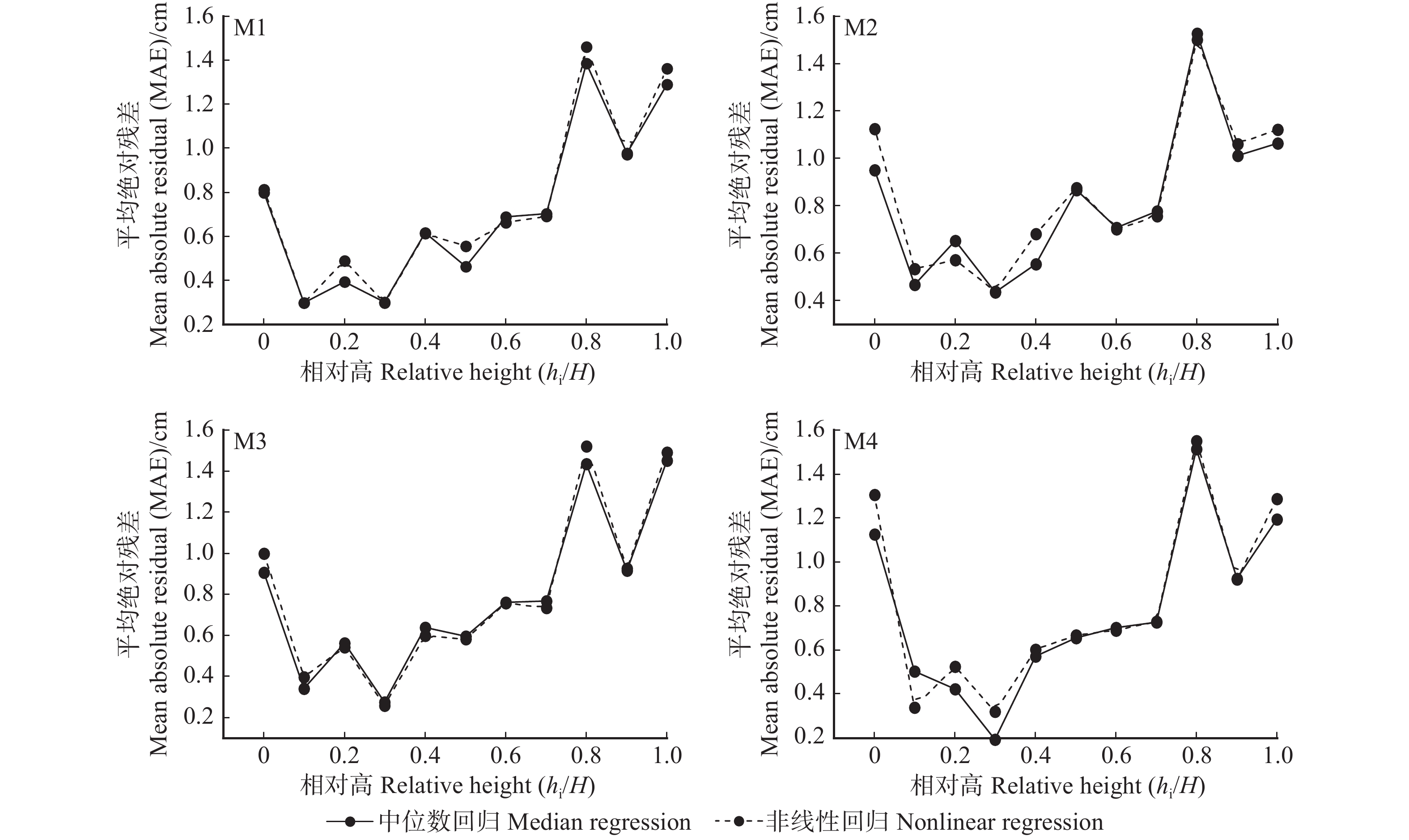
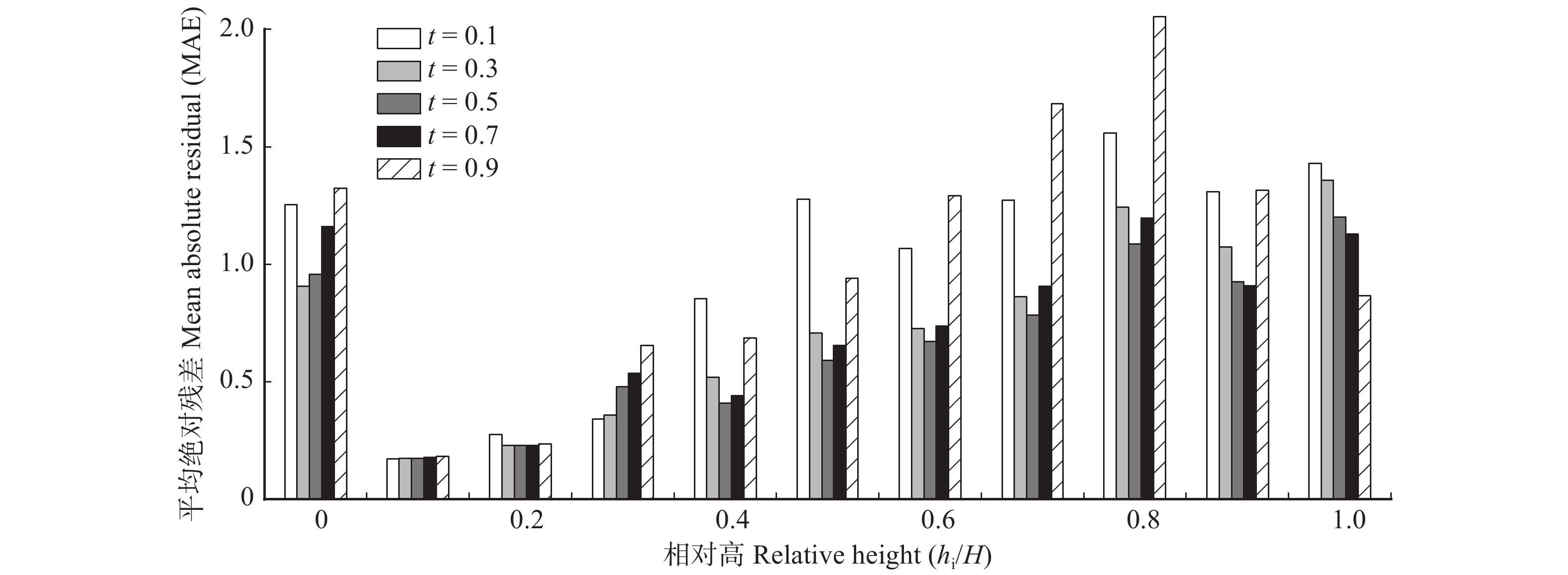
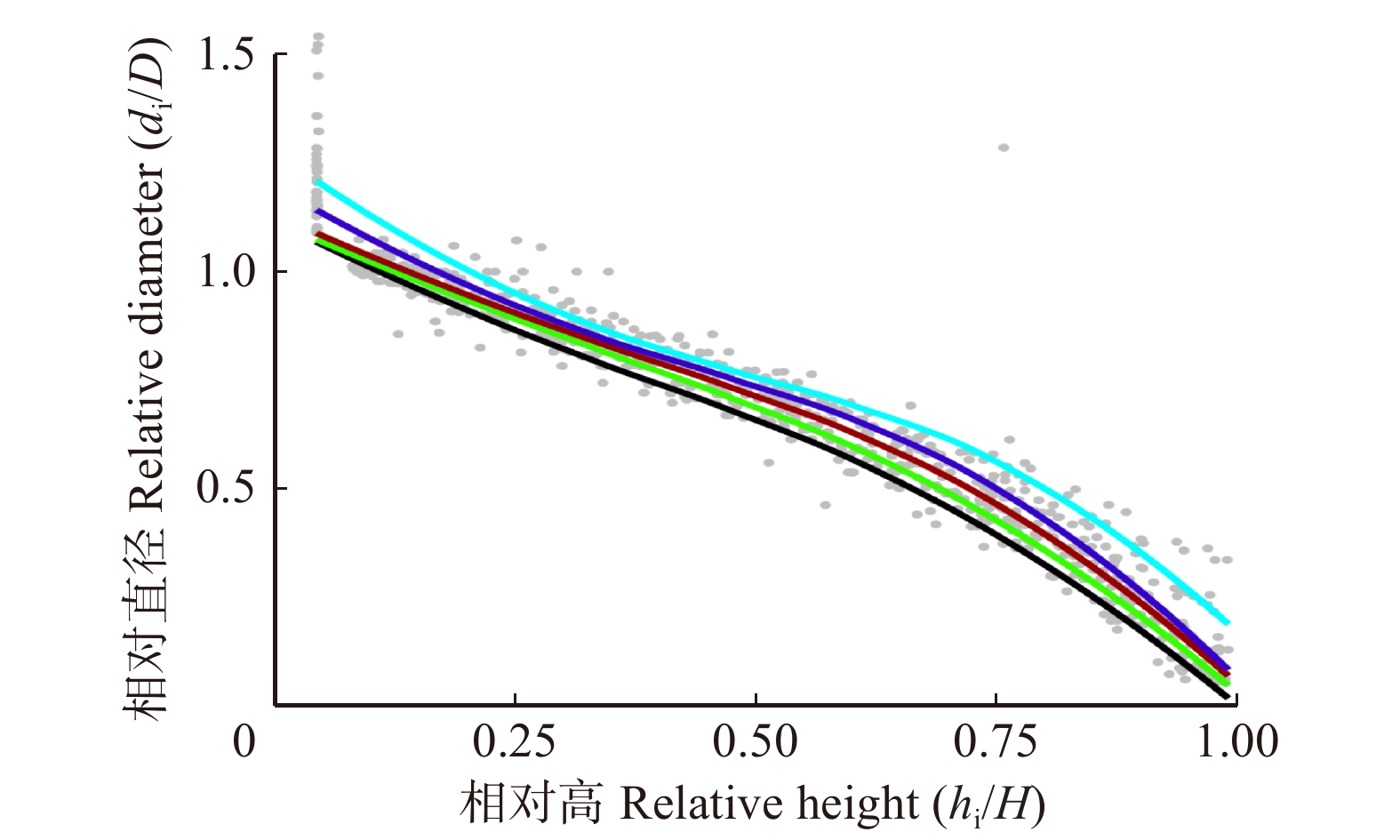
摘要:目的 采用非线性分位数回归法构建不同分位点的杉木可变指数削度方程，与非线性模型进行比较，以提高杉木干形的预测精度。方法 利用福建省将乐国有林场的73 株（793组）杉木解析木数据，选取4个可变指数削度方程，基于5折交叉验证，分别采用非线性分位数回归与非线性回归构建削度方程。选用调整后决定系数（R2）、均方根误差（RMSE）、平均误差（ME）、相对误差（RE）和平均绝对误差（MAE）5个模型评价指标，结合图形对各模型的拟合结果和预测结果进行评价。结果 （1）4个可变指数削度方程在5个分位点（t = 0.1, 0.3, 0.5, 0.7, 0.9）处均能收敛，说明分位数回归可以建立不同分位点的估测模型，能更全面地描述杉木干形的变化。（2）4个削度方程在分位点为0.5处的精度最高，R2均在0.97左右。对于削度方程M1和M3，基于中位数回归（t = 0.5）的拟合精度与预测精度均高于非线性回归，且M1的预测值更加集中。（3）在不同分位点下，各模型对树干不同位置的预测精度不同，分位值为0.9和0.3的模型分别对梢头部分和树干基部的预测精度最高。结论 基于分位数回归的可变指数削度方程不仅能精确预测平均条件下杉木的树干直径，而且能预测任意分位条件下杉木干形的变化趋势。不同分位点模型对树干不同位置的预测精度不同，基于M1削度方程，建立多分位点回归模型能进一步提高研究区杉木干形的预测精度。Abstract:Objective In order to improve prediction accuracy of Chinese fir stem profile, we used nonlinear quantile regression to establish variable exponential taper equations at different quantile points, and compared their fitting and prediction accuracy with nonlinear regression model.Method This study took 73 Chinese fir (Cunninghamia lanceolata) stem data from the Jiangle Forest Farm in Fujian Province of eastern China. Then we selected 4 variable exponential taper equations, and based on 5-fold cross-validation, used nonlinear quantile regression and nonlinear regression to establish taper equations, respectively. Five model evaluation indicators were selected, including the adjusted coefficient of determination (R2), root mean square error (RMSE), average error (ME), relative error (RE) and average absolute error (MAE), combined with graphs to evaluate the fitting and prediction results.Result The research results showed: (1) the 4 variable exponential taper equations converged at all quantile points (t = 0.1, 0.3, 0.5, 0.7, 0.9), indicating that quantile regression can develop different models at different quantiles. So this method can describe the change of Chinese fir stem shape more comprehensively. (2) The accuracy of four taper equations at the quantile point of 0.5 was all higher than others, with R2 about 0.97. For taper equations M1 and M3, the fitting and prediction accuracy based on the median regression (t = 0.5) were both higher than those of nonlinear regression. And the prediction values of the M1 equation were more concentrated. (3) At different quantile points, models had different prediction accuracies for varied stem positions. Models with quantile values of 0.9 and 0.3 had the highest prediction accuracy for the stem top part and the base part, respectively.Conclusion The variable exponential taper equations developed by quantile regression can not only accurately predict stem diameters under average condition, but also predict the changing trend of stem shape under arbitrary quantile conditions. Quantile models have different prediction accuracies for varied stem positions. The multi-quantile regression model of M1 can further improve the prediction accuracy of the Chinese fir stem profile.
-
-
![]()
图 1 不同分位点模型对杉木干形拟合图
Figure 1. Cunninghamia lanceolata stem curve simulation of quantile regression
![]()
图 2 基于非线性回归与中位数回归的各削度方程对不同树高处截面直径的预测结果
Figure 2. Prediction results of taper function at different heights of stem based on nonlinear regression and median regression
![]()
图 3 中位数回归的杉木干形预测图
散点为观测值，线条为中位数回归模型的预测曲线。Points are the observed values and the lines are predicting curves of the median regression models.
Figure 3. Stem profile prediction for Chinese fir based on median regression
![]()
图 4 基于不同分位点对树干不同位置的预测结果
Figure 4. Prediction results of different stem positions based on varied quantiles
表 1 杉木调查因子统计表
Table 1 Basic statistic information of sample trees
项目 Item 最小值 Min. 最大值 Max. 平均值 Mean 中位数 Median 标准差 SD 变异系数 CV 树干直径 Stem diameter (di)/cm 0.96 54.00 11.81 11.43 5.987 4.490 树干高 Height along stem (hi)/m 0.0 25.0 8.0 7.0 5.980 3.141 胸径 DBH (D)/cm 4.90 28.40 17.23 17.12 5.003 1.365 树高 Tree height (H)/m 4.1 25.5 17.3 18.2 5.980 1.236 
下载: 导出CSV
表 3 基于非线性回归与分位数回归的各削度方程的拟合结果
Table 3 Fit-goodness statistics of taper functions based on nonlinear regression and quantile regression
模型 Model 建模方法 Modeling method 分位点 Quantile 模型的评价指标 Evaluation index of the model R2 MAE/cm RMSE/cm RE ME/cm M1 非线性回归 Nonlinear regression 0.972 0.635 0.990 3 379.627 0.018 分位数回归 Quantile regression 0.1 0.940 0.953 1.427 5 076.793 0.843 0.3 0.960 0.699 1.122 4 391.152 0.192 0.5 0.975 0.627 0.801 3 337.980 −0.017 0.7 0.970 0.702 1.067 3 738.042 −0.310 0.9 0.930 1.089 1.542 5 799.579 −0.922 0.960 0.830 1.172 4 421.905 0.016 M2 非线性回归 Nonlinear regression 0.971 0.704 1.022 3 749.541 0.017 分位数回归 Quantile regression 0.1 0.920 1.329 1.742 7 076.160 1.263 0.3 0.950 0.932 1.303 4 961.605 0.629 0.5 0.968 0.825 1.180 4 395.446 −0.012 0.7 0.950 0.940 1.320 5 003.610 −0.423 0.9 0.900 1.473 1.877 7 844.500 −1.292 M3 非线性回归 Nonlinear regression 0.972 0.731 1.090 3 892.878 0.032 分位数回归 Quantile regression 0.1 0.940 1.062 1.484 5 656.519 0.999 0.3 0.960 0.764 1.179 4 070.373 0.525 0.5 0.974 0.626 1.008 3 397.303 −0.013 0.7 0.970 0.785 1.102 4 182.318 −0.350 0.9 0.920 0.309 1.685 6 969.442 −1.180 M4 非线性回归 Nonlinear regression 0.975 0.734 1.022 3 949.541 0.170 分位数回归 Quantile regression 0.1 0.940 0.953 1.472 5 076.793 0.843 0.3 0.960 0.726 1.137 3 867.362 0.345 0.5 0.979 0.710 1.003 3 781.487 −0.017 0.7 0.960 0.800 1.165 4 259.591 −0.267 0.9 0.920 1.141 1.664 7 513.007 −1.184
下载: 导出CSV
表 2 非线性回归与分位数回归对各削度方程的参数估计结果
Table 2 Parameter estimates of taper function based on nonlinear regression and quantile regression
模型 Model 建模方法 Modeling method 分位点 Quantile 模型参数 Parameters of model b1 b2 b3 b4 b5 M1 非线性回归 Nonlinear regression 0.783 3 −3.151 0 1.841 7 1.158 8 分位数回归 Quantile regression 0.1 −0.181 2 −0.500 4 0.231 5 1.194 4 0.3 0.623 3 −2.716 6 1.659 8 1.155 7 0.5 0.788 4 −3.388 2 2.068 0 1.191 0 0.7 1.533 0 −5.229 0 3.185 1 1.143 0 0.9 1.947 9 −6.171 4 3.604 2 1.149 8 M2 非线性回归 Nonlinear regression 2.899 0 6.165 0 分位数回归 Quantile regression 0.1 3.024 9 7.133 1 0.3 2.868 6 6.263 2 0.5 2.817 7 5.880 6 0.7 2.769 8 5.519 8 0.9 2.901 9 5.677 5 M3 非线性回归 Nonlinear regression 1.446 6 0.914 6 1.182 4 −1.770 1 1.306 2 分位数回归 Quantile regression 0.1 1.353 6 0.918 7 1.109 7 −1.422 7 1.188 5 0.3 1.312 0 0.929 5 0.766 2 −0.963 6 0.974 8 0.5 1.366 9 0.920 3 0.918 1 −1.223 2 1.029 3 0.7 1.440 5 0.921 0 1.359 8 −1.960 8 1.317 8 0.9 1.516 5 0.925 0 1.179 5 −2.099 3 1.482 4 M4 非线性回归 Nonlinear regression 1.390 6 0.636 9 −0.727 4 分位数回归 Quantile regression 0.1 1.331 0 0.754 1 −0.836 5 0.3 1.294 7 0.677 3 −0.749 8 0.5 1.293 5 0.629 2 −0.700 0 0.7 1.323 9 0.574 5 −0.651 2 0.9 1.598 4 0.565 1 −0.684 9 注：M1、M2、M3和M4分别代表文中的公式(1)、公式(2)、公式(3)和公式(4)。下同。Notes: M1, M2, M3 and M4 represent formula (1), formula (2), formula (3) and formula (4), respectively. The same below.
下载: 导出CSV
表 4 非线性回归模型与中位数回归模型的预测精度检验
Table 4 Prediction statistics of nonlinear regression models and median regression models
模型
Model非线性回归模型预测精度检验
Prediction statistics of non-linear regression中位数回归模型预测精度检验
Prediction statistics of median regressionR2 MAE/cm RMSE/cm RE ME/cm R2 MAE/cm RMSE/cm RE ME/cm M1 0.972 0.638 0.994 850.911 0.017 0.975 0.158 0.801 797.121 0.007 M2 0.961 0.832 1.180 1109.960 0.088 0.966 0.207 1.155 960.196 0.612 M3 0.970 0.177 1.028 945.102 0.015 0.979 0.174 1.031 926.861 0.011 M4 0.966 0.201 1.095 978.018 0.031 0.966 0.178 1.100 950.730 0.017
下载: 导出CSV
-
[1] 曾伟生, 廖志云. 削度方程的研究[J]. 林业科学, 1997, 33(2):32−37. Zeng W S, Liao Z Y. A study on taper equation[J]. Scientia Silvae Sinicae, 1997, 33(2): 32−37.
[2] 梁子瑜, 孙圆, 梁欣廉, 等. 基于地面激光扫描仪的树干削度方程提取[J]. 南京林业大学学报(自然科学版), 2014, 38(5):6−10. Liang Z Y, Sun Y, Liang X L, et al. The extraction of stem taper equation based on terrestrial laser scanning[J]. Journal of Nanjing Forestry University (Natural Science), 2014, 38(5): 6−10.
[3] 胥辉, 孟宪宇. 天山云杉削度方程与材种出材率表的研究[J]. 北京林业大学学报, 1996, 18(3): 21−30. Xu H, Meng X Y. Study on taper function and merchantable volumn yielding rate table of Picea sehrenkiana var. tianshaca[J]. Journal of Beijing Forestry University, 1996, 18(3): 21−30.
[4] 庞丽峰, 贾宏炎, 陆元昌, 等. 分段削度方程2种估计方法比较[J]. 林业科学, 2015, 51(12):141−148. Pang L F, Jia H Y, Lu Y C, et al. Comparison of two parameters estimation methods for segmented taper equations[J]. Scientia Silvae Sinicae, 2015, 51(12): 141−148.
[5] Lanssanova L R, Machado S D A, Garrett A T D A, et al. Mixed-effect non-linear modelling for diameter estimation along the stem of Tectona grandis in mid-western Brazil[J]. Southern Forests: a Journal of Forest Science, 2019, 81(2): 167−173. doi: 10.2989/20702620.2018.1531279
[6] Pukkala T, Hanssen K H, Andreassen K. Stem taper and bark functions for Norway spruce in Norway[J/OL]. Silva Fennica, 2019, 53(3): 10187 [2020−03−15]. https://doi.org/10.14214/sf.10187.
[7] 韩飞. 落叶松人工林削度方程的研究[D]. 哈尔滨: 东北林业大学, 2010. Han F. The study of taper equation for Larix olgensis plantation[D]. Harbin: Northeast Forestry University, 2010.
[8] Fonweban J, Gardiner B, Macdonald E, et al. Taper functions for Scots pine (Pinus sylvestris L.) and Sitka spruce (Picea sitchensis (Bong.) Carr.) in northern Britain[J]. Forestry, 2011, 84(1): 49−60. doi: 10.1093/forestry/cpq043
[9] Rojo A, Perales X, Sánchez-Rodríguez F, et al. Stem taper functions for maritime pine (Pinus pinaster Ait.) in Galicia (Northwestern Spain)[J]. European Journal of Forest Research, 2005, 124(3): 177−186. doi: 10.1007/s10342-005-0066-6
[10] 马岩岩, 姜立春. 基于非线性分位数回归的落叶松树干削度方程[J]. 林业科学, 2019, 55(10):68−75. Ma Y Y, Jiang L C. Stem taper function for Larix gmelinii based on nonlinear quantile regression[J]. Scientia Silvae Sinicae, 2019, 55(10): 68−75.
[11] Zang H, Lei X, Zeng W. Height-diameter equations for larch plantations in northern and northeastern China: a comparison of the mixed-effects, quantile regression and generalized additive models[J]. Forestry, 2016, 89(4): 434−445. doi: 10.1093/forestry/cpw022
[12] Cao Q V, Wang J. Evaluation of methods for calibrating a tree taper equation[J]. Forest Science, 2015, 61(2): 213−219. doi: 10.5849/forsci.14-008
[13] Zhang B, Sajjad S, Chen K, et al. Predicting tree height-diameter relationship from relative competition levels using quantile regression models for Chinese fir (Cunninghamia lanceolata) in Fujian Province, China[J]. Forests, 2020, 11(2): 183−185. doi: 10.3390/f11020183
[14] 谢晶晶. 非参数分位数回归模型估计方法的比较研究[D]. 厦门: 厦门大学, 2019. Xie J J. A comparative of nonparametric conditional quantile estimation[D]. Xiamen: Xiamen University, 2019.
[15] 高慧淋, 董利虎, 李凤日. 基于分位数回归的长白落叶松人工林最大密度线[J]. 应用生态学报, 2016, 27(11):3420−3426. Gao H L, Dong L H, Li F R. Maximum density-size line for Larix olgensis based on quantile regression[J]. Chinese Journal of Applied Ecology, 2016, 27(11): 3420−3426.
[16] 赵梦草, 顾海燕. 用分位数回归建立的混交林树高生长模型[J]. 东北林业大学学报, 2020, 48(2):24−28. Zhao M C, Gu H Y. Growth model of mixed forest tree height based on quantile regression[J]. Journal of Northeast Forestry University, 2020, 48(2): 24−28.
[17] 张冬燕, 王冬至, 李晓, 等. 基于分位数回归的针阔混交林树高与胸径的关系[J]. 浙江农林大学学报, 2020, 37(3):424−431. Zhang D Y, Wang D Z, Li X, et al. Relationship between height and diameter at breast height (DBH) in minxed coniferous and broadleaved forest based on quantile regression[J]. Journal of Zhejiang A&F University, 2020, 37(3): 424−431.
[18] 付立华, 侯金潮, 孙赫, 等. 基于分位数回归的华北落叶松干形曲线模拟[J]. 林业资源管理, 2020(1):151−157. Fu L H, Hou J C, Sun H, et al. Stem shape simulation for Larix principis-rupprechtii using quantile regression[J]. Forestry Resources Management, 2020(1): 151−157.
[19] 张森森. 基于混合效应模型的可变密度可变指数削度方程构建研究[D]. 北京: 中国林业科学研究院, 2017. Zhang S S. Nonlinear mixed-effects modeling of variable exponent taper equations with density for Chinese fir[D]. Beijing: Chinese Academy of Forestry, 2017.
[20] 梅光义, 孙玉军. 国内外削度方程研究进展[J]. 世界林业研究, 2015, 28(4):44−49. Mei G Y, Sun Y J. Research progress in stem taper equation[J]. World Forestry Research, 2015, 28(4): 44−49.
[21] 梅光义. 杉木人工林生长模型与多功能经营模拟研究[D]. 北京: 北京林业大学, 2017. Mei G Y. Study on growth model and multi-functional simulation of chinese fir plantation [D]. Beijing: Beijing Forestry University, 2017.
[22] 李婷婷. 浙江开化县林场杉木理论出材率表编制方法研究[D]. 北京: 北京林业大学, 2011. Li T T. Research on compiling method of theoretical yield rate table of Chinese fir in the forest farm of Kaihua County, Zhejiang [D]. Beijing: Beijing Forestry University, 2011.
[23] 陈萍. 福建省主要用材树种杉木削度方程的研究[J]. 华东森林经理, 2005, 19(1):58−63. Chen P. Research on the taper equation of the main timber species Chinese fir in Fujian Province[J]. East China Forestry Manager, 2005, 19(1): 58−63.
[24] 段爱国, 张建国, 童书振. 6种生长方程在杉木人工林林分直径结构上的应用[J]. 林业科学研究, 2003, 16(4):423−429. Duan A G, Zhang J G, Tong S Z. Application of six growth equations on stands diameter structure of Chinese fir plantations[J]. Forestry Science Research, 2003, 16(4): 423−429.
[25] 王明亮. 理论造材: 削度方程和出材率表的编制[J]. 林业科学研究, 1998, 11(3):271−276. Wang M L. Theoretical bucking taper equations and merchantable volume tables[J]. Forestry Science Research, 1998, 11(3): 271−276.
[26] 辛士冬, 姜立春. 利用分位数回归模拟人工樟子松树干干形[J]. 北京林业大学学报, 2020, 42(2):1−8. Xin S D, Jiang L C. Modeling stem taper profile for Pinus sylvestris plantations using nonlinear quantile regression[J]. Journal of Beijing Forestry University, 2020, 42(2): 1−8.
-
期刊类型引用(3)
1. 李辉，林沂，孟祥爽，史振伟，蔡万园. 基于地基激光雷达的栾树分形特征分析. 山东农业大学学报(自然科学版). 2022(03): 475-483 . 
百度学术
2. 何东健，熊虹婷，芦忠忠，刘建敏. 基于多视角立体视觉的拔节期玉米水分胁迫预测模型. 农业机械学报. 2020(06): 248-257 . 百度学术
3. 郭彩玲，刘刚. 基于颜色取样的苹果树枝干点云数据提取方法. 农业机械学报. 2019(10): 189-196 . 百度学术
其他类型引用(7)
计量
- 文章访问数: 1152
- HTML全文浏览量: 397
- PDF下载量: 69
- 被引次数: 10
