喀斯特常绿落叶阔叶混交林木本植物幼苗组成及其年际动态

杨廷丽; 安明态; 张广奇; 戚玉娇

doi:10.12171/j.1000-1522.20210018

喀斯特常绿落叶阔叶混交林木本植物幼苗组成及其年际动态

贵州大学林学院，贵州贵阳 550025

基金项目: 国家自然科学基金项目（32060266、31700385），贵州省科技计划项目（黔科合基础[2020]1Y124），贵州省一流学科建设项目（GNYL[2017]007）

详细信息

作者简介:
杨廷丽。主要研究方向：森林经理学。Email：yangtingligz@qq.com　地址：550025贵州省贵阳市花溪区贵州大学西校区崇德楼林学院

责任作者:
戚玉娇，副教授。主要研究方向：森林经理学和森林生态学研究。Email：yjqi@gzu.edu.cn　地址：同上

中图分类号: S718
计量
- 文章访问数: 849
- HTML全文浏览量: 221
- PDF下载量: 71
出版历程
- 收稿日期: 2021-01-19
- 修回日期: 2021-03-30
- 录用日期: 2022-03-08
- 网络出版日期: 2022-03-15
- 发布日期: 2022-04-24

Seedling composition and its interannual dynamics of woody plants in karst evergreen deciduous broadleaved mixed forest

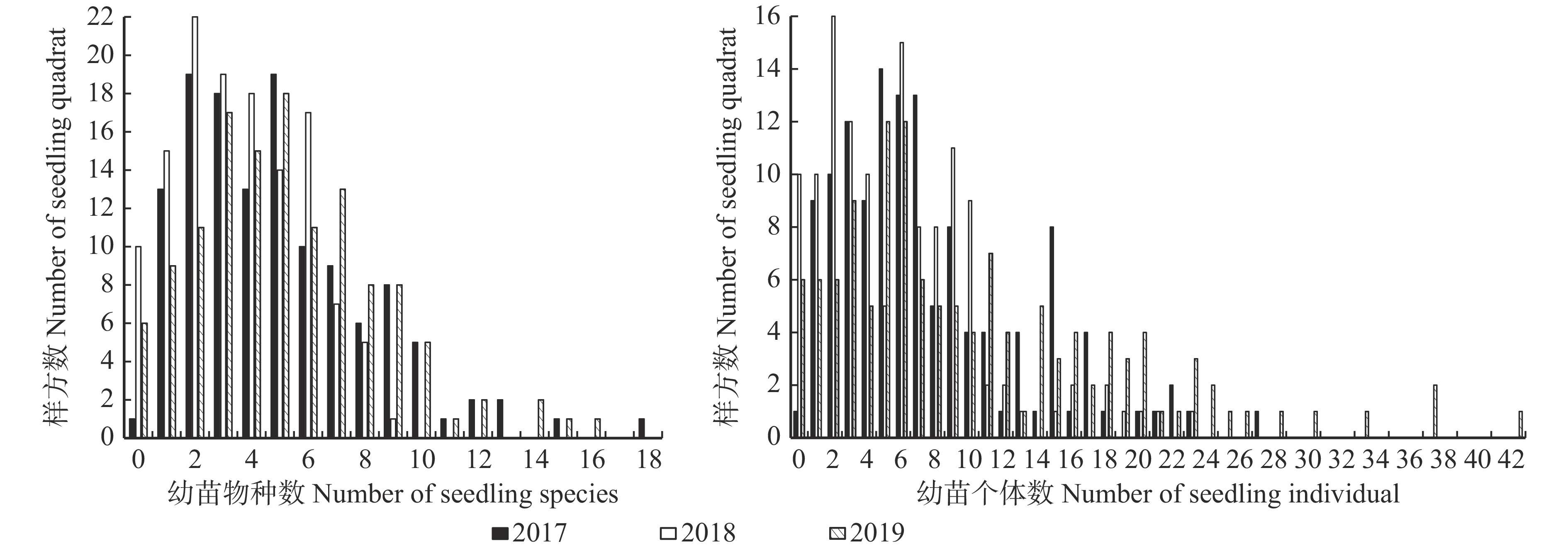
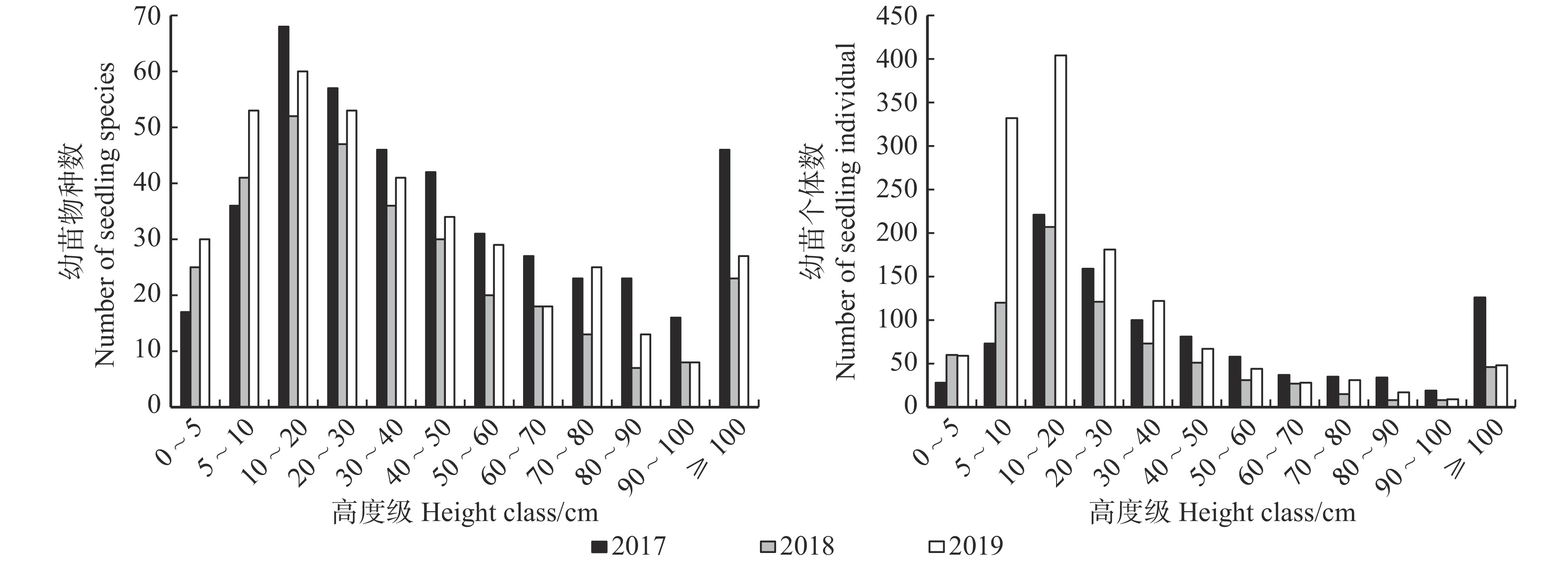
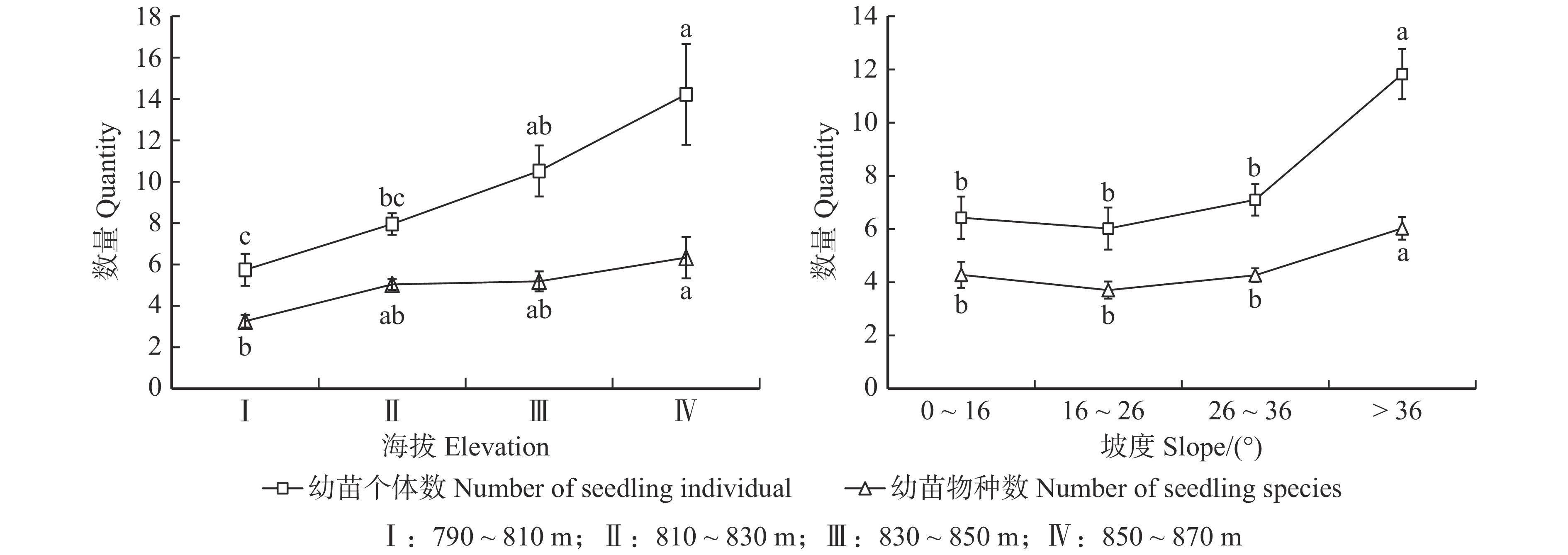
College of Forestry, Guizhou University, Guiyang 550025, Guizhou, China

摘要

摘要:
目的研究幼苗更新动态，为揭示群落更新机制和植被斑块分布格局提供理论依据。
方法依托于茂兰国家级自然保护区喀斯特常绿落叶阔叶混交林1.28 hm²固定样地，对样地内128个1 m × 1 m样方中胸径 < 1 cm的木本植物幼苗进行连续3年调查，分析了该森林群落中木本植物幼苗的种类、数量、萌发和死亡特征，探究了主要树种幼苗的年际分布格局。
结果（1）木本植物幼苗的数量和物种组成在年际之间均表现出明显的差异，2017年调查到55科96属121种971株（8 株/m²），2018年40科66属78种767株（6 株/m²）和2019年39科70属87种1 342株（10 株/m²），其中木质藤本幼苗是林下幼苗的重要组成部分。（2）林下幼苗的主要树种成分与样地内主要树种组成保持着一定的相似性，主要树种的幼苗在林下同样保持着优势地位。（3）木本植物幼苗个体数和物种数在高度级上的分布呈偏峰现象，10 ~ 20 cm高度级达到最高，之后逐渐减少，说明林下幼苗存在一定的负密度制约效应。（4）木本植物幼苗个体数和物种数都表现为随海拔、坡度的升高呈现增加的趋势，且其密度在空间上呈现复杂的斑块分布。
结论茂兰喀斯特常绿落叶阔叶混交林林下木本植物幼苗物种丰富，空间上呈现复杂的斑块分布且受海拔、坡度的显著影响，存在一定的负密度制约效应。幼苗的长期监测有助于我们更加准确地认识群落更新过程，为今后喀斯特森林的合理保护与科学管理提供依据。
- 喀斯特森林 /
- 物种组成 /
- 幼苗分布 /
- 年际动态
Abstract:
Objective The study of seedling regeneration is of great significance to reveal the mechanism of community regeneration and the distribution pattern of vegetation patches.
Method Based on the 1.28 ha fixed sample plot of karst evergreen deciduous broadleaved mixed forest in Maolan National Nature Reserve of southwestern China, the seedlings with DBH < 1 cm in 128 sample plots (1 m × 1 m) were investigated for three years. We analyzed the species, quantity, germination and death characteristics of woody plant seedlings in the forest community, and explored the annual distribution pattern of seedlings of main tree species.
Result (1) There were significant differences in the number and species composition of woody plant seedlings between different years. A total of 971 seedlings (8 plant/m²) were found in 2017, belonging to 55 families, 96 genera and 121 species; 767 seedlings (6 plant/m²) were found in 2018, belonging to 40 families, 66 genera and 78 species; and 1 342 seedlings (10 plant/m²) were found in 2019, belonging to 39 families, 70 genera and 87 species. Among them, the woody vine seedlings were important components of seedlings under the forest. (2) Main tree species composition of seedlings under forest was similar to that of main tree species in the sample plot, and also maintained the dominant position under the forest. (3) The number of individuals and species of woody plant seedlings showed a partial peak at the height classes, which reached the highest at the 10−20 cm height classes and then decreased gradually, indicating that there was a certain negative density dependence on the seedlings. (4) The number of individuals and species of woody plant seedlings increased with the increase of elevation and slope, and the density of woody plant seedlings showed a complex patch distribution.
Conclusion There are abundant species of woody plant seedlings under Maolan karst evergreen deciduous broadleaved mixed forest, showing complex patch distribution in space, and there is a certain negative density restriction effect due to the significant influence of altitude and slope. The long-term monitoring of seedlings will help us to understand the process of community renewal more accurately, and provide a basis for the rational protection and scientific management of karst forest in the future.
- karst forest /
- species composition /
- seedling distribution /
- interannual dynamics

HTML全文

树高和胸径是森林资源调查和经营效果评价的主要林分调查因子，也是林木生长发育和林分材积数量研究的重要变量。树高与胸径关系一直是林学研究的热点。通过量化特定树种的树高与胸径关系拟合树高−胸径预测模型，在计算树干材积、出材率表编制、生长和收获模型、森林资源调查、立地质量评价以及森林碳储量估算方面具有广泛应用。

分位数回归方法具有较长的统计学历史和理论基础，最早于1978年由Koenker等^[1]根据中位数回归推导提出。由于对调查数据模型方差没有严格要求，利用自变量的多个分位数求得因变量条件分布的相应分位数方程，能够描述任意发散点处变量的变化趋势。对具有尖峰、厚尾、异方差显著的数据拟合效果更加稳健^[2]，相对最小二乘回归具有更大优势。已广泛应用于林分自疏边界线、直径分布规律、林分密度指数、林木干形、树冠轮廓以及森林病虫害等领域。国外学者利用分位数回归建立了树高−胸径关系模型^[3]。国内学者利用分位数回归拟合了树冠轮廓^[4]、树冠表面积及树冠体积^[5]，结合哑变量方法构建了大兴安岭落叶松（Larix gmelinii）的树高与胸径关系最优模型^[6]。Cao等^[7]提出利用组合分位数法预测火炬松（Pinus taeda）干形曲线，并通过插值系数修正削度方程以提高预测精度。

树木生长受气候、立地条件和经营措施等多种因素的影响和制约，不同样地间的树高−胸径关系存在较大差异，仅含胸径一个变量的树高模型不能描述不同林况下树高−胸径关系的差异，因此很多引入林分变量或者单木变量构建的广义模型也被用于描述不同林分的模型差异。除此之外，近年来混合效应模型在树高模型中也得到了一定的应用，可以反映总体平均变化趋势及个体之间的变异，不需要假设观测值相互独立^[8]，显著提高了模型的预测精度。Zhang等^[9]在以样地为随机效应的混合模型中引入林分密度和立地指数，结果表明该模型可以显著提高长白落叶松（Larix olgensis）树高−胸径模型的预测精度。燕云飞等^[10]基于广义混合效应构建了拟合效果最优的红松（Pinus koraiensis）人工林枝下高模型。

以往树高−胸径关系研究均选定某种方程进行非线性回归，且对多层次、多树种的混交林树高−胸径关系的描述相比纯林要困难和复杂很多^[11]。同时随着方程通用程度的提高，用于局部地区的误差也会增大^[12]。因此，本研究以吉林蛟河针阔混交林典型树种为研究对象，构建含有林分变量的广义树高−胸径模型，并基于基础模型和广义模型分布拟合不同树种的分位数回归和以样方效应作为分类变量的混合模型，对比分析各树种不同树高−胸径模型的预测效果和拟合差异，为温带针阔混交林资源调查、收获、经营与管理提供依据。

1. 研究区概况与研究方法

1.1 研究区概况

吉林省林业实验区国有林保护中心位于吉林省东部、长白山西麓，隶属于长白山系张广才岭山脉。行政区域处于吉林省蛟河市前进乡境内，属温带大陆性季风气候，年平均气温3.4 ℃，降水量708.8 mm，无霜期为120 ~ 130 d。地貌类型以低山丘陵区为主，海拔459 ~ 517 m。森林资源和植物种类丰富，植被类型为次生针阔混交林，主要乔木树种包括红松、水曲柳（Fraxinus mandshurica）、蒙古栎（Quercus mongolica）、紫椴（Tilia amurensis）、色木槭（Acer mono）、白牛槭（Acer mandshuricum）和胡桃楸（Juglans mandshurica）等。

1.2 数据来源与统计

在研究区设置总面积大小为21.12 hm²监测样地，经纬度为43°57′35″ ~ 43°57′54″N，127°43′37″ ~ 127°44′09″E。2009年对植被开展初次调查，2014年、2019年对样地进行复测。调查时将样地划分为528个20 m × 20 m的连续调查样方，记录所有样方中胸径大于1 cm的木本植物种类，测量胸径、树高、冠幅、坐标、林分郁闭程度等。基于样地内树种组成（表1）和各树种树高−胸径模型的初步拟合结果，选择重要值最高的乔木树种作为研究样本，其中筛选出1 531株红松、3 066株色木槭、1 822株紫椴和1 856株水曲柳。建模时将各组数据随机分成两部分，其中80%作为训练数据，20%作为测试数据（表2）。根据调查数据，计算各样方的林分密度、胸高断面积和优势木高（表3）。在每个20 m × 20 m样方内，分别选择1 ~ 3株最高的林木计算平均值，作为该树种在该样方的优势木高。利用R软件的quantreg包和nlme包进行非线性分位数回归和混合效应模型分析。

表 1 优势树种主要指标统计

Table 1. Main indicator statistics of dominant tree species

树种 Tree species	重要值 Important value	多度 Abundance	频度 Frequency	胸高断面积/（m²·hm⁻²） Basal area/（m²·ha⁻¹）
色木槭 Acer mono	11.42	3 071	505	87.58
水曲柳 Fraxinus mandshurica	11.13	1 861	420	119.81
紫椴 Tilia amurensis	9.46	1 825	472	84.91
红松 Pinus koraiensis	8.21	1 535	411	74.72
暴马丁香 Syringa reticulata	7.28	3 483	466	4.66
春榆 Ulmus davidiana	6.79	2 279	446	26.94
拧筋槭 Acer triflorum	6.51	1 474	444	41.76
蒙古栎 Quercus mongolica	5.62	646	337	55.80
白牛槭 Acer mandshuricum	4.67	1 492	358	15.56
白桦 Betula platyphylla	4.44	876	249	36.81

下载: 导出CSV

| 显示表格

表 2 单木树高−胸径模型拟合及检验数据

Table 2. Fitting and testing data of individual tree height-DBH model

数据分类 Dataset classification	树种 Tree species	样本数 Number of sample	胸径 DBH/cm				树高 Tree height/m
数据分类 Dataset classification	树种 Tree species	样本数 Number of sample	平均值 Mean	最小值 Min. value	最大值 Max. value	标准差 SD	平均值 Mean	最小值 Min. value	最大值 Max. value	标准差 SD
拟合数据 Fitting data	红松 Pinus koraiensis	1 226	19.14	1.60	75.80	15.90	12.25	1.00	33.40	6.75
	色木槭 Acer mono	2 452	14.13	1.20	88.80	12.62	11.22	2.00	37.20	6.10
	紫椴 Tilia amurensis	1 458	19.86	1.20	70.40	13.76	14.42	1.60	32.70	6.36
	水曲柳 Fraxinus mandshurica	1 484	26.17	2.00	75.00	12.32	17.82	2.70	27.30	4.15
检验数据 Testing data	红松 Pinus koraiensis	305	19.28	2.00	65.30	16.12	12.11	1.90	34.40	6.98
	色木槭 Acer mono	614	14.32	1.40	62.00	12.55	11.49	1.20	29.40	6.42
	紫椴 Tilia amurensis	364	20.12	1.70	65.80	14.60	14.45	1.80	34.00	6.68
	水曲柳 Fraxinus mandshurica	372	24.84	1.80	62.40	11.76	17.75	4.50	27.00	4.21

下载: 导出CSV

| 显示表格

表 3 林分调查因子统计表

Table 3. Statistical table of stand description factors

数据分类 Dataset classification	树种 Tree species	胸高断面积/（m²·hm⁻²） Basal area/（m²·ha⁻¹）				林分密度/（株·hm⁻²） Stand density/（tree·ha⁻¹）				优势木高 Dominant tree height/m
数据分类 Dataset classification	树种 Tree species	平均值 Mean	最小值 Min. value	最大值 Max. value	标准差 SD	平均值 Mean	最小值 Min. value	最大值 Max. value	标准差 SD	平均值 Mean	最小值 Min. value	最大值 Max. value	标准差 SD
拟合数据 Fitting data	红松 Pinus koraiensis	32.91	15.10	257.89	13.62	1 223.63	350	2 900	384.76	16.30	1.80	33.40	6.97
	色木槭 Acer mono	32.66	15.02	257.89	17.34	1 244.02	350	3 100	400.18	16.98	4.00	37.20	5.56
	紫椴 Tilia amurensis	34.00	15.02	257.89	14.38	1 284.93	350	2 900	387.05	19.62	3.40	34.00	4.79
	水曲柳 Fraxinus mandshurica	31.44	15.02	257.89	15.41	1 420.70	350	3 100	447.84	24.21	2.80	35.30	4.49
检验数据 Testing data	红松 Pinus koraiensis	33.49	19.21	58.18	7.35	1 215.16	450	2 575	346.34	16.88	2.00	33.40	7.14
	色木槭 Acer mono	33.46	16.19	257.89	16.51	1 258.15	450	2 900	374.19	17.23	3.20	37.20	5.31
	紫椴 Tilia amurensis	33.82	15.02	257.89	16.92	1 310.16	600	2 900	412.81	19.67	6.20	34.00	5.15
	水曲柳 Fraxinus mandshurica	31.91	15.10	257.89	21.16	1 488.58	600	3 100	443.16	24.95	15.20	34.80	3.97

下载: 导出CSV

| 显示表格

1.3 研究方法

1.3.1 基础模型与广义模型

以往大多选定某种非线性方程描述树种的树高−胸径关系，而混交林相对于纯林或年龄结构和林层单一的林分来说，其树高与胸径的关系更为复杂^[13]。根据以往的研究，基于树高−胸径散点图和树种的分布特征，选择以下11个常用的树高模型作为候选基础模型（表4），对树高−胸径分布数据进行非线性拟合，比较各模型的拟合精度，筛选预测精度最高的作为研究区树高−胸径的最优基础模型。

表 4 候选树高−胸径关系模型

Table 4. Candidate models of tree height-DBH relation

模型编号 Model No.	表达式 Equation	参考文献 Reference
M1	$H=1.3 + {\beta }_{1}{D}^{{\beta }_{2}}$	[14]
M2	$H=1.3+\beta_1\left[\dfrac{D}{1+D}\right]^{\beta_2}$	[15]
M3	$H=1.3+\exp\left(\beta_1+\dfrac{\beta_2}{D+1}\right)$	[16]
M4	$H=1.3 + {\beta }_{1}\left[1-\exp\left(-{\beta }_{2}D\right)\right]$	[17]
M5	$H=1.3 + {\beta }_{1}\exp\left(\dfrac{{\beta }_{2}}{D}\right)$	[18]
M6	$H=1.3 + {{\beta }_{1}[1-\exp(-{\beta }_{2}D\left)\right]}^{{\beta }_{3}}$	[19]
M7	$H=1.3 + {\beta }_{1}{D}^{\left[{\beta }_{2}{D}^{(-{\beta }_{3})}\right]}$	[20]
M8	$H=1.3+\exp\left(\beta_1+\dfrac{\beta_2}{D+\beta_3}\right)$	[21]
M9	$H=1.3 + {\beta }_{1}\exp\left[-{\beta }_{2}\exp\left(-{\beta }_{3}D\right)\right]$	[22]
M10	$H=1.3+\dfrac{D^2}{\beta_1+\beta_2D+\beta_3D^2}$	[23]
M11	$H=1.3+\dfrac{\beta_1}{1+\beta_2\exp\left(-\beta_3D\right)}$	[24]
注：H为树高预测值；D为胸径；β₁、β₂、β₃为模型参数。Notes: H is the predicted tree height; D is the DBH; β₁, β₂ and β₃ are the model parameters.

下载: 导出CSV

| 显示表格

但在结构更加复杂的针阔混交林中，仅仅将胸径作为预测变量，无法反映其他林分变量对树高的影响。本研究采用再参数化的方法建立树高−胸径模型，依据林分变量与树高之间的关系，适当融合林分密度、胸高断面积和优势木高度等变量。具体做法如下：基于所选择的最优基础模型，对林分变量与树高进行相关性分析；比较不同变量引入对模型的影响，筛选模型中的其他变量，确定与树高相关性较大且能显著提高模型预测能力的变量作为协变量；基于最优基础模型构建各树种预测精度最高的广义模型。

1.3.2 分位数回归

分位数回归是利用解释变量估计响应变量的条件分位数分布，全面刻画整个条件分布的综合回归方法^[25−26]。不仅可以度量响应变量对分布中心的影响，而且可以度量对分布上尾和下尾的影响。最小二乘法假定误差服从正态分布，而分位数回归的响应变量的平均预测值对残差分布没有特殊要求^[27]，从而确保参数估计的稳健性和模型拟合的灵活性。最小二乘回归的目标是最小化误差平方和，分位数回归的核心思想是从均值推广到分位数，也是通过最小化残差绝对值的非对称损失函数来估计分为点处参数值。

$\min\left[{\sum} _{{y}_{\tau }\geqslant {\hat{y}}_{\tau }}\tau \left({y}_{\tau }-{\hat{y}}_{\tau }\right) + {\sum} _{{y}_{\tau } < {\hat{y}}_{\tau }}(1-\tau )\left({\hat{y}}_{\tau }-{y}_{\tau }\right)\right]$

(1)

式中：τ表示要估计的分位数， ${y}_{\tau }$ 和 ${\hat{y}}_{\tau }$ 分别为响应变量在分位数τ处的观测值和估计值。

分位数组合法利用抽样数据拟合不同组合的分位数曲线，基于相邻两条分位数回归曲线插值得到修正的分位数曲线。假设第i个样方第j个树木的胸径值为 ${x}_{ij}$ ，树高观测值为 ${y}_{ij}$ 分布在第m和（m + 1）分位数估计值之间，即 ${\hat{y}}_{m}\left({x}_{ij}\right)$ ≤ ${y}_{ij}$ ≤ ${\hat{y}}_{m + 1}\left({x}_{ij}\right)$ 。使用插值法计算通过该点的修正曲线。

${\hat{y}}_{ij}=\alpha {\hat{y}}_{m}\left({x}_{ij}\right) + (1-\alpha ){\hat{y}}_{m + 1}\left({x}_{ij}\right)$

(2)

式中： $\alpha$ = $\dfrac{{\hat{y}}_{m + 1}\left({x}_{ij}\right)-{y}_{ij}}{{\hat{y}}_{m + 1}\left({x}_{ij}\right)-{\hat{y}}_{m}\left({x}_{ij}\right)}$ 表示插值系数， ${\hat{y}}_{ij}$ 为树高估计值， ${\hat{y}}_{m}\left({x}_{ij}\right)\mathrm{和}{\hat{y}}_{m + 1}\left({x}_{ij}\right)$ 分别为胸径为 ${x}_{ij}$ 的树木在第m和（m + 1）分位数处的树高估计值。

当抽样数量大于1时，在各分位数组合中选择使平均误差值符号发生改变的相邻曲线。当胸径值大于最大分位数回归的预测值时，将 $\hat{y}$ _m和 $\hat{y}$ _{m + 1}分别定义为 $\hat{y}$ _{m − 1}和 $\hat{y}$ _m，之后利用公式（2）得到树高曲线。

由于基础模型只有一个预测变量缺乏其他变量信息，很难完整描述响应变量在不同分位点的分布。本研究选择9个分位点（τ = 0.1，0.2，0.3，0.4，0.5，0.6，0.7，0.8，0.9），基于所选的最优基础模型拟合树高−胸径曲线，同时使用三分位数、五分位数和九分位数组合法得到树高修正曲线，通过模型评价和比较得到基于最佳分位数的基础模型，进而在该模型中融入林分变量构建广义分位数模型。

1.3.3 非线性混合效应模型

在筛选出的最优基础模型和广义模型加入随机效应构建非线性混合模型，本研究中选择样方为随机效应，混合效应模型一般形式可表示为

$\hat{\boldsymbol{H}}_{ij}=f\left(\hat{\boldsymbol{\phi}}_i,\hat{\boldsymbol{D}}_{ij}\right)+\boldsymbol{\varepsilon}_{ij},\ {\boldsymbol{\varepsilon}}_{ij}\sim{{{{\rm{N}}}}}(0,\ \sigma^2)$

(3)

式中： $\hat{\boldsymbol{H}}_{ij}$ 和 $\hat{\boldsymbol{D}}_{ij}$ 分别为第i个样方第j株树的树高和胸径预测向量，f为包含树高和胸径变量的函数表达式， $\hat{\boldsymbol{\phi}}_i$ 为混合效应参数向量，ε_ij为误差项。

参数向量 $\hat{\boldsymbol{\phi}}_i$ 的固定效应参数和随机效应参数可表示为

${\boldsymbol{\phi}}_i=\hat{\boldsymbol{X}}_i \hat{\boldsymbol{\beta}}+ {\hat{\boldsymbol{Z}}_i} {\hat{\boldsymbol{u}}_i},\; {\hat{\boldsymbol{u}}_i} \sim {\text{N}}(0,\; {\text{φ}})$

(4)

式中： $\hat{\boldsymbol{\beta }}$ 为固定效应参数向量， ${\hat{\boldsymbol{u}}}_{i}$ 为第i个样方的随机效应参数向量，遵循均值为0、协方差矩阵为 ${\text{φ}}$ 的正态分布， ${\hat{\boldsymbol{X}}}_{i}$ 和 ${\hat{\boldsymbol{{Z}}}}_{i}$ 分别是 $\hat{\boldsymbol{\beta }}$ 和 ${\hat{\boldsymbol{u}}}_{i}$ 的设计矩阵。

非线性混合效应模型中，方差−协方差结构由包括相关因子和加权因子的矩阵R_i来表示。

${\boldsymbol{R}}_{i}={\sigma }^{2}{\boldsymbol{G}}_{i}^{0.5}{\boldsymbol{\varGamma }}_{i}{\boldsymbol{G}}_{i}^{0.5}$

(5)

式中：σ²指模型的残差方差值，Γ_i为组内误差相关性结构，G_i为描述样方内方差异质性的对角矩阵，其对角元素由方差函数提供^[28]。

随机效应的方差−协方差结构（D）反映了样方间的差异，矩阵因随机参数个数的不同也会有不同的形式，本研究中选用广义正定矩阵结构，当混合模型中具有两个随机参数时表达式如下

$\boldsymbol{D}=\left[\begin{array}{cc}{\sigma }_{{u}_{1}}^{2}& {\sigma }_{{u}_{1}{u}_{2}}\\ {\sigma }_{{u}_{1}{u}_{2}}& {\sigma }_{{u}_{2}}^{2}\end{array}\right]$

(6)

式中： ${u}_{1}$ 、 ${u}_{2}$ 为随机参数， ${\sigma }_{{u}_{1}}^{2}$ 、 ${\sigma }_{{u}_{2}}^{2}$ 分别为 ${u}_{1}$ 、 ${u}_{2}$ 的方差， ${\sigma }_{{u}_{1}{u}_{2}}$ 为 ${u}_{1}$ 和 ${u}_{2}$ 的协方差。

混合效应模型中固定效应部分的检验方法与传统模型相同，对于随机效应的检验则需要计算随机参数值，本研究需计算在拟合数据中不涉及的样方的随机参数，利用式7^[29]进行计算。

$\hat{u}_i\approx\hat{D}\hat{Z}_i^{\mathrm{T}}\left(\hat{Z}_i\hat{D}\hat{Z}_i^{\mathrm{T}}+\hat{R}_i\right)^{-1}\hat{e}_i$

(7)

式中： $\hat{\boldsymbol{D}}$ 为随机效应参数的方差协方差矩阵， ${\hat{\boldsymbol{R}}}_{i}$ 为样方内方差协方差矩阵， ${\hat{\boldsymbol{Z}}}_{i}$ 为设计矩阵， ${\hat{\boldsymbol{Z}}}_{i}^{\mathrm{T}}$ 为转置矩阵， ${\hat{e}}_{i}$ 为实际值减去用固定效应参数计算的预测值。

1.3.4 模型评价与检验

研究中通过决定系数（R²）、平均绝对误差（MAE）和均方根误差（RMSE）评价和比较模型拟合结果，并利用未参与建模的20%独立样本检验11个候选非线性模型，上述评价指标的计算公式。

${R}^{2}=1-{\sum} _{i,j=1}^{n}{\left({H}_{ij}-{\hat{H}}_{ij}\right)}^{2}/{\sum} _{i,j=1}^{n}{\left({H}_{ij}-{\overline{H}}_{ij}\right)}^{2}$

(8)

$\mathrm{M}\mathrm{A}\mathrm{E}={\sum} _{i,j=1}^{n}\left|{H}_{ij}-{\hat{H}}_{ij}\right|/n$

(9)

$\mathrm{R}\mathrm{M}\mathrm{S}\mathrm{E}=\sqrt{{\sum} _{i,j=1}^{n}{\left({H}_{ij}-{\hat{H}}_{ij}\right)}^{2}/n}$

(10)

式中： ${H}_{ij}$ 和 ${\hat{H}}_{ij}$ 为第i个样方第j个样本的树高实际值和预测值， ${\overline{H}}_{ij}$ 为预测值的均值；n 为样本数。

除考虑以上指标外，还选择赤池信息准则（AIC）、贝叶斯信息准则（BIC）和似然比检验（LRT）对混合效应模型进行评价，AIC和BIC数值越小则表明模型拟合精度越高，LRT得到的P值若 < 0.000 1，则认为两个模型差异显著。

$\mathrm{AIC}=-2\mathrm{\mathit{L}}+2\lambda$

(11)

$\mathrm{BIC}=-2\mathrm{\mathit{L}}+\lambda\mathrm{ln}\ n$

(12)

式中：L为模型的对数似然值，λ 为固定效应参数的个数。

2. 结果与分析

2.1 基础模型拟合

基于红松、色木槭、紫椴和水曲柳的建模数据，对表4中的11个基础模型进行拟合，比较其MAE、RMSE和R²值（表5）。结果表明，对于4个树种的树高−胸径关系拟合，各模型均具有良好表现。MAE范围为1.887 ~ 2.818，RMSE范围为2.557 ~ 3.582，R²则为0.552 ~ 0.811，其中红松、紫椴水曲柳以M6即Richards模型拟合结果最优，色木槭的Richards模型拟合结果与其最优模型相差极小。使用检验数据对拟合模型进行评价比较，得到相同结果。且Richards方程生物学意义明确、灵活性较高易收敛，被认为很适合描述树高与胸径之间的关系^[30−31]，因此选择Richards作为4个树种的基础模型。

表 5 候选模型拟合与评价

Table 5. Fitting and evaluation of candidate models

模型 Model	红松 Pinus koraiensis			色木槭 Acer mono			紫椴 Tilia amurensis			水曲柳 Fraxinus mandshurica
模型 Model	MAE	RMSE	R²	MAE	RMSE	R²	MAE	RMSE	R²	MAE	RMSE	R²
M1	2.381	3.153	0.780	2.118	2.846	0.781	2.818	3.582	0.684	2.248	2.774	0.552
M2	2.361	3.128	0.784	2.080	2.806	0.787	2.521	3.357	0.722	2.079	2.571	0.615
M3	2.211	2.982	0.803	1.926	2.697	0.803	2.503	3.344	0.724	2.083	2.575	0.614
M4	2.179	2.945	0.808	1.895	2.654	0.809	2.530	3.343	0.724	2.088	2.575	0.614
M5	2.279	3.032	0.797	2.030	2.772	0.793	2.547	3.374	0.719	2.076	2.568	0.616
M6	2.140	2.925	0.811	1.890	2.654	0.809	2.491	3.332	0.726	2.063	2.557	0.619
M7	2.149	2.936	0.809	1.887	2.653	0.809	2.495	3.337	0.725	2.069	2.562	0.617
M8	2.144	2.932	0.810	1.888	2.652	0.810	2.496	3.335	0.725	2.074	2.565	0.617
M9	2.174	2.945	0.808	1.931	2.691	0.804	2.513	3.348	0.723	2.077	2.570	0.615
M10	2.146	2.935	0.809	1.887	2.652	0.810	2.493	3.336	0.725	2.068	2.560	0.618
M11	2.231	2.991	0.802	1.986	2.742	0.796	2.553	3.381	0.718	2.091	2.587	0.610
注：MAE.平均绝对误差；RMSE.均方根误差；R².决定系数。下同。Notes: MAE, mean absolute error; RMSE, root mean squared error; R², coefficient of determination. The same below.

下载: 导出CSV

| 显示表格

2.2 广义模型构建

对表3的林分特征因子进行相关性分析，结果如表6所示。4个树种的树高和胸径与优势木高呈显著正相关关系（P < 0.01），色木槭和紫椴树高、胸径与林分密度呈显著负相关关系（P < 0.01）。因此将优势木高和林分密度两个变量分别引入基础模型构建广义模型，但包含林分密度因子的色木槭和紫椴树高方程不收敛，而引入胸高断面积的水曲柳树高方程也不收敛，这表明添加优势木高度可显著提高模型精度。因此本研究最优广义模型的表达式为

表 6 树高与林分变量相关性分析

Table 6. Correlation analysis between tree height and stand variables

树种 Tree species	因子 Factor	树高 Tree height	胸径 DBH	胸高断面积 Basal area	林分密度 Stand density	优势木高 Dominate tree height
红松 Pinus koraiensis	树高 Tree height	1.000
	胸径 DBH	0.855^**	1.000
	胸高断面积 Basal area	0.020	0.040	1.000
	林分密度 Stand density	0.010	0.006	0.092^**	1.000
	优势木高 Dominate tree height	0.587^**	0.440^**	0.069^*	0.018	1.000
色木槭 Acer mono	树高Tree height	1.000
	胸径 DBH	0.845^**	1.000
	胸高断面积 Basal area	0.031	0.030	1.000
	林分密度 Stand density	−0.284^**	−0.270^**	0.089^**	1.000
	优势木高 Dominate tree height	0.484^**	0.353^**	0.139^**	−0.261^**	1.000
紫椴 Tilia amurensis	树高 Tree height	1.000
	胸径 DBH	0.776^**	1.000
	胸高断面积 Basal area	0.068^**	0.064^**	1.000
	林分密度 Stand density	−0.207^**	−0.200^**	0.105^**	1.000
	优势木高 Dominate tree height	0.537^**	0.294^**	0.178^**	−0.175^**	1.000
水曲柳 Fraxinus mandshurica	树高Tree height	1.000
	胸径 DBH	0.684^**	1.000
	胸高断面积 Basal area	0.067^**	0.133^**	1.000
	林分密度 Stand density	−0.037	−0.081^**	0.084^**	1.000
	优势木高 Dominate tree height	0.256^**	0.264^**	−0.021^**	0.318^**	1.000
注：^** 表示两个变量在 P < 0.01 水平上显著相关，^* 表示两个变量在 P < 0.05 水平上显著相关。Notes:^** means significant correlation at P < 0.01 level between two variables, ^* means significant correlation at P < 0.05 level between two variables.

下载: 导出CSV

| 显示表格

$H=1.3+(\beta_1+a_1H_{\mathrm{D},i})\left[1-\exp\left(-\beta_2D\right)\right]^{\beta_3}$

(13)

式中：H_D为某个树种的优势木高，H_D,i为第i个样方的优势木高， ${\beta }_{1}$ 、 ${\beta }_{2}$ 、 ${\beta }_{3}$ 、 ${a}_{1}$ 为模型参数。

2.3 分位数回归

在不同分位点处（τ = 0.1，0.2，0.3，0.4，0.5，0.6，0.7，0.8，0.9），基于Richards方程利用分位数回归分别拟合树高−胸径关系模型（表5）。利用回归评价指标检验不同分位数模型对观测值的拟合程度（表7），基础模型及分位数回归模型的决定系数R²值均在0.6以上，表明各分位点的树高方程均具有较好的拟合效果。其中τ = 0.5的中位数回归与非线性回归的拟合效果基本一致，反映了分位数回归模型的灵活性。

表 7 分位数回归模型拟合精度

Table 7. Goodness-of-fit tests for quantile regression models

分位数 Quantile	红松 Pinus koraiensis			色木槭 Acer mono			紫椴 Tilia amurensis			水曲柳 Fraxinus mandshurica
分位数 Quantile	MAE	RMSE	R²	MAE	RMSE	R²	MAE	RMSE	R²	MAE	RMSE	R²
Richards	2.140	2.925	0.811	1.890	2.654	0.809	2.491	3.332	0.726	2.063	2.557	0.619
0.1	3.415	4.497	0.780	3.103	4.105	0.770	4.029	5.166	0.696	3.426	4.156	0.614
0.2	2.779	3.826	0.793	2.460	3.406	0.793	3.140	4.238	0.715	2.680	3.387	0.615
0.3	2.368	3.322	0.805	2.081	2.963	0.804	2.742	3.791	0.720	2.323	2.976	0.615
0.4	2.179	3.063	0.809	1.924	2.764	0.807	2.520	3.493	0.723	2.116	2.693	0.615
0.5	2.122	2.942	0.811	1.873	2.666	0.809	2.461	3.374	0.724	2.056	2.583	0.617
0.6	2.191	2.953	0.810	1.918	2.675	0.808	2.542	3.363	0.724	2.119	2.596	0.616
0.7	2.440	3.181	0.807	2.161	2.897	0.803	2.830	3.630	0.719	2.372	2.846	0.614
0.8	2.912	3.690	0.797	2.577	3.354	0.791	3.388	4.268	0.697	2.887	3.422	0.615
0.9	4.081	5.027	0.753	3.451	4.293	0.762	4.629	5.519	0.679	3.965	4.582	0.602

下载: 导出CSV

| 显示表格

利用未参与建模的20%测试数据检验模型的预测能力（表8）。分位数组合法分别采用三分位数组合（τ = 0.1，0.5，0.9）、五分位数组合（τ = 0.1，0.3，0.5，0.7，0.9）和九分位数组合（τ = 0.1，0.2，0.3，0.4，0.5，0.6，0.7，0.8，0.9）。随机抽样或抽取平均木时幼树会带来较大预测误差，基于王君杰等^[32]的研究结果，选取10株最大树木计算插值系数进行预测。结果表明：对于分位数组合法，三分位数和五分位数组合的预测精度高于九分位数组合；其中红松树高的拟合效果略优于中位数回归，但其他树种模型预测精度均低于中位数回归。因此，本研究选择中位数为4个树种的最优分位数树高曲线式（13）构建广义分位数模型。

表 8 分位数模型验证评价结果

Table 8. Quantile model validation evaluation results

方法 Method	红松 Pinus koraiensis		色木槭 Acer mono		紫椴 Tilia amurensis		水曲柳 Fraxinus mandshurica
方法 Method	RMSE	MAE	RMSE	MAE	RMSE	MAE	RMSE	MAE
中位数回归 Median regression	2.963	2.094	2.898	2.020	3.540	2.549	2.560	2.050
九分位数组合 Nine quantile combination	2.956	2.118	2.997	2.185	3.510	2.761	2.601	2.150
五分位数组合 Five quantile combination	2.927	2.095	2.971	2.155	3.507	2.758	2.602	2.152
三分位数组合 Triquartile combination	2.926	2.092	2.983	2.162	3.596	2.851	2.592	2.139

下载: 导出CSV

| 显示表格

表 11 树高−胸径模型拟合评价统计量

Table 11. Fitting and evaluation statistics of tree height-DBH models

树种 Tree species	指标 Index	基础模型 Base model	广义模型 Generalized model	中位数回归 Median quantile regression		非线性混合效应 Nonlinear mixed-effects
树种 Tree species	指标 Index	基础模型 Base model	广义模型 Generalized model	简单模型 Simple model	广义模型 Generalized model	简单模型 Simple model	广义模型 Generalized model
红松 Pinus koraiensis	MAE	2.140	1.669	2.122	1.666	1.259	1.358
	RMSE	2.925	2.225	2.942	2.228	1.687	1.826
	R²	0.811	0.891	0.811	0.891	0.937	0.927
色木槭 Acer mono	MAE	1.890	1.575	1.873	1.568	1.258	1.359
	RMSE	2.654	2.159	2.666	2.166	1.740	1.875
	R²	0.809	0.875	0.809	0.874	0.919	0.906
紫椴 Tilia amurensis	MAE	2.491	1.722	2.461	1.706	1.399	1.611
	RMSE	3.332	3.379	3.374	2.401	1.955	2.235
	R²	0.726	0.860	0.724	0.858	0.906	0.877
水曲柳 Fraxinus mandshurica	MAE	2.063	2.041	2.056	2.031	2.004	2.527
	RMSE	2.557	2.524	2.583	2.536	2.478	2.038
	R²	0.619	0.629	0.617	0.627	0.643	0.629

下载: 导出CSV

| 显示表格

2.4 非线性混合效应模型构建

在基础模型和广义模型基础上考虑样方效应对树高的影响，在4个树种的基础模型上增加样方为随机效应，共8种情况收敛（表9），红松、紫椴和水曲柳增加1个随机效应参数时拟合效果最好，而色木槭引入2个随机参数的混合模型预测精度更高，模型表达式分别为

表 9 混合效应模型验证评价结果

Table 9. Verification and evaluation results of mixed effect model

树种 Tree species	基础混合效应模型 Base mixed-effect model						广义混合效应模型 Generalized mixed-effect model
树种 Tree species	随机参数 Random parameter	AIC	BIC	L	LRT	P	随机参数 Random parameter	AIC	BIC	L	LRT	P
红松 Pinus koraiensis	${u}_{1}$	5 619.3	5 644.8	−2 804.6			${u}_{1}$	5 346.9	5 377.5	−2 667.4
	${u}_{1}$ , ${u}_{2}$	5 643.2	5 678.9	−2 814.6	19.878 2	< 0.001	${u}_{3}$	5 354.2	5 384.8	−2 671.1
							${u}_{1}$ , ${u}_{3}$	5 350.9	5 391.8	−2 667.5	7.238 0	0.026 8
色木槭 Acer mono	${u}_{2}$	10 966.2	10 995.2	−5 478.1			${u}_{1}$	10 597.5	10 632.3	−5 292.7
	${u}_{1}$ , ${u}_{2}$	10 962.2	11 002.9	−5 474.1	7.964 9	0.018 6	${u}_{3}$	10 602.8	10 637.6	−5 295.4
							${u}_{1}$ , ${u}_{3}$	10 594.2	10 640.6	−5 289.1	12.571 9	0.001 9
							${u}_{1}$ , ${u}_{2}$	10 601.4	10 647.8	−5 292.8
紫椴 Tilia amurensis	${u}_{1}$	7 109.6	7 136.0	−3 549.8			${u}_{1}$	6 662.5	6 694.2	−3 325.2
							${u}_{3}$	6 661.8	6 693.5	−3 324.9
							${u}_{1},{u}_{3}$	6 665.8	6 708.0	−3 324.9	4.415 0	0.998 7
水曲柳 Fraxinus mandshurica	${u}_{1}$	7 007.8	7 034.4	−3 498.9			${u}_{1}$	6 966.0	6 997.8	−3 477.0
	${u}_{2}$	7 009.0	7 035.5	−3 499.5			${u}_{3}$	6 964.3	6 996.1	−3 476.1
	${u}_{1}$ , ${u}_{2}$	7 011.8	7 049.0	−3 498.9	1.126 9	0.569 2	${u}_{1},{u}_{3}$	6 964.9	7 007.4	−3 474.5	3.322 9	0.189 9
注：AIC.赤池信息准则；BIC.贝叶斯信息准则；L.对数似然值；LRT.似然比检验。Notes: AIC, Akaike information criterion; BIC, Bayesian information criterion; L, log-likelihood value; LRT, likelihood ratio test.

下载: 导出CSV

| 显示表格

$H=1.3 + {({\beta }_{1} + {u}_{1i})[1-\exp(-{\beta }_{2}D\left)\right]}^{{\beta }_{3}}$

(14)

$H=1.3 + {({\beta }_{1} + {u}_{1i})[1-\exp(-{\beta }_{2}D\left)\right]}^{{(\beta }_{3 + }{u}_{2i})}$

(15)

式中： ${u}_{1i}$ 、 ${u}_{2i}$ 为样方水平的随机参数。

在广义模型基础上，引入不同组合形式的随机效应作为自变量构建广义混合效应模型，4个树种共有13种情况收敛（），当引入3个以上随机参数时，模型不收敛；仅引入参数 ${u}_{1}$ 的红松树高模型和仅引入参数 ${u}_{3}$ 的紫椴及水曲柳树高模型AIC、BIC值最小，且L值达到最大，而色木槭以随机效应作用于 ${u}_{1}$ 和 ${u}_{3}$ 两个参数时模型预测精度最高，一般表达式分别为

$H=1.3+(\beta_1+u_{1i}+a_1H_{\mathrm{D},\mathit{\mathrm{\mathit{i}}}})\left[1-\exp\left(-\beta_2D\right)\right]^{\beta_3}$

(16)

$H=1.3+\left[\beta_1+u_{1i}+(a_1+u_{3i})H_{\mathrm{D},\mathrm{\mathit{i}}}\right]\left[1-\exp\left(-\beta_2D\right)\right]^{\beta_3}$

(17)

$H=1.3+\left[\beta_1+(a_1+u_{3i})H_{\mathrm{D},\rm{\mathit{i}}}\right]\left[1-\exp\left(-\beta_2D\right)\right]^{\beta_3}$

(18)

式中： ${u}_{1i}$ 、 ${u}_{3i}$ 为样方水平的随机参数。

选择各树种拟合效果最好的混合效应模型，参数估计值见表10。

表 10 混合效应模型参数估计

Table 10. Parameter estimates of mixed-effect models

参数 Parameter	基础混合效应模型 Base mixed-effect model				广义混合效应模型 Generalized mixed-effect model
参数 Parameter	红松 Pinus koraiensis	色木槭 Acer mono	紫椴 Tilia amurensis	水曲柳 Fraxinus mandshurica	红松 Pinus koraiensis	色木槭 Acer mono	紫椴 Tilia amurensis	水曲柳 Fraxinus mandshurica
公式编号 Equation No.	（14）	（15）	（14）	（14）	（16）	（17）	（18）	（18）
a	21.192（0.387^***）	18.626（0.251^***）	19.366（0.263^***）	20.021（0.190^***）	9.186（0.535^***）	7.776（0.467^***）	4.522（0.468^***）	16.513（0.523^***）
b	0.065（0.004^***）	0.085（0.004^***）	0.100（0.005^***）	0.101（0.007^***）	0.071（0.004^***）	0.092（0.004^*）	0.104（0.005^***）	0.110（0.007^***）
c	1.366（0.056^***）	1.142（0.033^***）	1.367（0.066^***）	1.445（0.126^***）	1.305（0.057^***）	1.142（0.034^***）	1.322（0.066^***）	1.567（0.142^***）
a₁					0.577（0.024^***）	0.554（0.023^***）	0.715（0.022^***）	0.132（0.019^***）
${\mathrm{\sigma }}_{{u}_{1}}^{2}$	14.927 2	10.920 5	11.410 7	0.201 9	2.877 0	14.850 5
${\mathrm{\sigma }}_{{u}_{2}}^{2}$		0.011 9
${{\mathrm{\sigma }}^{2}}_{{u}_{3}}$						0.038 0	0.001 7	0.000 4
${\mathrm{\sigma }}_{{u}_{1}{u}_{2}}$		0.162 1
${\mathrm{\sigma }}_{{u}_{1}{u}_{3}}$						−0.718 2
${\mathrm{\sigma }}^{2}$	3.637 0	3.638 8	4.908 0	6.422 3	3.811 3	3.858 9	5.275 9	6.201 5
注：^*表示在 P < 0.001 水平上显著。Note: ^* means significant at P < 0.001 level.

下载: 导出CSV

| 显示表格

2.5 模型评价与检验

4个树种的各模型拟合统计量见表11。结果表明：基于混合效应的模型精度明显高于不含随机参数的模型；在不含随机效应的模型中，中位数回归形式和一般形式的广义模型精度差异较小；广义中位数模型在预测精度上高于基础模型的中位数回归，红松、色木槭、紫椴和水曲柳的模型R²分别提高了9.9%、8.0%、18.5%和1.6%，MAE值分别降低了27.4%、19.5%、44.3%和1.2%，RMSE值分别下降了32.0%、23.1%、40.5%和1.9%。在增加随机效应的模型中，基础混合模型的拟合结果略优于广义混合模型，4个树种R²仅分别提高了1.1%、1.4%、3.3%和2.2%，但两者并未有显著差异。红松、色木槭、紫椴和水曲柳的基础混合效应和广义混合效应模型的残差分布图如图1所示，残差分布较为均匀，没有出现明显的不规则形状，这说明各树种的混合模型均没有明显异方差现象。

图 1 基础混合效应模型（A1、B1、C1、D1）和广义混合效应模型（A2、B2、C2、D2）的残差分布图

Figure 1. Residual plots of base mixed-effect models (A1, B1, C1, D1) and generalized mixed-effect models (A2, B2, C2, D2)

下载: 全尺寸图片幻灯片

混合效应模型中随机效应部分的检验需要估算随机参数值，本研究以20%的样木作为检验数据，利用公式（7）分别计算未包含在拟合数据的样方的随机参数；同时与基础模型、广义模型和分位数模型的预测精度进行比较，检验结果如表12。可以看出，混合效应模型的MAE和RMSE值均小于其他模型，拟合效果相较分位数回归得到了显著提高，其中基础混合模型相比于广义混合模型预测精度更高。

表 12 树高−胸径模型统计检验

Table 12. Goodness-of-fit statistics of tree height-DBH models

树种 Tree species	指标 Index	基础模型 Base model	广义模型 Generalized model	中位数回归 Median quantile regression		非线性混合效应 Nonlinear mixed-effects
树种 Tree species	指标 Index	基础模型 Base model	广义模型 Generalized model	简单模型 Simple model	广义模型 Generalized model	简单模型 Simple model	广义模型 Generalized model
红松 Pinus koraiensis	MAE	2.128	2.119	2.094	2.163	1.600	1.856
红松 Pinus koraiensis	RMSE	2.963	3.024	2.963	3.063	2.216	2.635
色木槭 Acer mono	MAE	2.018	1.906	2.020	2.052	1.620	1.712
色木槭 Acer mono	RMSE	2.867	2.782	2.898	2.944	2.341	2.529
紫椴 Tilia amurensis	MAE	2.571	1.685	2.549	2.697	1.609	1.635
紫椴 Tilia amurensis	RMSE	3.471	2.349	3.540	3.754	2.058	2.206
水曲柳 Fraxinus mandshurica	MAE	2.041	2.037	2.050	2.051	2.039	2.049
水曲柳 Fraxinus mandshurica	RMSE	2.526	2.517	2.560	2.561	2.528	2.534

下载: 导出CSV

| 显示表格

3. 讨　　论

3.1 基于基础模型的分位数回归

分位数回归既能描述均值条件下树高的分布趋势，也能描述特定分位点树高−胸径曲线的形状变化^[33]。传统的最小二乘法易受残差分布限制，与之相比分位数回归具有更加稳健的参数估计值，模型稳定性和灵活性也更强，能够避免选取数据的人为影响。在不同分位点拟合树高−胸径模型，同时基于参数估计和建模样本计算三分位数、五分位数和九分位数组合的插值系数，王君杰等^[34]最近发现中位数回归优于非线性回归；本文与之不同发现中位数回归与非线性回归的拟合效果基本一致。经过对11个非线性模型的评价比较，选择Richards方程作为基础模型进行分位数回归，其中中位数回归拟合效果最好，4个树种的R²值均大于0.6，对树高的预测能力不逊于非线性回归，这表明Richards方程能广泛适用树高−胸径模型的拟合。

三分位数组合的预测效果均优于五分位数和九分位数组合，尤其在红松树高的预测中，两类分位数组合的预测精度相较中位数和非线性回归均有提高；以往研究显示五分位数组合对树高曲线和削度方程的拟合要优于三分位数组合，但都没有考虑抽取方案对分位数组合的影响^[35]。因此抽取方案的不同会导致分位数组合之间的差异不同，总体上三分位数组合（τ = 0.1，0.5，0.9）的预测能力较好且效果稳定，本研究中选取多个分位数组合也并未使预测结果有明显提高^[32]。

3.2 引入林分变量和随机效应对树高模型的影响

在林分生长过程中，有学者利用含有优势木胸径、树高和林分密度等因子的模型来描述树高−胸径关系，模型拟合结果得到提升^[36]。为了比较不同样方间树高−胸径的变化规律，本研究对胸高断面积、林分密度和优势木高3个林分变量进行Pearson相关性分析，发现引入变量的广义模型的拟合效果均优于基础模型，当引入1个变量时，红松、色木槭、紫椴和水曲柳均仅增加优势木高变量的模型可以收敛，部分其他林分变量与树高有显著关系，但含优势木高的模型相较含林分密度或胸高断面积的广义模型对树高−胸径的关系影响更大；4个树种中水曲柳的广义模型相较基础模型的拟合效果提升幅度最小，R²仅提高0.01，这可能与水曲柳的直径分布有关，幼树数量较少，成熟林木较多，在林分中多位于林冠层，内部资源和空间竞争相对稳定，处于优势地位，这类大个体的树高不易受林分变量较大影响。这说明即使是在具有相似地形和气候条件的林分中，不同树种的树高−胸径关系可能不同。森林群落中特定的环境条件和树木之间的复杂联系可能会导致树高−胸径生长关系不同^[37]。优势木高反映了立地质量以及林分生长和收获，优势木高的参数估计值为正值，这表明由于立地质量的不同，优势木高与树高之间呈正相关关系，引入优势木高的广义模型更适用于树高−胸径模拟的拟合。

基于基础模型和各树种的最优广义模型，对应构建以样方水平为随机效应的混合模型，比较不同随机参数下各树种树高模型的拟合效果。研究发现，红松、紫椴和水曲柳基础混合效应模型均以增加随机参数 ${u}_{1}$ ，广义混合效应模型中红松增加参数 ${u}_{1}$ ，紫椴和水曲柳将 ${u}_{3}$ 同时作为随机参数，拟合效果最优；色木槭基础混合效应模型增加随机参数 ${u}_{1}$ 、 ${u}_{2}$ ，广义混合效应模型则是将 ${u}_{1}$ 、 ${u}_{3}$ 同时作为随机参数，预测精度最高。通过对各模型进行检验评价，结果表明混合效应模型对树高的预测效果优于固定效应模型，这与王冬至等^[38]的研究结果一致，表明考虑样方水平的随机影响能更好地描述树高生长规律。此外，本研究的模型评价和检验结果显示基础混合效应模型的预测能力略优于广义混合效应模型，但未有显著差异，同样的情况也出现在陈浩等^[39]对马尾松和周晏平等^[40]对樟子松的研究结果中，说明在增加样方效应后，林分变量对树高−胸径关系的影响被样方水平所涵盖，对混合效应模型的影响较弱。在Zang等^[41]对中国北部和东北落叶松人工林树高−胸径关系的研究中，基础混合效应模型比其他模型提供了更好的拟合和更稳定的估计，表明不需要额外的树木和林分变量。

4. 结　　论

本研究基于分位数回归和混合效应模型对阔叶红松林中优势树种红松、色木槭、紫椴和水曲柳的树高−胸径关系进行模型拟合，在11个非线性候选模型中确定Richards方程为基础模型，并引入优势木高构建广义模型，模型拟合结果得到较大提高；4个树种基于中位数回归的基础模型和广义模型拟合精度与基础模型的非线性回归相近。

加入样方水平为随机效应后，混合效应模型相较固定效应模型更具优越性，模型预测能力得到显著提高，而基础混合模型和广义混合模型的拟合优度差异较小。这表明林分变量和随机效应对树高模型的影响是显著的，在不考虑样方效应的情况下，可选择广义模型作为东北地区针阔混交林树高模型；若考虑样方效应，基础混合效应模型能提供更稳定的预测趋势和拟合结果。

图 1 各样方出现的幼苗物种数和个体数统计

Figure 1. Number of seedling species and individuals in each seedling quadrat

下载: 全尺寸图片幻灯片

图 2 幼苗的物种数及个体数在不同高度级上的分布

Figure 2. Distribution of seedling species and individuals at different height levels

下载: 全尺寸图片幻灯片

图 3 幼苗随海拔、坡度的分布

不同小写字母表示不同海拔、坡度间的差异显著（P < 0.05）。 Different lowercase letters indicate significant differences between different altitudes and slopes(P < 0.05).

Figure 3. Seedling distribution with altitude and slope

下载: 全尺寸图片幻灯片

图 4 幼苗密度的克里格空间插值分析

Figure 4. Kriging spatial interpolation analysis of seedling density

下载: 全尺寸图片幻灯片

表 1 3年调查到的幼苗科、属、种

Table 1 Seedling families, genera and species investigated in the past three years

年份 Year	所有幼苗 All seedlings	乔木幼苗 Tree seedling	灌木幼苗 Shrub seedling	木质藤本幼苗 Seedling of woody vine	幼苗数量 Seedling number
2017	55科96属121种55 families, 96 genera, 121 species	29科47属55种 29 families, 47 genera, 55 species	31科42属49种 31 families, 42 genera, 49 species	15科16属17种 15 families, 16 genera, 17 species	971
2018	40科66属78种 40 families, 66 genera, 78 species	25科38属44种 25 families, 38 genera, 44 species	15科21属24种 15 families, 21 genera, 24 species	8科10属10种 8 families, 10 genera, 10 species	767
2019	39科70属87种 39 families, 70 genera, 87 species	22科34属42种 22 families, 34 genera, 42 species	19科27属30种 19 families, 27 genera, 30 species	13科15属15种 13 families, 15 genera, 15 species	1 342
2017—2019	63科124属161种 63 families, 124 genera, 161 species	34科61属74种 34 families, 61 genera, 74 species	35科50属62种 35 families, 50 genera, 62 species	20科25属26种 20 families, 25 genera, 26 species	3 080

下载: 导出CSV

表 2 茂兰喀斯特森林木本植物幼苗的种类及数量特征

Table 2 Species and quantity characteristics of woody plant seedlings in karst forest of Maolan

树种 Species	科 Family	属 Genus	幼苗数量 Number of seedling			出现样方数 Number of quadrat presented			重要值 Important value
树种 Species	科 Family	属 Genus	2017	2018	2019	2017	2018	2019	2017	2018	2019
齿叶黄皮 Clausena dunniana	芸香科 Rutaceae	黄皮属 Clausena	38	36	67	30	24	31	4.30	4.98	4.78
杨梅叶蚊母树 Distylium myricoides	金缕梅科 Hamamelidaceae	蚊母树属 Distylium	45	30	27	27	16	14	4.43	3.71	2.04
针齿铁仔 Myrsine semiserrata	紫金牛科 Myrsinaceae	铁仔属 Myrsine	35	6	12	25	4	7	3.76	0.83	0.96
朴树 Celtis sinensis	大麻科 Cannabaceae	朴属 Celtis	30	16	17	25	13	15	3.50	2.47	1.74
狭叶润楠 Machilus rehderi	樟科 Lauraceae	润楠属 Machilus	38	24	34	19	15	13	3.44	3.21	2.23
南方桂樱 Laurocerasus australis	蔷薇科 Rosaceae	桂樱属 Laurocerasus	28	26	29	19	18	15	2.93	3.67	2.19
粗糠柴 Mallotus philippensis	大戟科 Euphorbiaceae	野桐属 Mallotus	13	11	22	13	9	14	1.69	1.70	1.85
广西密花树 Rapanea kwangsiensis	紫金牛科 Myrsinaceae	密花树属 Rapanea	18	4	20	9	2	12	1.63	0.48	1.63
榆树 Ulmus pumila	榆科 Ulmaceae	榆属 Ulmus	14	7	7	10	7	6	1.50	1.22	0.70
贵州鹅耳枥 Carpinus kweichowensis	桦木科 Betulaceae	鹅耳枥属 Carpinus	11	10	14	6	6	11	1.04	1.31	1.33
野柿 Diospyros kaki var. sylvestris	柿科 Ebenaceae	柿属 Diospyros	8	3	6	7	3	4	0.96	0.52	0.52
小叶柿 Diospyros mollis	柿科 Ebenaceae	柿属 Diospyros	6	15	35	6	12	24	0.78	2.29	3.07
西南卫矛 Euonymus hamiltonianus	卫矛科 Celastraceae	卫矛属 Euonymus	5	19	3	5	9	3	0.65	2.23	0.33
海桐 Pittosporum tobira	海桐科 Pittosporaceae	海桐属 Pittosporum	6	17	8	4	3	6	0.62	1.44	0.74
伞花木 Eurycorymbus cavaleriei	无患子科 Sapindaceae	伞花木属 Eurycorymbus	3	3	2	3	3	1	0.39	0.52	0.15
香叶树 Lindera communis	樟科 Lauraceae	山胡椒属 Lindera	2	13	25	2	7	14	0.26	1.62	1.96
圆叶乌桕 Triadica rotundifolia	大戟科 Euphorbiaceae	乌桕属 Triadica	2	2	9	2	1	5	0.26	0.24	0.70
梾木 Swida macrophylla	山茱萸科 Cornaceae	山茱萸属 Cornus	1	3	4	1	3	6	0.13	0.52	0.59
黄梨木 Boniodendron minus	无患子科 Sapindaceae	黄梨木属 Boniodendron	9	17	25	9	17	19	1.17	2.97	2.33
角叶槭 Acer sycopseoides	槭树科 Aceraceae	槭属 Acer	16	1	3	4	1	20	1.14	0.17	1.59
九里香 Murraya exotica	芸香科 Rutaceae	九里香属 Murraya	9	10	35	8	9	3	1.09	1.64	1.53
长叶冻绿 Rhamnus crenata	鼠李科 Rhamnaceae	鼠李属 Rhamnus	1	2	5	1	1	5	0.13	0.24	0.56
异叶花椒 Zanthoxylum dimorphophyllum	芸香科 Rutaceae	花椒属 Zanthoxylum	8	5	4	8	3	2	1.04	0.65	0.30
小叶女贞 Ligustrum quihoui	木犀科 Oleaceae	女贞属 Ligustrum	5	10	18	4	8	14	0.57	1.53	1.70
十大功劳 Mahonia fortunei	小檗科 Berberidaceae	十大功劳属 Mahonia	5	3	3	3	3	3	0.49	0.52	0.33
南天竹 Nandina domestica	小檗科 Berberidaceae	南天竹属 Nandina	45	18	17	24	10	10	4.20	2.27	1.37
长梗罗伞 Brassaiopsis glomerulata	五加科 Araliaceae	罗伞属 Brassaiopsis	9	3	5	5	2	4	0.85	0.41	0.48
棕竹 Rhapis excelsa	棕榈科 Arecaceae	棕竹属 Rhapis	13	6	7	7	4	3	1.22	0.83	0.48
九管血 Ardisia brevicaulis	紫金牛科 Myrsinaceae	紫金牛属 Ardisia	3	2	34	2	2	14	0.31	0.35	2.30
箬竹 Indocalamus tessellatus	禾本科 Poaceae	箬竹属 Indocalamus	3	12	21	2	2	3	0.31	1.00	1.00
硃砂根 Ardisia crenata	紫金牛科 Myrsinaceae	紫金牛属 Ardisia	5	7	1	3	4	1	0.49	0.89	0.11
苎麻 Boehmeria nivea	荨麻科 Urticaceae	苎麻属 Boehmeria	8	5	7	4	3	3	0.72	0.65	0.48
雀梅藤 Sageretia thea	鼠李科 Rhamnaceae	雀梅藤属 Sageretia	5	6	3	5	6	3	0.65	1.05	0.33
寒莓 Rubus buergeri	蔷薇科 Rosaceae	悬钩子属 Rubus	5	10	9	2	6	5	0.41	1.31	0.70
络石 Trachelospermum jasminoides	夹竹桃科 Apocynaceae	络石属 Trachelospermum	85	105	151	31	31	37	6.80	10.24	8.35
球兰 Hoya carnosa	夹竹桃科 Apocynaceae	球兰属 Hoya	16	5	12	6	5	5	1.29	0.87	0.82
菝葜 Smilax china	菝葜科 Smilacaceae	菝葜属 Smilax	23	17	40	13	13	25	2.20	2.53	3.33
珍珠莲 Ficus sarmentosa var. henryi	桑科 Moraceae	榕属 Ficus	19	24	106	13	15	27	2.00	3.21	5.94
常春藤 Hedera nepalensis var. sinensis	五加科 Araliaceae	常春藤属 Hedera	28	5	11	20	5	6	3.01	0.87	0.85
链珠藤 Alyxia sinensis	夹竹桃科 Apocynaceae	链珠藤属 Alyxia	1	19	40	1	12	15	0.13	2.55	2.60
小计 Sub-total			624	537	898	388	317	428	62.49	69.71	64.99
其他 Others			347	230	444	251	139	250	37.51	30.29	35.01

下载: 导出CSV

表 3 10个主要树种的幼苗物种新生和死亡数量组成

Table 3 Composition of seedling species regeneration and death of 10 main tree species

树种 Species	幼苗数量 Number of seedling			出现样方数 Number of quadrat presented			新生幼苗数量（新生率） Number of newborn seedling (newborn rate)		死亡幼苗数量（死亡率） Number of dead seedling (mortality rate)
树种 Species	2017	2018	2019	2017	2018	2019	2017—2018	2018—2019	2017—2018	2018—2019
齿叶黄皮 Clausena dunniana	38	36	67	30	24	31	29(80.6%)	48(71.6%)	31(81.6%)	17(47.2%)
粗糠柴 Mallotus philippensis	13	11	22	13	9	14	10(90.9%)	18(81.8%)	12(92.3%)	7(63.6%)
贵州鹅耳枥 Carpinus kweichowensis	11	10	14	6	6	11	10(100.0%)	9(64.3%)	11(100.0%)	5(50.0%)
黄梨木 Boniodendron minus	9	17	25	9	17	19	14(82.4%)	22(88.0%)	6(66.7%)	14(82.4%)
南方桂樱 Laurocerasus australis	28	26	29	19	18	15	22(84.6%)	26(89.7%)	24(85.7%)	23(88.5%)
朴树 Celtis sinensis	30	16	17	25	13	15	10(62.5%)	10(58.8%)	24(80.0%)	9(56.3%)
西南卫矛 Euonymus hamiltonianus	5	19	3	5	9	3	19(100.0%)	3(100.0%)	5(100.0%)	19(100.0%)
狭叶润楠 Machilus rehderi	38	24	34	19	15	13	11(45.8%)	21(61.8%)	25(65.8%)	11(45.8%)
杨梅叶蚊母树 Distylium myricoides	45	30	27	27	16	14	24(80.0%)	11(40.7%)	39(86.7%)	14(46.7%)
榆树 Ulmus pumila	14	7	7	10	7	6	5(71.4%)	6(85.7%)	12(85.7%)	6(85.7%)
小计 Sub-total	231	196	245	163	134	141	154(78.6%)	174(71.0%)	189(81.8%)	125(63.8%)

下载: 导出CSV

表 4 茂兰喀斯特森林木本植物幼苗密度半方差函数的模型类型及参数

Table 4 Fitted model types and parameters for semivariograms of woody plant seedling density from karst forest of Maolan

年份 Year	最优模型 Optimal model	块金值 Nugget (C₀)	基台值 Sill (C₀ + C)	空间结构比 Spatial structure ratio C₀/(C₀ + C)	变程 Range (A)/m	R²
2017	高斯模型 Gaussian model	3.200	33.630	0.095	14.896	0.654
2018	线性模型 Linear model	21.050	25.126	0.838	76.850	0.521
2019	线性模型 Linear model	63.482	84.584	0.751	76.850	0.863
注：C 表示拱高。Note: C means arch height.

下载: 导出CSV

参考文献(65)

[1]	Szwagrzyk J, Szewczyk J, Bodziarczyk J. Dynamics of seedling banks in beech forest: results of a 10-year study on germination, growth and survival[J]. Forest Ecology and Management, 2001, 141(3): 237−250. doi: 10.1016/S0378-1127(00)00332-7
[2]	尹华军, 程新颖, 赖挺, 等. 川西亚高山65年人工云杉林种子雨、种子库和幼苗定居研究[J]. 植物生态学报, 2011, 35(1): 35−44. doi: 10.3724/SP.J.1258.2011.00035 Yin H J, Cheng X Y, Lai T, et al. Seed rain, soil seed bank and seedling regeneration in a 65-year Picea asperata plantation in subalpine coniferous, western Sichuan, China[J]. Chinese Journal of Plant Ecology, 2011, 35(1): 35−44. doi: 10.3724/SP.J.1258.2011.00035
[3]	李小双, 彭明春, 党承林. 植物自然更新研究进展[J]. 生态学杂志, 2007, 26(12): 2081−2088. Li X S, Peng M C, Dang C L. Research progress on natural regeneration of plants[J]. Chinese Journal of Ecology, 2007, 26(12): 2081−2088.
[4]	李洁琼, 宋晓阳, 曹敏. 云南哀牢山和玉龙雪山森林树种幼苗对海拔梯度的响应及其季节性差异[J]. 应用生态学报, 2016, 27(11): 3403−3412. Li J Q, Song X Y, Cao M. Response of tree seedlings to altitudinal gradient and its seasonal variation in Ailao Mountain and Yulong Mountain, Yunnan Province, China[J]. Chinese Journal of Applied Ecology, 2016, 27(11): 3403−3412.
[5]	李晓亮, 王洪, 郑征, 等. 西双版纳热带森林树种幼苗的组成、空间分布和旱季存活[J]. 植物生态学报, 2009, 33(4): 658−671. Li X L, Wang H, Zheng Z, et al. Composition, spatial distribution and survival during the dry season of tree seedlings in a tropical forest in Xishuangbanna, SW China[J]. Chinese Journal of Plant Ecology, 2009, 33(4): 658−671.
[6]	张健, 李步杭, 白雪娇, 等. 长白山阔叶红松林乔木树种幼苗组成及其年际动态[J]. 生物多样性, 2009, 17(4): 385−396. doi: 10.3724/SP.J.1003.2009.09102 Zhang J, Li B H, Bai X J, et al. Composition and interannual dynamics of tree seedlings in broad-leaved Korean pine (Pinus koraiensis) mixed forest in Changbai Mountain[J]. Biodiversity Science, 2009, 17(4): 385−396. doi: 10.3724/SP.J.1003.2009.09102
[7]	刘帅. 长白山针阔混交林乔木幼苗动态研究 [D]. 北京: 北京林业大学, 2016. Liu S. Studies on the tree seedling dynamics of coniferous and broad leaved mixed forests in Changbai Mountain [D]. Beijing: Beijing Forestry University, 2016.
[8]	Comita L S, Aguilar S, Perez R, et al. Patterns of woody plant species abundance and diversity in the seedling layer of a tropical forest[J]. Journal of Vegetation Science, 2007, 18: 163−174. doi: 10.1658/1100-9233(2007)18[163:POWPSA]2.0.CO;2
[9]	李艳丽, 杨华, 亢新刚, 等. 长白山云冷杉针阔混交林天然更新空间分布格局及其异质性[J]. 应用生态学报, 2014, 25(2): 311−317. Li Y L, Yang H, Kang X G, et al. Spatial heterogeneity of natural regeneration in a spruce-fir mixed broad leaf-conifer forest in Changbai Mountains[J]. Chinese Journal of Applied Ecology, 2014, 25(2): 311−317.
[10]	李雪云, 潘萍, 欧阳勋志, 等. 闽楠天然次生林幼树幼苗更新特征及空间分布格局[J]. 东北林业大学学报, 2018, 46(9): 11−15. doi: 10.3969/j.issn.1000-5382.2018.09.003 Li X Y, Pan P, Ouyang X Z, et al. Spatial distribution patterns and regeneration characteristics of seedling and sapling in natural secondary Phoebe bournei forest[J]. Journal of Northeast Forestry University, 2018, 46(9): 11−15. doi: 10.3969/j.issn.1000-5382.2018.09.003
[11]	Albrecht M A, McCarthy B C. Seedling establishment shapes the distribution of shade-adapted forest herbs across a topographical moisture gradient[J]. Journal of Ecology, 2009, 97(5): 1037−1049. doi: 10.1111/j.1365-2745.2009.01527.x
[12]	Oshima C, Tokumoto Y, Nakagawa M. Biotic and abiotic drivers of dipterocarp seedling survival following mast fruiting in Malaysian Borneo[J]. Journal of Tropical Ecology, 2014, 31(2): 23−24.
[13]	Dai N, Kenji S, Akiko S. Seedling establishment of deciduous trees in various topographic positions[J]. Journal of Vegetation Science, 2002, 13(1): 11−14.
[14]	Tang C Q, Qhsawa M. Coexistence mechanisms of evergreen, deciduous and coniferous trees in a mid-montane mixed forest on Mt. Emei, Sichuan, China[J]. Plant Ecology, 2002, 161: 215−230. doi: 10.1023/A:1020395830795
[15]	Cantón Y, Barrio G D, Solé-Benet A, et al. Topographic controls on the spatial distribution of ground cover in the Tabernas badlands of SE Spain[J]. Catena, 2004, 55(3): 341−365. doi: 10.1016/S0341-8162(03)00108-5
[16]	彭闪江, 黄忠良, 彭少麟, 等. 植物天然更新过程中种子和幼苗死亡的影响因素[J]. 广西植物, 2004, 24(2): 113−121, 124. doi: 10.3969/j.issn.1000-3142.2004.02.004 Peng S J, Huang Z L, Peng S L, et al. Factors influencing mortality of seed and seedling in plant nature regeneration process[J]. Guihaia, 2004, 24(2): 113−121, 124. doi: 10.3969/j.issn.1000-3142.2004.02.004
[17]	Wright S J, Muller-Landau H C, Calderón O, et al. Annual and spatial variation in seedfall and seedling recruitment in a neotropical forest[J]. Ecology, 2005, 86(4): 848−860. doi: 10.1890/03-0750
[18]	郭秋菊. 择伐和火干扰对长叶松幼苗更新的影响 [D]. 杨凌: 西北农林科技大学, 2013. Guo Q J. Effects of selection and prescribed firedisturbances on longleaf pine seedling regeneration [D]. Yangling: Northwest A&F University, 2013.
[19]	Connell J H, Green P T. Seedling dynamics over thirty-two years in a tropical rain forest tree[J]. Ecology, 2000, 81(2): 568−584. doi: 10.1890/0012-9658(2000)081[0568:SDOTTY]2.0.CO;2
[20]	Norden N, Chave J, Caubère A, et al. Is temporal variation of seedling communities determined by environment or by seed arrival? A test in a neotropical forest[J]. Journal of Ecology, 2007, 95(3): 507−516. doi: 10.1111/j.1365-2745.2007.01221.x
[21]	Metz M R, Comita L S, Chen Y Y, et al. Temporal and spatial variability in seedling dynamics: a cross-site comparison in four lowland tropical forests[J]. Journal of Tropical Ecology, 2008, 24(1): 9−18. doi: 10.1017/S0266467407004695
[22]	龙翠玲. 茂兰喀斯特森林林隙大小对树种更新的影响[J]. 南京林业大学学报 (自然科学版), 2008, 32(2): 34−38. Long C L. Effects of gap size on regeneration of karst forest in Maolan Natural Reserve of Guizhou Province[J]. Journal of Nanjing Forestry University (Natural Sciences Edition), 2008, 32(2): 34−38.
[23]	朱守谦. 喀斯特森林生态研究 [M]. 贵阳: 贵州科技出版社, 2003. Zhu S Q. Ecological researchon karst forest [M]. Guiyang: Guizhou Science and Technology Publishing House, 2003.
[24]	符裕红, 彭琴, 李安定, 等. 喀斯特石灰岩产状地下生境的土壤质量[J]. 森林与环境学报, 2017, 37(3): 353−359. Fu Y H, Peng Q, Li A D, et al. Soil quality of the limestone underground habitat types in karst areas[J]. Journal of Forest and Environment, 2017, 37(3): 353−359.
[25]	龙健, 李娟, 江新荣, 等. 贵州茂兰喀斯特森林土壤微生物活性的研究[J]. 土壤学报, 2004, 41(4): 597−602. doi: 10.3321/j.issn:0564-3929.2004.04.016 Long J, Li J, Jang X R, et al. Soil microbial activities in Maolan karst forest, Guizhou Province[J]. Acta Pedologica Sinica, 2004, 41(4): 597−602. doi: 10.3321/j.issn:0564-3929.2004.04.016
[26]	宋新章, 张智婷, 张慧玲, 等. 长白山森林不同演替阶段采伐林隙幼苗更新特征[J]. 江西农业大学学报, 2010, 32(3): 504−509, 516. doi: 10.3969/j.issn.1000-2286.2010.03.017 Song X Z, Zhang Z T, Zhang H L, et al. Characteristics of seedlings in logging gaps of forests at different succession stages in Changbai Mountain[J]. Acta Agriculturae Universitatis Jiangxiensis, 2010, 32(3): 504−509, 516. doi: 10.3969/j.issn.1000-2286.2010.03.017
[27]	尤冬梅. 环境因子对水杉种子萌发与幼苗生长的影响研究 [D]. 武汉: 华中师范大学, 2008. You D M. The effects of environmental factors on seedgermination and seedling growth of Metasequoia glyptostroboides [D]. Wuhan: Central China Normal University, 2008.
[28]	喻理飞, 朱守谦, 祝小科, 等. 退化喀斯特森林恢复评价和修复技术[J]. 贵州科学, 2002, 20(1): 7−13. doi: 10.3969/j.issn.1003-6563.2002.01.002 Yu L F, Zhu S Q, Zhu X K, et al. A study on evaluation of restoration and remedy technology of degraded karst forest[J]. Guizhou Science, 2002, 20(1): 7−13. doi: 10.3969/j.issn.1003-6563.2002.01.002
[29]	刘济明. 茂兰喀斯特森林中华蚊母树群落土壤种子库动态初探[J]. 植物生态学报, 2000, 24(3): 366−374. doi: 10.3321/j.issn:1005-264X.2000.03.021 Liu J M. A preliminary study on the soil seed bank dynamics of the Distylium chinensis community in the Maolan karst forest[J]. Chinese Journal of Plant Ecology, 2000, 24(3): 366−374. doi: 10.3321/j.issn:1005-264X.2000.03.021
[30]	玉屏, 兰洪波, 冉景丞, 等. 茂兰自然保护区生物多样性现状及保护对策[J]. 现代农业科技, 2011(15): 233−234, 236. doi: 10.3969/j.issn.1007-5739.2011.15.142 Yu P, Lan H B, Ran J C, et al. Status and conservation countermeasures of biodiversity in Maolan National Reserve[J]. Modern Agricultural Science and Technology, 2011(15): 233−234, 236. doi: 10.3969/j.issn.1007-5739.2011.15.142
[31]	龙翠玲, 余世孝, 熊志斌, 等. 茂兰喀斯特森林林隙的植物多样性与更新[J]. 生物多样性, 2005, 13(1): 43−50. doi: 10.3321/j.issn:1005-0094.2005.01.006 Long C L, Yu S X, Xiong Z B, et al. Species diversity and regeneration in forest gaps of the karst forest in Maolan National Nature Reserve, Guizhou Province[J]. Biodiversity Science, 2005, 13(1): 43−50. doi: 10.3321/j.issn:1005-0094.2005.01.006
[32]	孟令君, 姚杰, 秦江环, 等. 吉林蛟河针阔混交林乔木幼苗组成及其密度格局影响因素[J]. 植物生态学报, 2018, 42(6): 653−662. doi: 10.17521/cjpe.2018.0025 Meng L J, Yao J, Qin J H, et al. Drivers of composition and density pattern of tree seedlings in a secondary mixed conifer and broad-leaved forest, Jiaohe, Jilin, China[J]. Chinese Journal of Plant Ecology, 2018, 42(6): 653−662. doi: 10.17521/cjpe.2018.0025
[33]	王政权, 王庆成, 李哈滨. 红松老龄林主要树种的空间异质性特征与比较的定量研究[J]. 植物生态学报, 2000, 24(6): 718−723. doi: 10.3321/j.issn:1005-264X.2000.06.013 Wang Z Q, Wang Q C, Li H B. Characteristics and comparison of spatial heterogeneity of the main species of Korean pine in old growth forests[J]. Chinese Journal of Plant Ecology, 2000, 24(6): 718−723. doi: 10.3321/j.issn:1005-264X.2000.06.013
[34]	刘志理, 毕连柱, 宋国华, 等. 典型阔叶红松林叶面积指数的空间异质性[J]. 北京林业大学学报, 2018, 40(11): 1−11. Liu Z L, Bi L Z, Song G H, et al. Spatial heterogeneity of leaf area index in a typical mixed broadleaved-Korean pine forest in Xiaoxing’an Mountains of northeastern China[J]. Journal of Beijing Forestry University, 2018, 40(11): 1−11.
[35]	刘检明. 林冠结构对林下幼苗多样性影响的研究: 以八大公山亚热带山地常绿落叶阔叶混交林为例 [D]. 武汉: 中国科学院大学 (中国科学院武汉植物园), 2018. Liu J M. Study on relationships between canopy structure and seedling diversity: a case study in the dynamic plot of subtropical evergreen and deciduous broadleaved mixed forest in Badagongshan [D]. Wuhan: Wuhan Botanical Garden, Chinese Academy of Sciences, 2018.
[36]	姚杰, 闫琰, 张春雨, 等. 吉林蛟河针阔混交林乔木幼苗组成与月际动态[J]. 植物生态学报, 2015, 39(7): 717−725. doi: 10.17521/cjpe.2015.0068 Yao J, Yan Y, Zhang C Y, et al. Composition and monthly dynamics of tree seedlings in a coniferous and broad-leaved mixed forest in Jiaohe, Jilin Province, China[J]. Chinese Journal of Plant Ecology, 2015, 39(7): 717−725. doi: 10.17521/cjpe.2015.0068
[37]	邱华, 舒皓, 吴兆飞, 等. 长白山阔叶红松林乔木幼苗组成及多度格局的影响因素[J]. 生态学报, 2020, 40(6): 2049−2056. Qiu H, Shu H, Wu Z F, et al. Influencing factors of composition and abundance pattern of tree seedlings in broadleaved Korean pine (Pinus koraiensis) mixed forest, Changbai Mountain, China[J]. Acta Ecologica Sinica, 2020, 40(6): 2049−2056.
[38]	宋慧清, 倪鸣源, 朱师丹. 乔木与木质藤本的水力与光合性状的差异: 以热带森林崖豆藤属和买麻藤属为例[J]. 植物生态学报, 2020, 44(3): 192−204. doi: 10.17521/cjpe.2019.0304 Song H Q, Ni M Y, Zhu S D. Hydraulic and photosynthetic characteristics differ between co-generic tree and liana species: a case study of Millettia and Gnetum in tropical forest[J]. Chinese Journal of Plant Ecology, 2020, 44(3): 192−204. doi: 10.17521/cjpe.2019.0304
[39]	郝建辉. 海南霸王岭木质藤本对低地次生林群落更新的影响 [D]. 重庆: 西南大学, 2010. Hao J H. The effects of lianas on the regeneration of lowland secondary forest in nature reserve, Hainan Island [D]. Chongqing: Southwest University, 2010.
[40]	郝建辉, 陶建平, 赵科, 等. 木质藤本对人工林群落结构及更新的影响[J]. 西南大学学报 (自然科学版), 2011, 33(2): 34−39. Hao J H, Tao J P, Zhao K, et al. The effects of lianas on community structure and regeneration of vatica astrotricha plantations in Bawangling Nature Reserve, Hainan Province, China[J]. Journal of Southwest University (Natural Science Edition), 2011, 33(2): 34−39.
[41]	巩合德, 杨国平, 鲁志云, 等. 哀牢山常绿阔叶林乔木树种的幼苗组成及时空分布特征[J]. 生物多样性, 2011, 19(2): 151−157. doi: 10.3724/SP.J.1003.2011.07010 Gong H D, Yang G P, Lu Z Y, et al. Composition and spatio-temporal distribution of tree seedlings in an evergreen broad-leaved forest in the Ailao Mountains, Yunnan[J]. Biodiversity Science, 2011, 19(2): 151−157. doi: 10.3724/SP.J.1003.2011.07010
[42]	Changyang C H, Lu C L, Sun I F, et al. Long-term seedling dynamics of tree species in a subtropical rain forest, Taiwan[J]. Taiwania, 2013, 58(1): 35−43.
[43]	刘帅, 肖翠, 王均伟, 等. 长白山阔叶红松林乔木幼苗年际动态及影响因素[J]. 北京林业大学学报, 2016, 38(11): 57−66. Liu S, Xiao C, Wang J W, et al. Interannual seedling dynamic and influencing factors on seedling survival of tree species in a broadleaved Korean pine (Pinus koraiensis) mixed forest in Changbai Mountains, northeastern China[J]. Journal of Beijing Forestry University, 2016, 38(11): 57−66.
[44]	Nathan R, Safriel U N, Noy-Meir S, et al. Spatiotemporal variation in seed dispersal and recruitment near and far from Pinus halepensis trees[J]. Ecology, 2000, 81(8): 2156−2169. doi: 10.1890/0012-9658(2000)081[2156:SVISDA]2.0.CO;2
[45]	李华. 种子萌发及幼苗形态的研究[J]. 种子, 1992(3): 46−48. Li H. Study on seed germination and seedling morphology[J]. Seed, 1992(3): 46−48.
[46]	刘有军, 刘世增, 纪永福, 等. 碟果虫实种子萌发对策及生态适应性[J]. 生态学报, 2010, 30(24): 6910−6918. Liu Y J, Liu S Z, Ji Y F, et al. Seed germinative strategy and ecological adaptability of Corisperimum patelliforme[J]. Acta Ecologica Sinica, 2010, 30(24): 6910−6918.
[47]	万海霞, 贾宝光, 余家治, 等. 栓翅卫矛种子萌发条件的初步研究[J]. 中国种业, 2018(2): 62−64. doi: 10.3969/j.issn.1671-895X.2018.02.020 Wan H X, Jia B G, Yu J Z, et al. Preliminary study on seed germination conditions of Euonymus amurensis[J]. China Seed Industry, 2018(2): 62−64. doi: 10.3969/j.issn.1671-895X.2018.02.020
[48]	孙海群, 冀建鹏. 低温冻害对萌动的霸王种子发芽特性的影响[J]. 黑龙江畜牧兽医, 2013(7): 76−77. Sun H Q, Ji J P. Effect of low temperature injury on germinating seed germination characteristics of Sarcozygium xanthoxylon Bunge seed[J]. Heilongjiang Animal Science and Veterinary Medicine, 2013(7): 76−77.
[49]	闫琰, 张新娜, 姚杰, 等. 吉林蛟河不同演替阶段针阔混交林乔木幼苗数量组成及其时间动态[J]. 植物生态学报, 2016, 40(2): 127−139. doi: 10.17521/cjpe.2015.0365 Yan Y, Zhang X N, Yao J, et al. Composition and temporal dynamics of tree seedlings at different successional stages of conifer and broad-leaved mixed forests in Jiaohe, Jilin Province, China[J]. Chinese Journal of Plant Ecology, 2016, 40(2): 127−139. doi: 10.17521/cjpe.2015.0365
[50]	谭一波, 詹潮安, 肖泽鑫, 等. 广东南澳岛中华楠种群结构及动态特征[J]. 生态学杂志, 2010, 29(10): 1901−1906. Tan Y B, Zhan C A, Xiao Z X, et al. Population structure and dynamic characteristics of Machilus chinensis in Nan’ao Island, Guangdong Province[J]. Chinese Journal of Ecology, 2010, 29(10): 1901−1906.
[51]	Comita L S, Hubbell S P. Local neighborhood and species’ shade tolerance influence survival in a diverse seedling bank[J]. Ecology, 2009, 90(2): 328−334. doi: 10.1890/08-0451.1
[52]	Harms K E, Condit R, Hubbell S P, et al. Habitat associations of trees and shrubs in a 50-ha neotropical forest plot [J]. 2001, 89(6): 947−959.
[53]	Martínez I, Taboada F G, Wiegand T, et al. Spatial patterns of seedling-adult associations in a temperate forest community[J]. Forest Ecology and Management, 2013, 296: 74−80. doi: 10.1016/j.foreco.2013.02.005
[54]	宾粤, 叶万辉, 曹洪麟, 等. 鼎湖山南亚热带常绿阔叶林20公顷样地幼苗的分布[J]. 生物多样性, 2011, 19(2): 127−133. doi: 10.3724/SP.J.1003.2011.07290 Bin Y, Ye W H, Cao H L, et al. Seedling distribution in a subtropical evergreen broad-leaved forest plot in the Dinghu Mountain[J]. Biodiversity Science, 2011, 19(2): 127−133. doi: 10.3724/SP.J.1003.2011.07290
[55]	张忠华, 胡刚, 祝介东, 等. 喀斯特森林土壤养分的空间异质性及其对树种分布的影响[J]. 植物生态学报, 2011, 35(10): 1038−1049. Zhang Z H, Hu G, Zhu J D, et al. Spatial heterogeneity of soil nutrients and its impact on tree species distribution in a karst forest of Southwest China[J]. Chinese Journal of Plant Ecology, 2011, 35(10): 1038−1049.
[56]	黄萍, 刘艳红. 北京松山油松林林分结构和地形对幼苗更新的影响[J]. 生态学杂志, 2018, 37(4): 1003−1009. Huang P, Liu Y H. Effects of stand structure and terrain factors on seedling regeneration of Pinus tabuliformis forest in the Songshan National Nature Reserve, Beijing[J]. Chinese Journal of Ecology, 2018, 37(4): 1003−1009.
[57]	张忠华, 胡刚, 倪健, 等. 茂兰喀斯特常绿落叶阔叶混交林树种的空间分布格局及其分形特征[J]. 生态学报, 2015, 35(24): 8221−8230. Zhang Z H, Hu G, Ni J, et al. Spatial distribution patterns and their fractal properties for trees in a subtropical mixed evergreen-deciduous broad-leaved karst forest in Maolan, southwestern China[J]. Acta Ecologica Sinica, 2015, 35(24): 8221−8230.
[58]	黄云霞, 徐萱, 张莉芗, 等. 百山祖常绿阔叶林灌草层物种组成和分布的10年动态[J]. 生物多样性, 2016, 24(12): 1353−1363. doi: 10.17520/biods.2016095 Huang Y X, Xu X, Zhang L X, et al. Ten-years period of grass and small woody plant dynamics in a 5-ha evergreen forest plot in Baishanzu, Zhejiang Province[J]. Biodiversity Science, 2016, 24(12): 1353−1363. doi: 10.17520/biods.2016095
[59]	殷正, 范秀华. 长白山不同演替阶段温带森林林下草本植物对乔木幼苗的影响[J]. 生态学报, 2020, 40(7): 2194−2204. Yin Z, Fan X H. Effects of herbs on tree seedlings in different succession stages of temperate forests in Changbai Mountain, China[J]. Acta Ecologica Sinica, 2020, 40(7): 2194−2204.
[60]	符裕红, 黄宗胜, 喻理飞, 等. 岩溶区典型根系地下生境的土壤质量分析[J]. 水土保持研究, 2012, 19(3): 67−73. Fu Y H, Huang Z S, Yu L F, et al. Analysis on the soil quality of different typical underground root habitat types in karst areas[J]. Research of Soil and Water Conservation, 2012, 19(3): 67−73.
[61]	陈欣, 韩有志. 关帝山不同密度云杉林的更新特征[J]. 山西农业科学, 2019, 47(8): 1446−1449. doi: 10.3969/j.issn.1002-2481.2019.08.31 Chen X, Han Y Z. Regeneration characteristics of spruce forests with different densities in Guandi Mountain[J]. Journal of Shanxi Agricultural Sciences, 2019, 47(8): 1446−1449. doi: 10.3969/j.issn.1002-2481.2019.08.31
[62]	于金莹, 亢新刚, 刘怀东. 林隙及其对天然林更新的影响[J]. 林业与生态科学, 2005, 20(2): 133−137. Yu J Y, Kang X G, Liu H D. Study advances on the forest gap and its influence on natural forest regeneration[J]. Forestry and Ecological Sciences, 2005, 20(2): 133−137.
[63]	姜玲玲, 许中旗. 东北东部山地蒙古栎林的年龄结构及天然更新研究[J]. 林业与生态科学, 2020, 35(4): 365−368, 376. Jiang L L, Xu Z Q. Research on age structure and natural regeneration of Quercus mongolica forest on eastern mountains in northeast region[J]. Forestry and Ecological Sciences, 2020, 35(4): 365−368, 376.
[64]	陈香茗. 长白山阔叶红松林种子雨及幼苗时空动态研究 [D]. 北京: 北京林业大学, 2011. Chen X M. Spatiotemporal dynamics of seed rain and seedling in broad-leaved Korean pine mixed forests in Changbai Mountain [D]. Beijing: Beijing Forestry University, 2011.
[65]	白志强, 刘华, 张新平, 等. 新疆额尔齐斯河流域杨树幼苗天然更新影响因子分析[J]. 西北林学院学报, 2011, 26(1): 98−102. Bai Z Q, Liu H, Zhang X P, et al. Analysis on influence factors of natural regeneration of young poplar seedlings in Irtysh River Basin, Xinjiang[J]. Journal of Northwest Forestry University, 2011, 26(1): 98−102.

施引文献(26)

期刊类型引用(13)

1.	陈仕媛，马岚，陈佩岩. 北方土石山区不同粒径团聚体特征及其对坡面侵蚀过程的影响. 水土保持研究. 2025(02): 102-110+139 . 百度学术
2.	张紫薇，赵文俊，李奇，马一淳，田亮，杨广勇，李忠武，刘窑军. 表层土壤结构对红壤坡耕地产流及产沙过程的影响. 土壤学报. 2024(02): 434-444 . 百度学术
3.	王琳涛，王云琦，王玉杰，张建聪，尹乐，张洪珊 . 火干扰对缙云山土壤团聚体稳定性的影响. 中国水土保持科学(中英文). 2023(03): 25-33 . 百度学术
4.	孙旭，马岚，张金阁，王福星，薛梦华，宋天翼，张旖璇，李浩玉. 模拟降雨条件下枯落物覆盖对坡面地表和壤中流产流过程的影响. 陕西师范大学学报(自然科学版). 2022(04): 136-144 . 百度学术
5.	刘文景，许文年，夏露，陈剑刚，程虎，余飞，夏栋，刘大翔，余绍鹏. 鄂西长江小流域土地利用方式对土壤团聚体稳定性的影响. 长江流域资源与环境. 2021(04): 925-935 . 百度学术
6.	郭岩松，毕曼. 土壤侵蚀影响因素研究进展. 河南科技. 2021(20): 136-140 . 百度学术
7.	黎俊佑，马岚，刘京晶，沈雨珺，张金阁，孙占薇，燕琳，张栋. 雨型对华北土石山区坡面土壤侵蚀的影响. 水土保持学报. 2020(04): 1-6 . 百度学术
8.	胡昭，张懿，郭建. 黄土丘陵区典型林地土壤团聚体稳定性评价. 人民黄河. 2020(08): 104-108 . 百度学术
9.	郭威震，胡斐南，谭滔滔，马任甜，刘婧芳，李喆，赵世伟. 土壤表面电场对黑土团聚体破碎和侵蚀的影响. 应用生态学报. 2020(08): 2644-2652 . 百度学术
10.	张金阁，马岚，张栋，刘京晶，黎俊佑，孙占薇，燕琳，孙旭，王福星. 华北土石山区草地过滤带布设方式对坡面产流产沙的影响. 水土保持学报. 2020(05): 76-80 . 百度学术
11.	毛旭芮，王明力，杨建军，丁肇龙，曹月娥. 采煤对露天煤矿土壤理化性质及可蚀性影响. 西南农业学报. 2020(11): 2537-2544 . 百度学术
12.	李肖，陈晨，林杰，朱茜，董波，丁鸣鸣. 侵蚀强度对淮北土石山区土壤团聚体组成及稳定性的影响. 水土保持研究. 2019(04): 56-61+67 . 百度学术
13.	王婷，李建平，张翼，井乐，张茹. 不同降水下天然草地土壤水稳定性团聚体分布特征. 草业科学. 2019(08): 1935-1943 . 百度学术

其他类型引用(13)

资源附件(0)

图(4) / 表(4)

计量

文章访问数: 849
HTML全文浏览量: 221
PDF下载量: 71
被引次数: 26

1. 研究区概况与研究方法
1.1 研究区概况
1.2 数据来源与统计
1.3 研究方法
1.3.1 基础模型与广义模型
1.3.2 分位数回归
1.3.3 非线性混合效应模型
1.3.4 模型评价与检验
2. 结果与分析
2.1 基础模型拟合
2.2 广义模型构建
2.3 分位数回归
2.4 非线性混合效应模型构建
2.5 模型评价与检验
3. 讨　　论
3.1 基于基础模型的分位数回归
3.2 引入林分变量和随机效应对树高模型的影响
4. 结　　论

1. 研究区概况与研究方法
1.1 研究区概况
1.2 数据来源与统计
1.3 研究方法
1.3.1 基础模型与广义模型
1.3.2 分位数回归
1.3.3 非线性混合效应模型
1.3.4 模型评价与检验
2. 结果与分析
2.1 基础模型拟合
2.2 广义模型构建
2.3 分位数回归
2.4 非线性混合效应模型构建
2.5 模型评价与检验
3. 讨　　论
3.1 基于基础模型的分位数回归
3.2 引入林分变量和随机效应对树高模型的影响
4. 结　　论

参考文献(65)

施引文献(26)

资源附件(0)

喀斯特常绿落叶阔叶混交林木本植物幼苗组成及其年际动态

作者简介: 杨廷丽。主要研究方向：森林经理学。Email：yangtingligz@qq.com 地址：550025贵州省贵阳市花溪区贵州大学西校区崇德楼林学院

责任作者: 戚玉娇，副教授。主要研究方向：森林经理学和森林生态学研究。Email：yjqi@gzu.edu.cn 地址：同上

计量

出版历程

Seedling composition and its interannual dynamics of woody plants in karst evergreen deciduous broadleaved mixed forest

1. 研究区概况与研究方法

1.1 研究区概况

1.2 数据来源与统计

1.3 研究方法

1.3.1 基础模型与广义模型

1.3.2 分位数回归

1.3.3 非线性混合效应模型

1.3.4 模型评价与检验

2. 结果与分析

2.1 基础模型拟合

2.2 广义模型构建

2.3 分位数回归

2.4 非线性混合效应模型构建

2.5 模型评价与检验

3. 讨 论

3.1 基于基础模型的分位数回归

3.2 引入林分变量和随机效应对树高模型的影响

4. 结 论

期刊类型引用(13)

其他类型引用(13)

计量

出版历程

目录

1. 研究区概况与研究方法

1.1 研究区概况

1.2 数据来源与统计

1.3 研究方法

1.3.1 基础模型与广义模型

1.3.2 分位数回归

1.3.3 非线性混合效应模型

1.3.4 模型评价与检验

2. 结果与分析

2.1 基础模型拟合

2.2 广义模型构建

2.3 分位数回归

2.4 非线性混合效应模型构建

2.5 模型评价与检验

3. 讨 论

3.1 基于基础模型的分位数回归

3.2 引入林分变量和随机效应对树高模型的影响

4. 结 论

作者简介:
杨廷丽。主要研究方向：森林经理学。Email：yangtingligz@qq.com　地址：550025贵州省贵阳市花溪区贵州大学西校区崇德楼林学院

责任作者:
戚玉娇，副教授。主要研究方向：森林经理学和森林生态学研究。Email：yjqi@gzu.edu.cn　地址：同上

3. 讨　　论

4. 结　　论

3. 讨　　论

4. 结　　论