Modal sensitivity and vibration mode of full-size oriented strand board panel under three boundary conditions
-
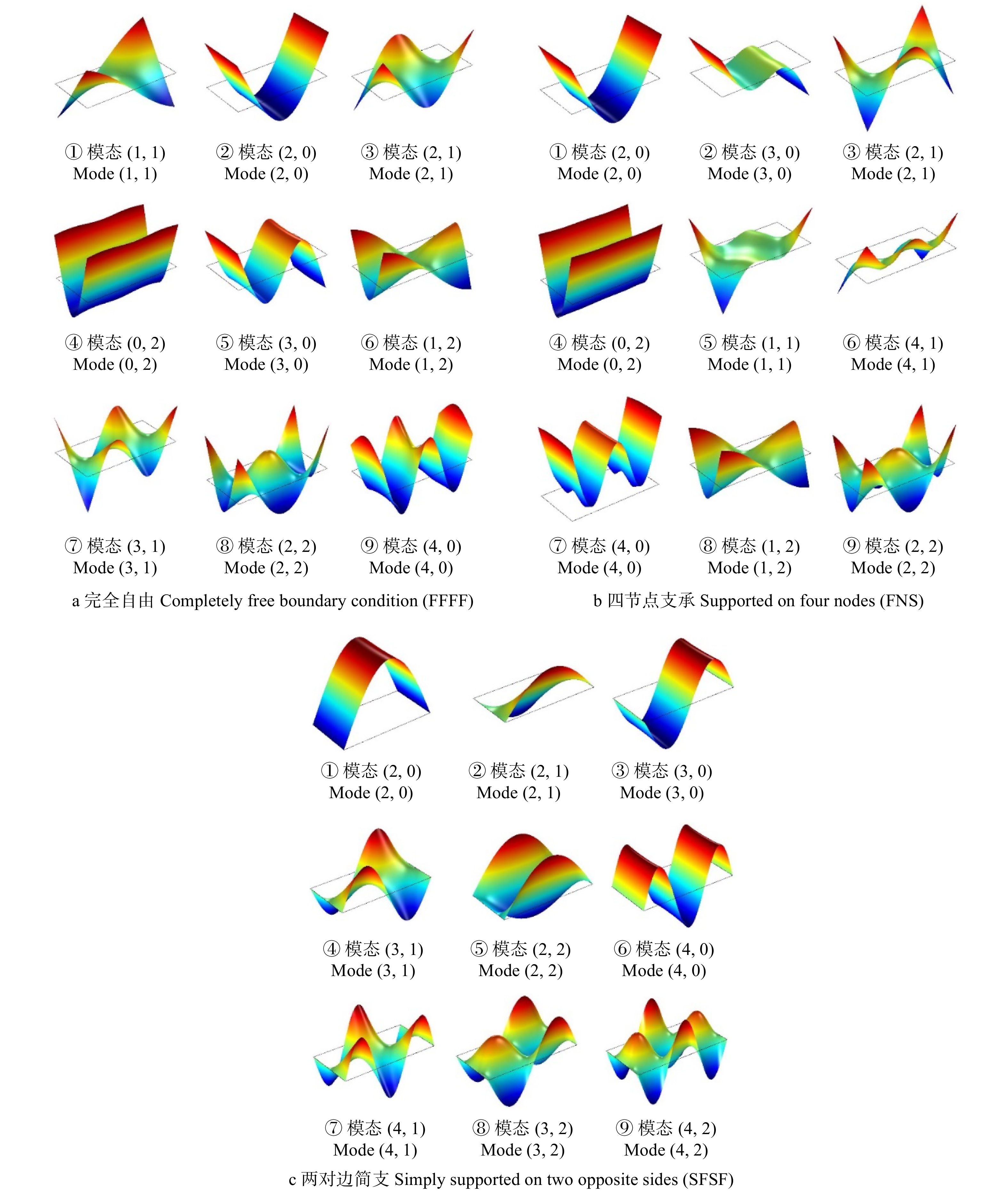
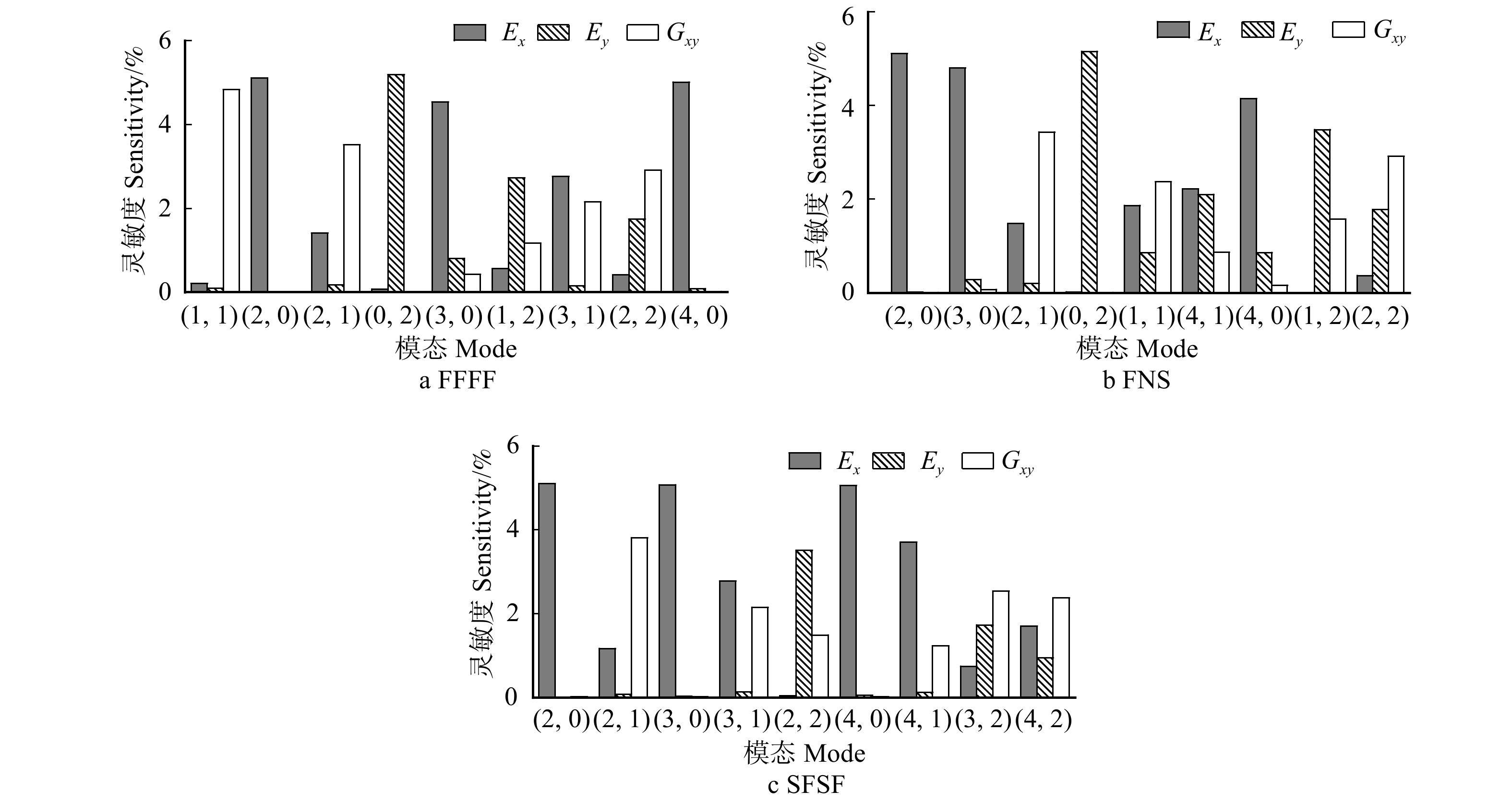
摘要:目的 研究完全自由、四节点支承和两对边简支3种边界条件下足尺定向刨花板的模态灵敏度和振动模态，为开展3种边界条件下足尺定向刨花板弹性常数振动检测结果的对比研究奠定基础。方法 以4种厚度的足尺定向刨花板为研究对象，采用有限元软件COMSOL Multiphysics对完全自由、四节点支承和两对边简支的足尺定向刨花板进行了模态灵敏度分析，分别确定这3种边界条件下对其长度和宽度方向的弹性模量与面内剪切模量这3个弹性常数灵敏度最高的模态；通过试验模态分析测得足尺定向刨花板在这3种边界条件下的前9阶振动模态参数，并对比和分析其在这3种边界条件下的振动模态参数检测结果。结果 计算和试验模态分析得到的这3种边界条件下足尺定向刨花板的前9阶模态振型形状和阶次分别是相同的；足尺定向刨花板在这3种边界条件下的前9阶模态中，除模态（m, 0）、（0, 2）和（1, 1）外，其余模态均为单一方向的弯曲和扭转或不同方向弯曲的叠加模态；用于计算足尺定向刨花板长度和宽度方向的弹性模量与面内剪切模量的最高灵敏度模态，在完全自由下为模态（2, 0）、（0, 2）和（1, 1），对应阶次分别为第2、4和1阶；在四节点支承下为模态（2, 0）、（0, 2）和（2, 1），对应阶次分别为第1、4和3阶；在两对边简支下为模态（2, 0）、（2, 2）和（2, 1），对应阶次分别为第1、5和2阶。结论 从振型角度说明基于计算模态分析方法和试验模态分析方法分别进行3种边界条件下足尺定向刨花板的模态灵敏度分析和振动模态测试具有可行性。Abstract:Objective To lay the foundation for the comparative study on vibration testing results of elastic constants for full-size oriented strand board (OSB) panel under these three boundary conditions, the modal sensitivity and vibration mode of full-size OSB panel under completely free boundary condition, supported on four nodes and simply supported on two opposite sides were studied, respectively.Method Full-size OSB panels with 4 kinds of thicknesses were used as study object. The modal sensitivity analysis of full-size OSB panels under these three boundary conditions was carried out using finite element software COMSOL Multiphysics, and the modes with the highest sensitivity corresponding to their three elastic constants including modulus of elasticity (Ex and Ey) in the length and width direction as well as in-plane shear modulus Gxy were obtained; the first nine vibration modal parameters of full-size OSB panels under these three boundary conditions were measured through experimental modal analysis, and the testing results of their vibration modal parameters under three boundary conditions were compared and analyzed.Result The modal shape and order of the first nine mode of full-size OSB panel under three boundary conditions were separately identical through experimental and theoretical modal analysis; except for mode (m, 0), (0, 2) and (1, 1), the first nine modes of full-size OSB panel under three boundary conditions were superimposed modes for bending and torsional in single direction or bending in different directions; the modes with the highest sensitivity for calculating Ex
, Ey and Gxy of full-size OSB panel were mode (2, 0), (0, 2) and (1, 1) corresponding to the second, fourth and the first order under completely free boundary condition, mode (2, 0), (0, 2) and (2, 1) corresponding to the first, fourth and the third order for being supported on four nodes, mode (2, 0), (2, 2) and (2, 1) corresponding to the first, fifth and the second order for being simply supported on two opposite sides. Conclusion From the perspective of vibration mode, it is feasible for performing the modal sensitivity analysis and vibration modal test of full-size OSB panel under three boundary conditions based on theoretical and experimental modal analysis method. -
-
![]()
图 1 四节点支承的足尺定向刨花板的网格划分图
Figure 1. Mesh division diagram of full-size OSB panel supported on four nodes
![]()
图 2 3种边界条件下的足尺定向刨花板前9阶计算模态振型图
Figure 2. First nine calculated mode shapes of full-size OSB panel under three boundary conditions
![]()
图 3 3种边界条件下足尺定向刨花板前9阶模态对Ex、Ey和Gxy的灵敏度分析结果
Figure 3. Results of sensitivity analysis of the first nine modes to Ex, Ey and Gxy of full-size OSB panel under three boundary conditions
![]()
图 4 3种边界条件下的足尺定向刨花板的试验模态分析示意图和试验照片
Figure 4. Schematic diagram and test photos of experimental modal analysis for full-size OSB panel under three boundary conditions
![]()
图 5 3种边界条件下的足尺定向刨花板的前9阶试验模态振型图
Figure 5. First nine experimental modal shapes of full-size OSB panel under three boundary conditions
![]()
图 6 3种边界条件下的足尺定向刨花板的前9阶试验模态频率
Figure 6. First nine experimental modal frequencies of full-size OSB panel under three boundary conditions
![]()
图 7 不同边界条件下足尺定向刨花板的共有模态频率间的相关性
Figure 7. Correlations between the common modal frequencies of full-size OSB panel under different boundary conditions
表 1 被测足尺定向刨花板的基本参数
Table 1 Basic parameters of full-size oriented strand board (OSB) panel tested
板材
Panel尺寸
Dimension平均密度
Average density/
（kg·m−3）平均含水率
Average moisture
content/%OSB13 2 444 mm × 1 222 mm ×
13.6 mm584 4.8 OSB15 2 444 mm × 1 222 mm ×
15.6 mm535 4.9 OSB18 2 444 mm × 1 220 mm ×
18.7 mm558 5.2 OSB20 2 444 mm × 1 221 mm ×
20.1 mm530 4.7 注：OSB13、OSB15、OSB18和OSB20分别表示标称厚度为13、15、18和20 mm的足尺定向刨花板。Notes: OSB13, OSB15, OSB18 and OSB20 represent the full-size OSB panels with nominal thickness of 13, 15, 18 and 20 mm, respectively. 
下载: 导出CSV
表 2 足尺定向刨花板模态灵敏度分析的初始参数
Table 2 Initial parameters for modal sensitivity analysis of full-size OSB panel
板材
Panel弹性模量
Modulus of elasticity/MPa剪切模量
Shear modulus/MPa泊松比
Poisson’s ratio
(υxy)密度
Density (ρ)/
(kg·m−3)尺寸
DimensionEx Ey Gxy Gyz Gxz OSB 5 700 1 990 980 770 750 0.23 587.0 2 444 mm × 1 222 mm × 13.0 mm 注：表中数据取自参考文献[6]、[10]和[18]。Ex和Ey分别为足尺定向刨花板长度和宽度方向的弹性模量，Gxy、Gyz和Gxz分别为足尺定向刨花板x-y、y-z和x-z平面内的剪切模量。下同。Notes: data in the table are cited from reference [6], [10] and [18]. Ex and Ey represent MOE in the length and width directions of full-size OSB panel, respectively. Gxy, Gyz and Gxz represent shear modulus in the x-y, y-z and x-z planes of full-size OSB panels, respectively. Same as below.
下载: 导出CSV
表 3 3种边界条件下的足尺定向刨花板的前9阶试验模态参数
Table 3 First nine experimental modal parameters of full-size OSB panel under three boundary conditions
阶次
OrderFFFF FNS SFSF 模态频率
Modal frequency/Hz振型
Vibration
mode模态频率
Modal frequency/Hz振型
Vibration
mode模态频率
Modal frequency/Hz振型
Vibration
modeOSB
13OSB
15OSB
18OSB
20OSB
13OSB
15OSB
18OSB
20OSB
13OSB
15OSB
18OSB
201 7.1 8.0 9.9 10.7 （1, 1） 7.5 8.5 10.8 11.7 （2, 0） 3.1 4.0 4.7 5.1 （2, 0） 2 7.4 8.4 10.7 11.7 （2, 0） 13.5 15.7 18.6 20.5 （3, 0） 7.4 9.3 10.6 11.6 （2, 1） 3 17.4 19.7 23.7 25.9 （2, 1） 17.3 19.6 23.7 25.9 （2, 1） 11.9 15.5 18.2 20.0 （3, 0） 4 20.2 21.7 25.2 27.0 （0, 2） 20.1 21.8 25.1 27.1 （0, 2） 17.0 23.0 26.5 29.4 （3, 1） 5 21.7 24.9 29.3 32.2 （3, 0） 20.5 22.9 26.0 27.9 （1, 1） 24.5 30.5 32.3 35.0 （2, 2） 6 25.4 27.5 32.9 35.6 （1, 2） 22.3 24.0 27.3 29.4 （4, 1） 26.5 33.4 37.3 45.6 （4, 0） 7 29.8 33.3 40.1 47.0 （3, 1） 22.7 24.3 27.8 29.9 （4, 0） 32.5 41.2 43.5 52.5 （4, 1） 8 36.2 41.4 48.8 54.5 （2, 2） 25.6 27.6 33.0 35.4 （1, 2） 40.0 45.0 47.7 59.2 （3, 2） 9 44.8 49.8 62.6 68.9 （4, 0） 36.4 41.5 48.9 54.5 （2, 2） 46.6 62.2 68.0 75.3 （4, 2）
下载: 导出CSV
表 4 用于计算足尺定向刨花板弹性常数的频率所对应模态的阶次
Table 4 Order of the modes corresponding to the frequencies for calculating elastic constants of full-size OSB panel
边界条件
Boundary conditionEx Ey Gxy 模态
Mode阶次
Order模态
Mode阶次
Order模态
Mode阶次
OrderFFFF （2, 0） 2 （0, 2） 4 （1, 1） 1 FNS （2, 0） 1 （0, 2） 4 （2, 1） 3 SFSF （2, 0） 1 （2, 2） 5 （2, 1） 2
下载: 导出CSV
-
[1] 肖再然, 申伟, 刘振东. 中国定向刨花板市场[J]. 国际木业, 2020, 50(3):41−43. doi: 10.3969/j.issn.1671-4911.2020.03.010 Xiao Z R, Shen W, Liu Z D. Wood-based panels-oriented strand board (OSB) market of China[J]. International Wood Industry, 2020, 50(3): 41−43. doi: 10.3969/j.issn.1671-4911.2020.03.010
[2] 王正, 付海燕, 丁叶蔚, 等. 定向刨花板剪切模量和弹性模量动态测试[J]. 林业科学, 2019, 55(8):136−146. doi: 10.11707/j.1001-7488.20190815 Wang Z, Fu H Y, Ding Y W, et al. Dynamic testing of shear modulus and elastic modulus of oriented strand board[J]. Scientia Silvae Sinicae, 2019, 55(8): 136−146. doi: 10.11707/j.1001-7488.20190815
[3] 全国人造板标准化技术委员会. 定向刨花板: LY/T 1580—2010 [S]. 北京: 国家林业局, 2010. National Technical Committee on Wood-Based Panels Standardization of China. Oriented strand board: LY/T 1580−2010[S]. Beijing: State Forestry Administration, 2010.
[4] Larsson D. Using modal analysis for estimation of anisotropic material constants[J]. Journal of Engineering Mechanics, 1997, 123(3): 222−229. doi: 10.1061/(ASCE)0733-9399(1997)123:3(222)
[5] Zhou J H, Chui Y H, Gong M, et al. Comparative study on measurement of elastic constants of wood-based panels using modal testing: choice of boundary conditions and calculation methods[J]. Journal of Wood Science, 2017, 63(5): 1−16.
[6] Zhou J H, Chui Y H, Gong M, et al. Simultaneous measurement of elastic constants of full-size engineered wood panels by modal testing[J]. Holzforschung, 2016, 70(7): 673−682. doi: 10.1515/hf-2015-0117
[7] 李焕. 足尺人造板弹性常数的振动检测研究[D]. 北京: 北京林业大学, 2021. Li H. Study on determining elastic constants of full-size wood composite panels using vibration methods [D]. Beijing: Beijing Forestry University, 2021.
[8] 周卢婧. 基于双节线简支振动原理的足尺人造板力学性能检测方法研究[D]. 北京: 北京林业大学, 2018. Zhou L J. Nodal line simply supported vibration method for evaluating the mechanical properties of full-size wood composite panels [D]. Beijing: Beijing Forestry University, 2018.
[9] 管成, 刘晋浩, 张厚江, 等. 足尺人造板力学性能无损检测研究进展[J]. 北京林业大学学报, 2019, 41(9):164−172. Guan C, Liu J H, Zhang H J, et al. Literature review of mechanical properties of full-size wood composite panels using nondestructive testing technique[J]. Journal of Beijing Forestry University, 2019, 41(9): 164−172.
[10] 管成. 面向力学性能评估的足尺人造板四节点支承振动检测研究[D]. 北京: 北京林业大学, 2018. Guan C. Evaluation of mechanical properties of full-size wood composite panels supported on four nodes using vibration methods [D]. Beijing: Beijing Forestry University, 2018.
[11] 全国人造板标准化技术委员会. 人造板及饰面人造板理化性能试验方法: GB/T 17657—2013[S]. 北京: 中国标准出版社, 2014. National Technical Committee on Wood-Based Panels Standardization of China. Test methods of evaluating the properties of wood-based panels and surfaces decorated wood-based panels: GB/T 17657—2013[S]. Beijing: Standards Press of China, 2014.
[12] 李焕, 管成, 张厚江, 等. 足尺胶合板弹性模量的两对边简支振动检测研究[J]. 北京林业大学学报, 2021, 43(2):138−149. Li H, Guan C, Zhang H J, et al. Determining modulus of elasticity of full-size plywood panel simply supported on two opposite sides using a vibration method[J]. Journal of Beijing Forestry University, 2021, 43(2): 138−149.
[13] Ayorinde E O. On the sensitivity of derived elastic constants to the utilized modes in the vibration testing of composite plates[J]. Journal of Applied Mechanics, 1995, 211: 55−64.
[14] 曹树谦, 张文德. 振动结构模态分析—理论实验与应用[M]. 天津: 天津大学出版社, 2001. Cao S Q, Zhang W D. Modal analysis of vibrating structures: theoretical experiments and applications [M]. Tianjin: Tianjin University Press, 2001.
[15] 丁源. ABAQUS6.14中文版有限元分析从入门到精通[M]. 北京: 清华大学出版社, 2016. Ding Y. ABAQUS6.14 Chinese version of finite element analysis from entry to master [M]. Beijing: Tsinghua University Press, 2016.
[16] Ewins D J. Modal testing: theory and practice[M]. Letchworth: Research Studies Press Limited, 1986.
[17] Guan C, Zhang H J, Wang X P, et al. Experimental and theoretical modal analysis of full-sized wood composite panels supported on four nodes[J]. Materials, 2017, 10(6): 683. doi: 10.3390/ma10060683
[18] Thomas W H. Poisson’s ratios of an oriented strand board[J]. Wood Science and Technology, 2003, 37(3−4): 259−268. doi: 10.1007/s00226-003-0171-y
-
期刊类型引用(16)
1. 钟思琪，宁金魁，黄锦程，陈鼎泸，欧阳勋志，臧颢. 基于混合效应的杉木人工林冠幅模型. 森林与环境学报. 2024(02): 127-135 . 
百度学术
2. 段平，王云川，晋秋梅，李佳. 基于无人机可见光影像的单木胸径估算方法. 测绘与空间地理信息. 2023(01): 14-17 . 百度学术
3. 魏智海，魏姿芃. 基于单木分割及点云特征提取的单木胸径估测. 陕西林业科技. 2023(02): 18-23 . 百度学术
4. 王杰芬，夏磊，林露花，胡璐璐，徐怀兴，王聚中，徐小军. 结合放射线法和无人机影像提取冠幅估算杉木碳储量研究. 浙江林业科技. 2023(05): 42-50 . 百度学术
5. 夏洪涛，郭晓斌，张珍，田相林，郭福涛，孙帅超. 基于不同立地质量评价指标的杉木大径材林分树高-胸径模型. 中南林业科技大学学报. 2023(10): 80-88 . 百度学术
6. 肖德卿，罗芊芊，范辉华，邱群，周志春. 栽植模式对木荷幼林生长和形质性状家系变异影响. 林业科学. 2022(05): 85-92 . 百度学术
7. 王志波，季蒙，李永乐，李银祥，马世明，张海东. 华北落叶松人工林差分地位指数模型构建. 林业资源管理. 2021(01): 156-163 . 百度学术
8. 杨洋，尤龙辉，叶功富，聂森，程分生，余锦林. 沙质海岸基干林木麻黄幼林模拟抚育预测. 福建农林大学学报(自然科学版). 2021(02): 206-215 . 百度学术
9. 田红灯，申文辉，谭一波，郑威，何琴飞，朱慧，甘国娟. 不同林龄杉木人工林冠幅与生长因子的关系. 中南林业科技大学学报. 2021(05): 93-101 . 百度学术
10. 朱晋梅，朱光玉，易烜，杨琬珑，牟村，王琢玙. 湖南省栎类次生林冠幅—胸径模型模拟研究. 湖南林业科技. 2021(03): 46-51 . 百度学术
11. 赵保国，朱江，艾训儒，姚兰，郭秋菊，洪建峰. 水杉原生种群胸径树高与树冠的通径分析. 东北林业大学学报. 2021(10): 16-20 . 百度学术
12. 于晓池，李凤，欧阳，张鹏，郭小龙，肖遥，赵秋玲，杨桂娟，王军辉，麻文俊. 基于表型的灰楸核心种质构建. 林业科学研究. 2021(06): 38-45 . 百度学术
13. 贾鹏刚，夏凯，董晨，冯海林，杨垠晖. 基于无人机影像的银杏单木胸径预估方法. 浙江农林大学学报. 2019(04): 757-763 . 百度学术
14. 张冬燕，王冬至，范冬冬，张健东，李大勇. 不同立地类型华北落叶松人工林冠幅与胸径关系研究. 林业资源管理. 2019(04): 69-73 . 百度学术
15. 伍小敏，徐春，杨汉波，陈炙，郭洪英，黄振，王泽亮. 四川桤木天然林和人工林的单木生长模型研究. 四川林业科技. 2018(04): 8-11+44 . 百度学术
16. 周凤艳. 沙地樟子松不同林龄树高、胸径等生长指标的关系研究. 吉林林业科技. 2017(01): 12-15 . 百度学术
其他类型引用(13)
计量
- 文章访问数: 1057
- HTML全文浏览量: 288
- PDF下载量: 72
- 被引次数: 29
