基于非线性分位数混合效应构建杉木树高曲线模型

王宝莹; 梁瑞婷; 谢运鸿; 邱思玉; 孙玉军

doi:10.12171/j.1000-1522.20220496

基于非线性分位数混合效应构建杉木树高曲线模型

北京林业大学森林资源和环境管理国家林业和草原局重点开放性实验室，北京 100083

基金项目: 国家自然科学基金项目（31870620），林业科学技术推广项目（[2019]06）。

详细信息

作者简介:
王宝莹。主要研究方向：林业模型模拟。Email：wbying27@163.com　地址：100083 北京市海淀区清华东路35号北京林业大学林学院

责任作者:
孙玉军，博士，教授。主要研究方向：林业模型模拟。Email：sunyj@bjfu.edu.cn　地址：同上。

中图分类号: S757.2
计量
- 文章访问数: 555
- HTML全文浏览量: 135
- PDF下载量: 73
出版历程
- 收稿日期: 2022-12-06
- 修回日期: 2022-12-26
- 网络出版日期: 2023-11-05
- 刊出日期: 2023-11-29

Construction of Cunninghamia lanceolata tree height curve model based on nonlinear quantile mixed effect

Key Open Laboratory of State Forestry and Grassland Administration of Forest Resources and Environmental Management, Beijing Forestry University, Beijing 100083, China

摘要

摘要:
目的
探索树高−胸径模型构建新方法，将分位数回归与非线性混合效应法相结合应用于树高−胸径模型构建，以此提高模型的拟合精度。
方法
利用2018年福建省将乐国有林场30 m × 30 m固定样地1 306株杉木的实测树高、胸径数据，从4个树高−胸径模型中筛选拟合效果最好的为基础模型，基于基础模型分别采用非线性混合效应、分位数回归以及非线性分位数混合效应构建树高−胸径模型。采用评价指标均方根误差（RMSE）、调整后决定系数（ ${R}_{\mathrm{a}\mathrm{d}\mathrm{j}}^{2}$ ）和均方差（MSE），对各模型的拟合结果进行评价比较，采用赤池信息准则（AIC）、贝叶斯信息准则（BIC）以及对数似然函数值（Loglik）比较各最优模型的拟合精度和预测精度。
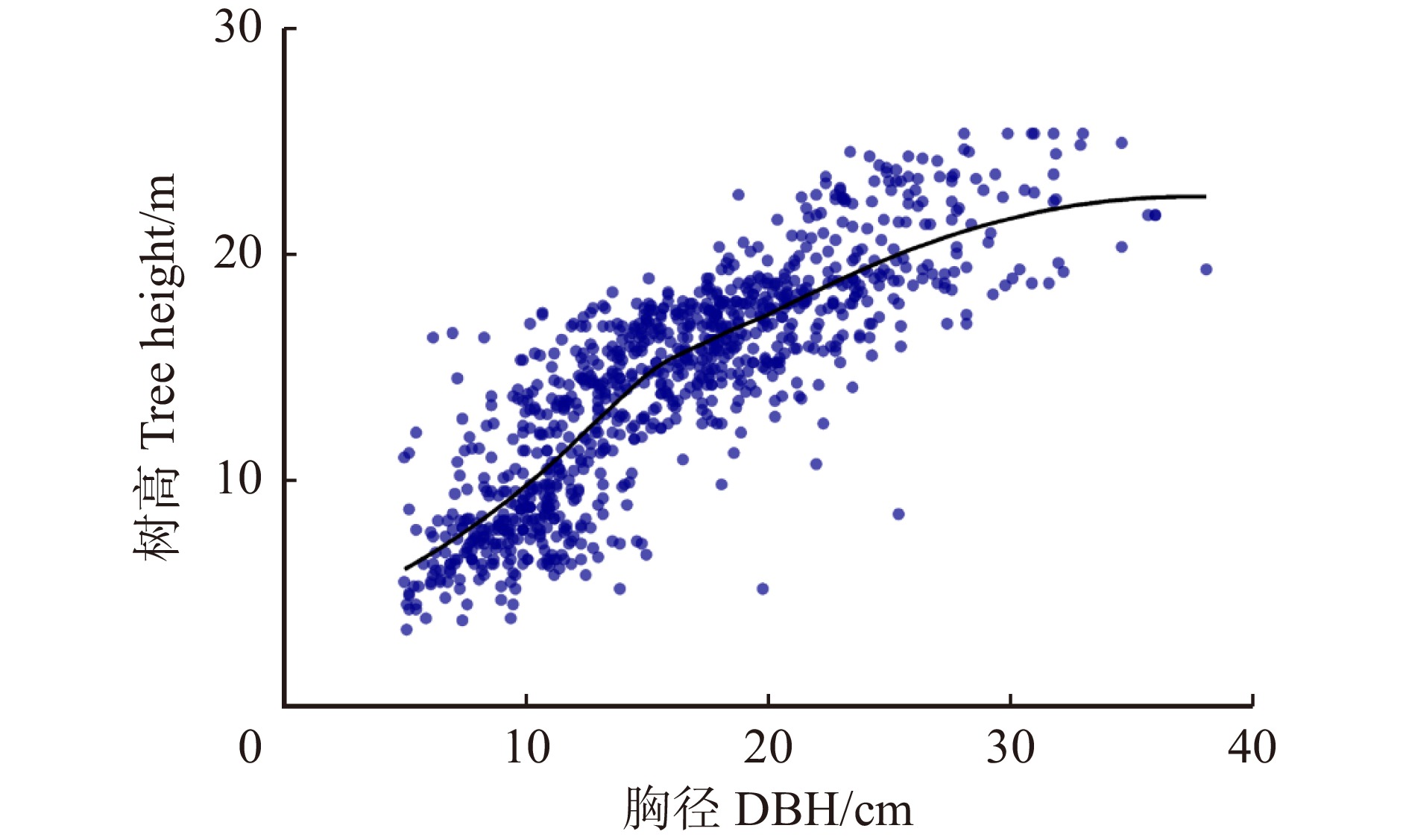
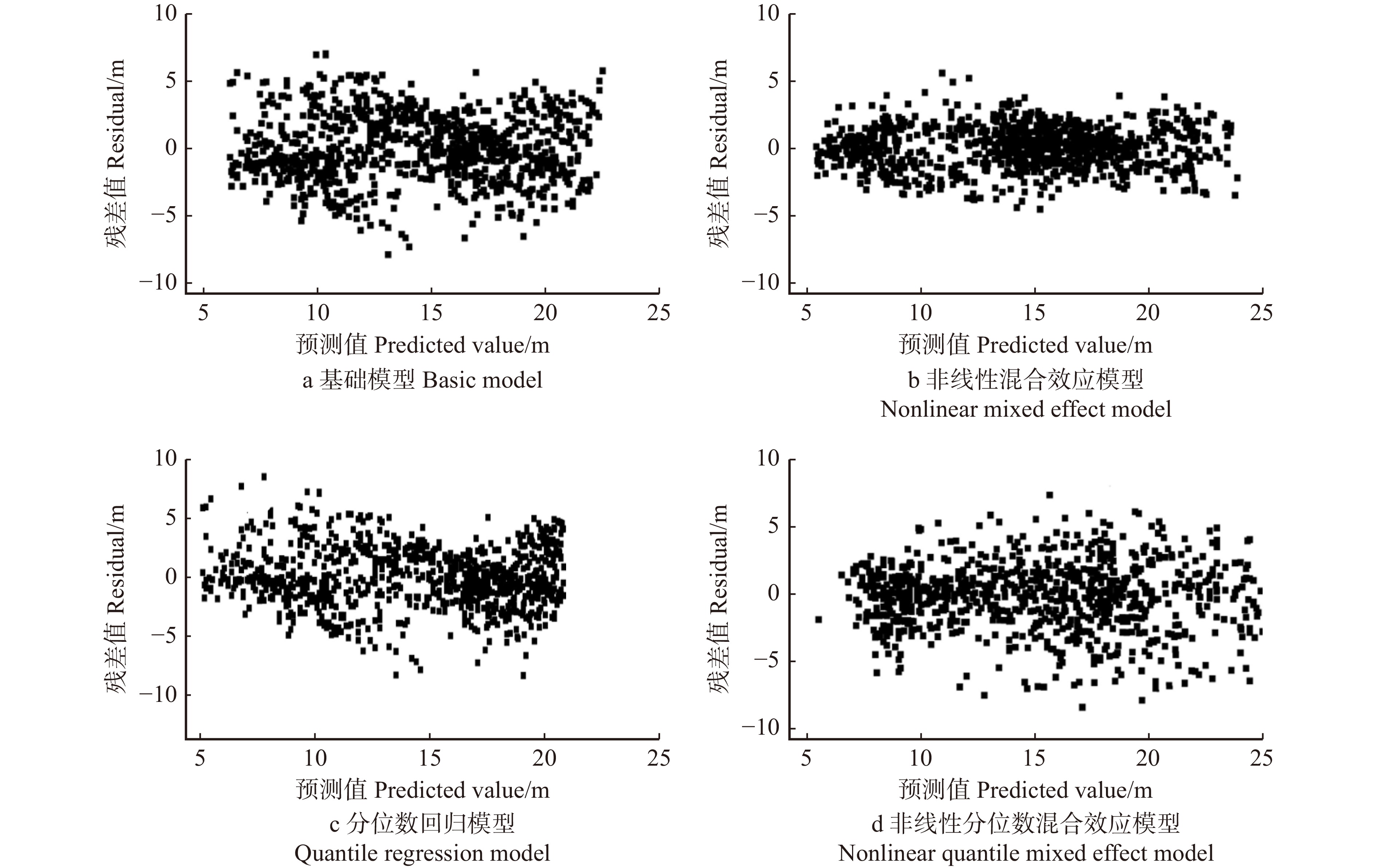
结果
根据评价指标对比显示，Logistic模型为基础模型。非线性混合效应模型的拟合效果最优（AIC为3 953.986，BIC为3 988.199，Loglik为−1 969.993），非线性分位数混合效应模型（AIC为3 979.418，BIC为4 028.293，Loglik为−1 979.709）次之。模型拟合效果排序为非线性混合效应模型 > 非线性分位数混合效应模型 > 基础模型 > 分位数回归模型。比较各模型的残差图可知各模型均不存在异方差现象，预测效果排序为非线性混合效应模型 > 非线性分位数混合效应模型 > 基础模型 > 分位数回归模型。
结论
本研究将分位数回归与非线性混合效应法相结合，该方法对分组数据结构中不同分位点个体间的差异与关联做出解释，提高了模型的稳定性以及拟合精度，将该方法应用到树高−胸径关系的研究上是一个可行的思路，为构建树高−胸径模型提供新方法。
- 非线性分位数混合效应模型 /
- 杉木 /
- 树高−胸径模型
Abstract:
Objective
This paper aims to explore a new method for constructing tree height-DBH model, and combine quantile regression with nonlinear mixed effect method to construct tree height-DBH model, so as to improve the fitting accuracy of the model.
Method
Based on the measured tree height and DBH data of 1 306 Cunninghamia lanceolata trees in the 30 m × 30 m fixed sample plot of C. lanceolata in Jiangle State-Owned Forest Farm of Fujian Province, eastern China in 2018, the basic model with the best fitting effect was selected from four tree height-DBH models. Based on the basic model, the tree height-DBH model was constructed by nonlinear mixed effect, quantile regression and nonlinear quantile mixed effect. The evaluation indexes of RMSE, $R_{\mathrm{adj}}^2$ and MSE were used to evaluate and compare the fitting results of each model. Akaike information criterion(AIC), Bayesian information criterion(BIC) and log likelihood (Loglik) were used to compare the fitting accuracy and prediction accuracy of each optimal model.
Result
According to the comparison of evaluation indicators, the Logistic model was the basic model. The fitting effect of nonlinear mixed effect model was the best (AIC = 3 953.986, BIC = 3 988.199, Loglik = −1 969.993), and the fitting effect of the nonlinear quantile mixed effect model (AIC = 3 979.418, BIC = 4 028.293, Loglik = −1 979.709) was only slightly lower than that of the nonlinear mixed effect model. The order of model fitting effect was nonlinear mixed effect model > nonlinear quantile mixed effect model > basic model > quantile regression model. By comparing the residual sample plots of each model, it can be seen that there was no heteroscedasticity. The order of prediction effect was nonlinear mixed effect model > nonlinear quantile mixed effect model > basic model > quantile regression model.
Conclusion
This study combines quantile regression with nonlinear mixed effect method. This method explains the differences and associations between individuals at different quantiles in the grouped data structure, and improves the stability and fitting accuracy of the model. It is a feasible idea to apply this method to the study of tree height-diameter relationship, and provides a new method for constructing tree height-DBH model.
- nonlinear quantile mixed effect model /
- Cunninghamia lanceolata /
- tree height-DBH model

HTML全文

从古至今，木材作为一种天然可再生的环保型材料，以其独特的性能和优良的环境学特性深受人们喜爱，并广泛应用于生产生活的各个方面。随着现代科学技术的突飞猛进、人类学理论的不断发展，考古木材作为进行植物考古学和环境考古学研究的一种典型生物材料，正成为专家学者的研究热点，国内外考古作业挖掘出大量的木质文物^[1-2]，例如：“Riksapplet号”沉船^[3]、“小白礁Ⅰ号”沉船^[4]、“南海Ⅰ号”船木^[5]等为出土考古木材的研究提供了丰富的材料。

Crestini等^[6]运用X射线衍射和扫描电镜的方法对埃及古代棺木进行研究，研究发现：考古木材中纤维素、半纤维素、木质素均产生不同程度的降解，小分子化合物发生改变，使考古木材变得脆弱而柔软。Łucejko等^[7]运用热裂解气相色谱–质谱联用法对取自比萨圣罗索雷古船的木材样品进行了主成分分析，样品木质素中愈创木基和紫丁香基的去甲基化表明考古木材中多糖产生了流失。卢芸^[8]指出大多木质文物纤维素结晶区被严重破坏，葡聚糖侧链的乙酰基消失，多糖类物质遭到严重降解，木质素相对含量明显增加。

考古木材微观结构的变化，将在很大程度上影响其水分吸着行为，因而可利用等温吸附曲线和水分吸着理论对考古木材与吸着水分子的相互作用进行分析，如Guggenheim-Anderson-de Boer模型^[9]、Hailwood-Horrobin模型^[10]等。Guo等^[11]利用Guggenheim-Anderson-de Boer模型计算得到每千克古代润楠木（Machilus pingii Cheng ex Yang）样品的最大单层吸水量为7.388 kg，而相同树种现代木材的最大单层吸水量仅为4.602 kg，考古木材的最大单层吸水量比现代木材增加了61%。

木材的水分吸着过程必然伴随着热量或能量的变化^[12]，因此基于吸附热力学考察水分吸着机理，有助于从本质上获得考古木材与水分相互作用方面的信息。曹金珍^[13]发现西藏云杉（Picea spinulosa）在水分吸着过程中微分吸着热、微分吸着熵值随吸湿过程不断增大。Simón 等^[14]研究了在吸湿和解吸平衡态下辐射松（Pinus radiata）的微分吸着熵值随含水率的变化曲线，结果显示在相同平衡含水率下辐射松解吸的微分吸着熵值大于吸湿值。

在以往的研究中，研究者多从成分、结构角度对考古木材水分吸着行为进行研究，且取得了一定进展，而对考古木材的吸附热力学研究较少，而且对各种热力学量变化的系统分析也很欠缺。本研究将聚焦考古木材在吸湿和解吸平衡状态下微分吸着热、自由能变化及微分吸着熵三大热力学量的研究，并分别利用电子显微镜（scanning electron microscope，SEM）、傅里叶红外光谱（Fourier transform infrared spectroscopy，FTIR）分析考古木材的细胞壁结构和化学成分变化，从而建立考古木材微观结构与其水分吸着行为间的构效关系，探究考古木材与现代木材在水分吸附热力学方面的差异及其原因，为从能量角度解析考古木材与水分之间的相互作用机制奠定理论基础，同时也可为我国木质文物的保护提供科学依据，以减少出土饱水木质文物与环境中水分的相互作用，提高木质文物的尺寸稳定性。

1. 材料与方法

1.1 材　料

本研究所用考古木材取自成都文物考古研究院发掘出土的南宋木棺残片，由研究院提供的材料和信息确定为考古柏木，并采集四川产柏木（Cupressus sp.）作为现代对照材。

1.2 细胞壁微观形貌表征

利用滑动切片机（REM-710，Yamato Kohki industrial Co.，Ltd，Japan）制得尺寸为1 mm（轴向） × 2 mm（弦向） × 2 mm（径向）的柏木考古木材与现代木材薄片试样（试样重复数为3），用于SEM观察。在80 ℃的鼓风干燥箱中将试样干燥至质量恒定后进行喷金处理，设置SEM（S-3400，Hitachi，日本）加速电压为3 kV，对柏木试样的细胞结构进行观察，初步了解考古木材的降解、腐朽情况。

1.3 化学成分表征

利用研磨机将考古木材与现代木材样品加工成100目以下的木粉，并在80 ℃的真空干燥箱中干燥至质量恒定，之后将木粉与溴化钾充分混合研磨压制成透明薄片，利用红外光谱仪（BRUKER vertex 70 V，德国）对试样进行检测分析，扫描范围设为400 ~ 4 000 cm⁻¹，分辨率为4 cm⁻¹，每个试样扫描次数为32次。重复测试3次，取平均值。

1.4 吸附热力学量测定

将柏木考古木材、现代木材样品加工为20 mg左右木片，并分别置于（103 ± 2）℃下干燥至质量恒定，用于动态水分吸附分析（dynamic vapor sorption，DVS）实验。将各组试样放入DVS中，分别预设恒定温度为25和50 ℃，相对湿度以10%为梯度从0%增加到90%，继续增加至95%，然后降回至90%，再以10%为梯度从90%降低到0%，试样在每个相对湿度下逐渐达到吸湿/解吸平衡后（当样品质量变化速率连续在10 min内小于0.000 1%/min，则认为样品达到吸湿/解吸平衡），仪器自动进入下一个相对湿度梯度。仪器每隔1 min记录样品质量、温度和相对湿度等数据，得到考古木材和现代木材的吸湿和解吸平衡的等温吸附曲线。

利用Hailwood-Horrobin水分吸着理论^[10]分析所获得的等温吸附曲线，木材在不同相对湿度条件下达到的平衡含水率可表达为

$M=M_{{\rm{h}}} + M_{{\rm{d}}}= \frac{18}{m}\left(\frac{{K}_{\mathrm{h}}{K}_{\mathrm{d}}H}{1 + {K}_{\mathrm{h}}{K}_{\mathrm{d}}H} + \frac{{K}_{\mathrm{d}}H}{1-{K}_{\mathrm{d}}H}\right)$

(1)

式中：M为试样平衡含水率（%）；M_h为水合水质量分数（%）；M_d为溶解水质量分数（%）；m为单位摩尔数吸着位点的绝干木材质量（g/mol）；K_h为水合水与溶解水之间的平衡常数；K_d为溶解水与环境温湿度之间的平衡常数；H为相对湿度（%）。

将式（1）整理成多项式，具体为

$\frac{H}{M}=A + BH-C{H}^{2}$

(2)

式中：A、B、C为多项式的拟合参数，A、B、C与K_h、K_d、m的关系为

$A=\frac{m}{18} \times \frac{1}{{K}_{\mathrm{d}}({K}_{\mathrm{h}} + 1)}$

(3)

$B=\frac{m}{18}\times \frac{{K}_{\mathrm{h}}-1}{{K}_{\mathrm{h}} + 1}$

(4)

$C=\frac{m}{18}\times \frac{{K}_{\mathrm{h}}{K}_{\mathrm{d}}}{{K}_{\mathrm{h}} + 1}$

(5)

通过不同相对湿度环境试样的等温吸湿曲线数据分析可得到拟合参数A、B、C的值，进而可以求解m、K_h和K_d的值，公式中 $\dfrac{m}{18}$ 的倒数 $\dfrac{18}{m}$ 表示木材中有效吸着基团被水合水饱和时的含水率，间接表达了水分吸着位点的数量。

利用各组试样的DVS数据拟合等温吸附曲线，进一步通过Clausius-Clapeyron公式^[15]分别计算考古木材与现代木材的微分吸着热Q_S、自由能变化ΔG和微分吸着熵ΔS，分析考古木材与现代木材在吸附热力学量间的差异，各热力学量的计算公式如下。

${Q_{\text{S}}} = 0.254\;{{{\text{d}}\left( {\ln \frac{1}{H}} \right)} \mathord{\left/ {\vphantom {{{\text{d}}\left( {\ln \frac{1}{H}} \right)} {{\text{d}}\left( {\frac{1}{T}} \right)}}} \right.} {{\text{d}}\left( {\frac{1}{T}} \right)}}$

(6)

$\Delta G= -\frac{\mathrm{R}T}{18} \ln\;H$

(7)

$\Delta S= Q_{\rm{S}} −\Delta G/T$

(8)

式中：T为温度（K）；R为气体常数。

T和H的值均由DVS实验的拟合数据获取，然后分别通过公式（6）、（7）计算得到Q_S和ΔG的值，进一步计算得到ΔS。

2. 结果与讨论

2.1 细胞壁结构

图1为考古木材与现代木材横切面、纵切面的SEM图片。对比考古木材与现代木材图像，可以发现在经历长时间的饱水腐朽下，考古木材除复合胞间层、细胞角隅保存相对完整外^[16-17]，其细胞壁结构发生了明显的变化，与现代木材完整有序的细胞排列相比（图1b），考古木材由于长时间遭受微生物降解，细胞结构发生破坏，细胞壁产生腐朽。

图 1 考古木材和现代木材的SEM图

Figure 1. SEM images of archaeological wood and recent wood

下载: 全尺寸图片幻灯片

具体而言，考古木材细胞壁上出现少量圆形孔洞（图1a），除此之外，从考古木材纵切面可见纹孔膜腐朽细节（图1c），并有菌丝体的存在（图1d），该菌丝体是导致考古木材在所处环境中受到降解的各类真菌的聚集体。Guo等^[11]通过氮气吸附法和压汞法对山桐子（Idesia polycarpa）考古木材多孔结构的研究结果表明，考古木材的中孔隙的总孔体积比现代木材样品增加了471%，这也进一步证明了本研究中考古木材孔洞增多的事实。

2.2 化学成分变化

对等量考古木材与现代木材样品的红外光谱进行归一化处理后，可用吸收峰高度半定量表示吸收峰所代表基团的含量^[18]，通过对比考古木材与现代木材试样红外光谱（图2）的特征吸收峰位置变化及消失情况，结合各特征吸收峰所代表的化学基团，研究得到考古木材3种主要化学成分（纤维素、半纤维素、木质素）的含量变化情况及极性基团−OH、−COOH类型和含量的变化情况。

图 2 考古木材与现代木材的红外光谱图

Figure 2. FTIR spectra of archaeological wood and recent wood

下载: 全尺寸图片幻灯片

3 337 ~ 3 401 cm⁻¹范围内的吸收峰代表极性基团−OH（O−H伸缩振动），考古木材在此处的吸收峰高度小于现代木材，证明考古木材中−OH含量减少。选取1 742 cm⁻¹（半纤维素C=O伸缩振动）、1 231 cm⁻¹（半纤维素酰氧键−COO伸缩振动）为半纤维素的代表特征峰，与现代木材相比，柏木考古木材的红外吸收光谱在1 742、1 231 cm⁻¹处吸光度变小，特别是在1 742 cm⁻¹处吸收峰几乎完全消失，证明考古木材细胞壁结构中的半纤维素降解严重；另一方面，1 231 cm⁻¹吸收峰代表半纤维素酰氧键−COO，考古木材在1 231 cm⁻¹处的吸收峰高度明显小于现代木材，证明考古木材半纤维素上的−COOH含量减少。

此外，由于考古木材纤维素结晶区中纤维素链的氢键网络的部分断裂，导致892 cm⁻¹处的峰几乎消失，而该处波峰代表C−H弯曲振动，并且对氢键系统的性质敏感，因此该峰几乎消失表明，在考古木材试样中形成更稳定氢键的羟基数量减少，同时这也表明考古木材中无论是无定形区还是结晶区中的纤维素链都发生部分降解^[19]。

指纹区吸收光谱峰数多、较为复杂，包含了更多能反映分子结构细微变化的信息^[20]。为了提供更多的信息，将FTIR光谱图分析细化至3个指纹区域（1 550 ~ 1 800 cm⁻¹、1 200 ~ 1 550 cm⁻¹和850 ~ 1 200 cm⁻¹），在这个范围内通过直接的红外光谱分析考古木材和现代木材仍存在一定局限性，因为指纹区往往存在高度重叠的峰，难以分辨和解析。为此，通过对考古木材和现代木材指纹区光谱进行分峰拟合处理，得到分峰拟合曲线，可以更直观地分析考古木材化学成分的变化。

通过对比考古木材和现代木材FTIR在1 550 ~ 1 800 cm⁻¹波数范围内的分峰拟合曲线（图3a）可以发现，考古木材在1 654 cm⁻¹（木质素中共轭C=O伸缩振动）处吸收峰吸光度值较高，这说明考古木材木质素相对含量升高。通过对比考古木材和现代木材FTIR在1 200 ~ 1 550 cm⁻¹波数范围内的分峰拟合曲线（图3b）可以发现，考古木材在1 418 cm⁻¹（木质素甲基中C−H弯曲振动）处吸收峰强度变大，代表考古木材木质素相对含量升高。通过对比分析考古木材和现代木材FTIR在850 ~ 1 200 cm^–1波数范围内的分峰拟合曲线（图3c）可以得到：考古木材在892 cm^–1（纤维素C−H弯曲振动）处峰几乎消失，这表明在考古木材中形成更稳定氢键的羟基数目减少；1 159 cm^–1（综纤维素C−O−C变形振动）处，考古木材吸收峰吸光度值很低，这表明综纤维素也有一定程度的降解。

图 3 考古木材与现代木材在1 550 ~ 1 800 cm^–1（a）、1 200 ~ 1 550 cm^–1（b）、850 ~ 1 200 cm^–1（c）波数范围内的分峰拟合曲线

Figure 3. Deconvolution of the “fingerprint” region for archaeological wood and recent wood FTIR spectrum 1 550 − 1 800 cm^–1 (a), 1 200 − 1 550 cm^–1(b), 850 −1 200 cm^–1(c)

下载: 全尺寸图片幻灯片

综上可以发现，考古木材与现代木材相比，半纤维素、纤维素降解严重，纤维素骨架结构受到破坏，因此在SEM下可观察到细胞壁产生腐朽，这种化学成分和化学结构的变化进一步导致了细胞壁孔洞的出现，从化学成分变化角度解释了SEM中观察到考古木材出现孔洞等腐朽现象的原因。通过Xia等^[21]的研究，考古木材木质素的化学结构也发生了变化，木质素仍会有降解，但考古木材中多糖类物质（纤维素、半纤维素）比芳环类物质（木质素）降解程度更高，因而木质素相对含量总体有升高趋势^[22-23]。除纤维素、半纤维素含量降低及木质素相对含量升高的变化外，考古木材中极性基团−OH、−COOH含量均减少，导致考古木材对水分的吸着减少。

2.3 吸附热力学分析

2.3.1 Hailwood-Horrobin模型拟合

通过Hailwood-Horrobin模型拟合等温吸附曲线，不仅可以得到给定温度下木材吸湿和解吸任意平衡含水率状态下的相对湿度，更可以通过研究考古木材与现代木材的曲线差异，帮助理解考古木材水分吸附行为的差异本质。

根据Hailwood-Horrobin模型分别拟合出在25和50 ℃下考古木材、现代木材的等温吸附曲线（图4）。从中可以看出各等温吸附曲线的拟合度R²值均高于0.99，即Hailwood-Horrobin模型能够较好地描述考古木材及现代木材的等温吸附曲线。所有的等温吸附曲线均呈现“S”形，这说明无论考古木材还是现代木材都属于Ⅱ类等温吸附曲线，具备多孔材料的吸附特征^[24-25]。在给定温度的每一个相对湿度条件下，考古木材的平衡含水率均高于现代木材，以温度为50 ℃，相对湿度为0.6时为例，考古木材平衡含水率为7.94%，现代木材为7.46%，考古木材是现代木材的1.1倍。这是因为图1a的 SEM图像显示考古木材物理环境遭到改变，细胞壁孔洞增多，即木材降解程度越大，木材细胞壁内孔隙越多，对水分的容纳空间增加，含水率越高，所以考古木材在经历长时间的降解后平衡含水率高于现代木材。

图 4 考古木材与现代木材的等温吸附曲线

Figure 4. Adsorption isotherms of archaeological wood and recent wood

下载: 全尺寸图片幻灯片

图5为50 ℃下考古木材吸湿和解吸平衡状态的等温吸附曲线，图5显示考古木材的解吸曲线始终位于吸湿之上，即出现了水分吸湿滞后现象。表1总结了在不同相对湿度条件下，考古木材、现代木材分别在25、50 ℃下的滞后率。从表1可以看出：随着温度或相对湿度的升高，考古木材和现代木材的滞后率均增大，即水分吸湿滞后均减小，这与以往研究结果一致^[13]；另一方面，考古木材与现代木材的水分吸湿滞后大小差异不大。

图 5 50 ℃下考古木材的吸湿和解吸等温吸附曲线

Figure 5. Adsorption and desorption isotherms of archaeological wood at 50 ℃

下载: 全尺寸图片幻灯片

2.3.2 吸湿平衡状态下的热力学量分析

2.3.2.1 微分吸着热

图6是吸湿平衡状态下考古木材与现代木材中水分的微分吸着热（Q_S）随含水率（moisture content，MC）的变化曲线，Q_S值越大表示木材中水分子与周围分子之间的结合力越强^[13]。与以往研究结果略有不同的是，在MC为5%处产生一明显拐点。根据曲线变化趋势，可以将曲线分为两个阶段，当MC小于 5%的低含水率状态下时，Q_S值逐渐增大直至达到最大值，这表明水分子和木材之间的氢键结合首先表现为单层分子之间的相互结合，且随MC增大，单个水分子和木材中的−OH形成了更多的氢键结合，相互作用更强，形成的键能更高^[18]。当含水率大于5%时，Q_S值随MC的增大而减小，此时单分子层吸着水几乎达到饱和，吸着水分子与木材实质之间形成的氢键结合作用变弱，键能降低^[13]。

表 1 考古木材、现代木材在25、50 ℃下不同相对湿度下的吸湿滞后

Table 1. Moisture sorption hysteresis of archaeological wood and recent wood under different relative humidities at 25 and 50 ℃

相对湿度 Relative humidity	滞后率 Hysteresis rate
相对湿度 Relative humidity	25 ℃考古木材 25 ℃-archaeological wood	50 ℃考古木材 50 ℃-archaeological wood	25 ℃现代木材 25 ℃-recent wood	50 ℃现代木材 50 ℃-recent wood
0.1	0.62	0.74	0.63	0.71
0.2	0.63	0.72	0.64	0.73
0.3	0.64	0.72	0.65	0.74
0.4	0.66	0.73	0.67	0.76
0.5	0.68	0.75	0.70	0.78
0.6	0.71	0.77	0.73	0.81
0.7	0.76	0.81	0.77	0.84
0.8	0.82	0.86	0.84	0.89
0.9	0.91	0.94	0.93	0.95

下载: 导出CSV

| 显示表格

图 6 考古木材与现代木材中水分的微分吸着热（Q_S）随含水率（MC）的变化曲线

Figure 6. Variation curves of differential heat of sorption (Q_S) of moisture in archaeological wood and recent wood against moisture content (MC)

下载: 全尺寸图片幻灯片

考古木材与现代木材相比，整体曲线形状基本相同，但考古木材的Q_S值随MC的变化曲线位于现代木材之下。根据上文FTIR分析，考古木材化学成分中纤维素、半纤维素大量降解，因此外界水分子可结合−OH、−COOH位点数量减少，这种化学环境的改变导致吸着水与木材分子之间的结合减少，Q_S值降低^[26]。

2.3.2.2 自由能变化

水分的自由能变化的物理意义是水分子润胀木材并切断木材分子相互间氢键结合，裸露木材吸着点所需做的功^[13]。基于图7所示吸湿平衡状态下考古木材与现代木材中水分的自由能变化ΔG值随MC的变化曲线，可以发现考古木材与现代木材的ΔG值均随MC的增大而减小。ΔG值与MC变化呈负相关可以解释为，MC升高使木材可塑性增大，吸着水分子与木材实质分子的协同运动加剧，因此裸露木材吸着点所需做的功减少。

图 7 考古木材（a）与现代木材（b）中水分的自由能变化（ΔG）随含水率的变化曲线

Figure 7. Variation curves of free energy change (ΔG) of moisture in archaeological wood (a) and recent wood (b) against moisture content

下载: 全尺寸图片幻灯片

其次，无论是考古木材或是现代木材，50 ℃相较于25 ℃的ΔG值都有一定程度的减小，这是因为温度升高吸着水分子能量增加，活动加剧，所需做的功减小。

2.3.2.3 微分吸着熵

微分吸着熵（ΔS）体现了木材中吸着的水分子与液态水分子相比排列规则上的差异，ΔS > 0代表木材中水分子排列更有规律^[13]。根据吸湿平衡状态下考古木材与现代木材中水分的ΔS值随MC的变化曲线（图8）可知：ΔS值与Q_S值随MC的变化曲线在MC小于5%呈现相似的变化趋势，都随MC的增大而增大。在MC小于5%的低含水率阶段，ΔS < 0表明木材中吸着的水分子比液态水分子更无序，这是因为此时木材中吸着的水分子主要为单分子层吸着水，它们比处于液体状态的水分子显示出更无序的状态；在经过5%的拐点之后，ΔS值随MC变化不明显。而随着MC的增大，ΔS值经历了由负到正、由无序到有序的变化，这说明吸着在木材中的水分子排列越来越规则。

图 8 考古木材与现代木材中水分的微分吸着熵（ΔS）随含水率的变化曲线

Figure 8. Variation curves of differential entropy of sorption (ΔS) of moisture in archaeological wood and recent wood againstmoisture content

下载: 全尺寸图片幻灯片

进一步分析考古木材与现代木材的曲线差异，考古木材ΔS值低于现代木材，这是因为考古木材在经历长期酸碱环境下软腐菌等真菌的降解后^[27]，细胞壁出现的孔洞分布是随机的，且与水结合的−OH分布也十分不均匀，造成考古木材中吸着的水分子排列更无序。

2.3.3 水分吸湿与解吸的比较

以50 ℃考古木材为例，分别绘制Q_S、ΔG、ΔS三大热力学量随MC的变化曲线，每张图包含吸湿和解吸平衡态下的两条曲线（图9）。发现吸湿和解吸变化曲线形状基本一致，但解吸的Q_S、ΔG和ΔS值均大于吸湿的值^[14]，对应于水分吸湿滞后（图5），这个现象即为“热力学吸湿滞后”，其表征在吸湿与解吸平衡态下，每一个吸着水分子与木材吸着点之间的氢键结合平均数的差异^[13]。

图 9 50 ℃下考古木材吸湿与解吸过程中的Q_S（a）、ΔG（b）、ΔS（c）随含水率的变化曲线

Figure 9. Variation curves of Q_S (a), Δ G (b), Δ S (c) in archaeological wood against moisture content during moisture adsorption and desorption at 50 ℃

下载: 全尺寸图片幻灯片

热力学吸湿滞后同样可以用水分吸湿滞后的“有效羟基说”^[28]解释，即在干燥状态下，部分木材吸着点之间形成氢键结合，当木材从干燥状态开始吸湿时，由于这部分已经形成的氢键结合的影响，处于游离状态能够吸着水分子的吸着点的数量减少，每个吸着水分子与木材吸着点之间的氢键结合平均数减少，从而吸湿的热力学量也低于解吸过程。

3. 结　　论

为探究考古木材的水分吸着行为与现代木材的差异机理，本研究从考古木材与现代木材微观结构与吸附热力学两个层面进行了研究。

考古木材三大主要成分（纤维素、半纤维素、木质素）均发生不同程度的降解，且考古木材中极性基团−OH、−COOH含量均减少，导致考古木材对水分的吸着减少。其中半纤维素、纤维素降解程度更大，因此在SEM中可观察到细胞壁产生腐朽，且可见孔洞分布；而考古木材中多糖类物质（纤维素、半纤维素）比芳环类物质（木质素）降解程度更高，因而木质素相对含量升高。

在吸附热力学研究中，通过DVS等温吸附实验并利用Hailwood-Horrobin模型拟合等温吸附曲线，在给定温度条件下考古木材的平衡含水率高于现代木材。结合Clausius-Clapeyron公式计算考古木材与现代木材的Q_S、ΔG和ΔS三大热力学量。考古木材因纤维素、半纤维素降解导致−OH、−COOH数量减小，Q_S值低于现代木材；考古木材与现代木材的ΔG值差异不大，且随温度升高而减小；考古木材的细胞壁孔洞、−OH分布不均匀，所以ΔS值低于现代木材；在MC小于5%，考古木材Q_S值和ΔS值随MC的变化曲线趋势相似，在此范围内木材中吸着的水分子主要为单分子层吸着水，随后在5%处产生一拐点，此时单分子层吸着水达到饱和，此后木材中吸着的水分子以多分子层吸着水占主导。最后，通过对比吸湿和解吸过程的热力学量（吸湿 < 解吸），发现考古木材存在热力学吸湿滞后现象。

基于以上对于考古木材水分吸着行为差异的研究，考古木材的吸湿性在经历长时间的泡水、腐朽下发生了较大变化，因此在出土饱水木质文物的保护工作中，建议采用各种物理、化学手段减少木质文物与环境中水分的相互作用，如涂覆防水涂层、浸渍脱水材料等，从而提高木质文物的尺寸稳定性^[29]，为我国出土饱水木质文物的保护提供理论支撑。

图 1 杉木胸径与树高分布

Figure 1. Tree height-DBH distribution of Cunninghamia lanceolata

下载: 全尺寸图片幻灯片

图 2 回归系数随分位数变化图

Figure 2. Regression coefficient plot with quantile change chart

下载: 全尺寸图片幻灯片

图 3 树高−胸径分位数回归模拟

虚线为最小二乘回归直线，实线为不同分位点处分位数回归直线。The dotted line is the least square regression line, the solid line is the quantile regression line for different quantiles.

Figure 3. Regression simulation of tree height-DBH quantile

下载: 全尺寸图片幻灯片

图 4 各个模型的残差分布图

Figure 4. Residual distribution plots for various models

下载: 全尺寸图片幻灯片

表 1 标准地调查数据

Table 1 Standard site survey data

因子 Factor	海拔 Elevation/m	断面积/（m²·hm⁻²） Basal area/(m²·ha⁻¹)	林分密度/（株·hm⁻²） Stand density/(tree·ha⁻¹)	建模数据 Modeling data		检验数据 Validation data
因子 Factor	海拔 Elevation/m	断面积/（m²·hm⁻²） Basal area/(m²·ha⁻¹)	林分密度/（株·hm⁻²） Stand density/(tree·ha⁻¹)	树高 Tree height/m	胸径 DBH/cm	树高 Tree height/m	胸径 DBH/cm
最小值 Min. value	184.00	7.22	467.00	3.40	5.00	4.20	5.20
最大值 Max. value	305.00	41.08	3 267.00	25.30	38.10	27.20	36.60
平均值 Mean	229.80	23.68	1 037.00	14.28	16.07	13.90	15.08
标准差 SD	36.90	9.92	964.00	4.92	6.28	5.10	6.55

下载: 导出CSV

表 2 4种候选的树高−胸径模型

Table 2 Four candidate tree height-DBH models

序号 No.	模型 Model	参数类型 Parameter type	表达式 Expression
1	Richards	三参数 3-parameter	$H=1.3+a(1-\mathrm{e}^{-bD})^c$
2	Logistic	三参数 3-parameter	$H=1.3+\dfrac{a}{1+b\mathrm{e}^{-cD}}$
3	Korf	三参数 3-parameter	$H=1.3+a\left(\mathrm{e}^{\frac{-b}{D^c}}\right)$
4	Ratkowsky	三参数 3-parameter	$H=1.3+a\mathrm{e}^{\frac{-b}{D+c}}$
注：H为树高；D为胸径；a，b，c均为模型参数。Notes: H is tree height, D is DBH, a, b, c are all model parameters.

下载: 导出CSV

表 3 候选基础模型参数估计和评价检验

Table 3 Parameter estimation and evaluation test of candidate basic models

模型 Model	参数 Parameter			评价指标 Evaluation index			排名 Rank
模型 Model	a	b	c	RMSE/m	${R}_{\mathrm{a}\mathrm{d}\mathrm{j}}^{2}$	MSE/m	排名 Rank
Richards	23.896 1	0.078 4	1.665 0	2.568	0.727	6.594	2
Logistic	20.795 2	7.812 1	0.168 7	2.564	0.728	6.575	1
Korf	43.814 8	7.745 7	−0.682 3	2.580	0.725	6.655	4
Ratkowsky	35.696 0	18.363 0	3.039 0	2.574	0.726	6.626	3

下载: 导出CSV

表 4 非线性混合效应模型拟合结果

Table 4 Fitting results of nonlinear mixed effect models

模型 Model	随机参数 Random parameter	参数个数 Number of parameters	参数 Parameter			评价指标 Evaluation index
模型 Model	随机参数 Random parameter	参数个数 Number of parameters	a	b	c	RMSE/m	${R}_{\mathrm{a}\mathrm{d}\mathrm{j}}^{2}$	MSE/m
基础模型 Basic model		3	20.795 2	7.812 1	0.168 7	2.564	0.728	6.575
	${\mu }_{1}$	4	19.054 1	4.002 0	0.152 0	1.778	0.869	3.162
	${\mu }_{2}$	4	23.692 8	3.408 9	0.094 7	1.850	0.858	3.424
	${\mu }_{3}$	4	21.758 3	3.965 8	0.122 3	1.841	0.860	3.390
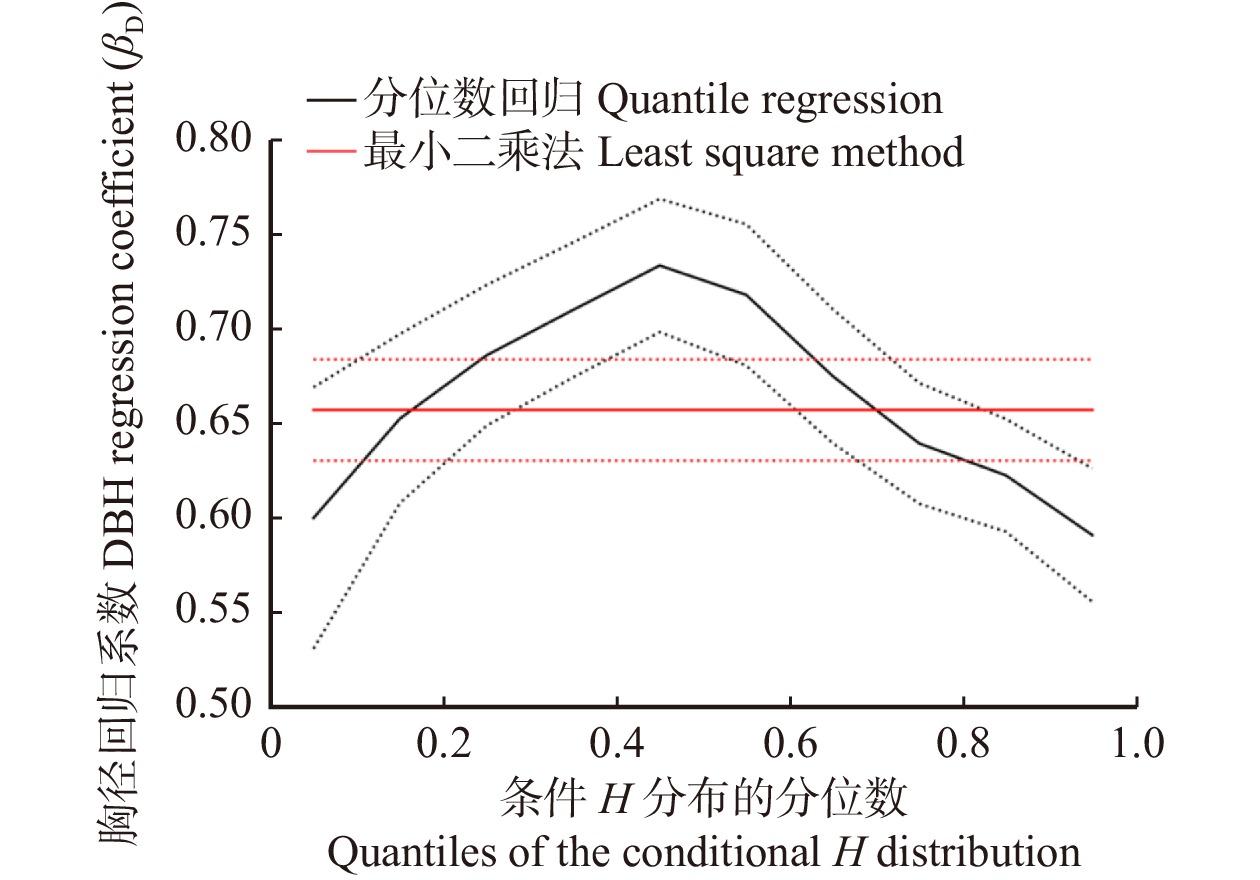
非线性混合效应模型 Nonlinear mixed effect model	${\mu }_{1}$ 、 ${\mu }_{2}$	5	18.958 1	4.020 3	0.153 6	1.720	0.878	2.957
	${\mu }_{1}$ 、 ${\mu }_{3}$	5	18.889 8	5.027 9	0.189 6	1.727	0.877	2.983
	${\mu }_{2}$ 、 ${\mu }_{3}$	5	22.573 3	3.850 9	0.108 2	1.733	0.876	3.003
	${\mu }_{1}$ 、 ${\mu }_{2}$ 、 ${\mu }_{3}$	6	未收敛 Not converged

下载: 导出CSV

表 5 分位数回归模型参数估计及评价

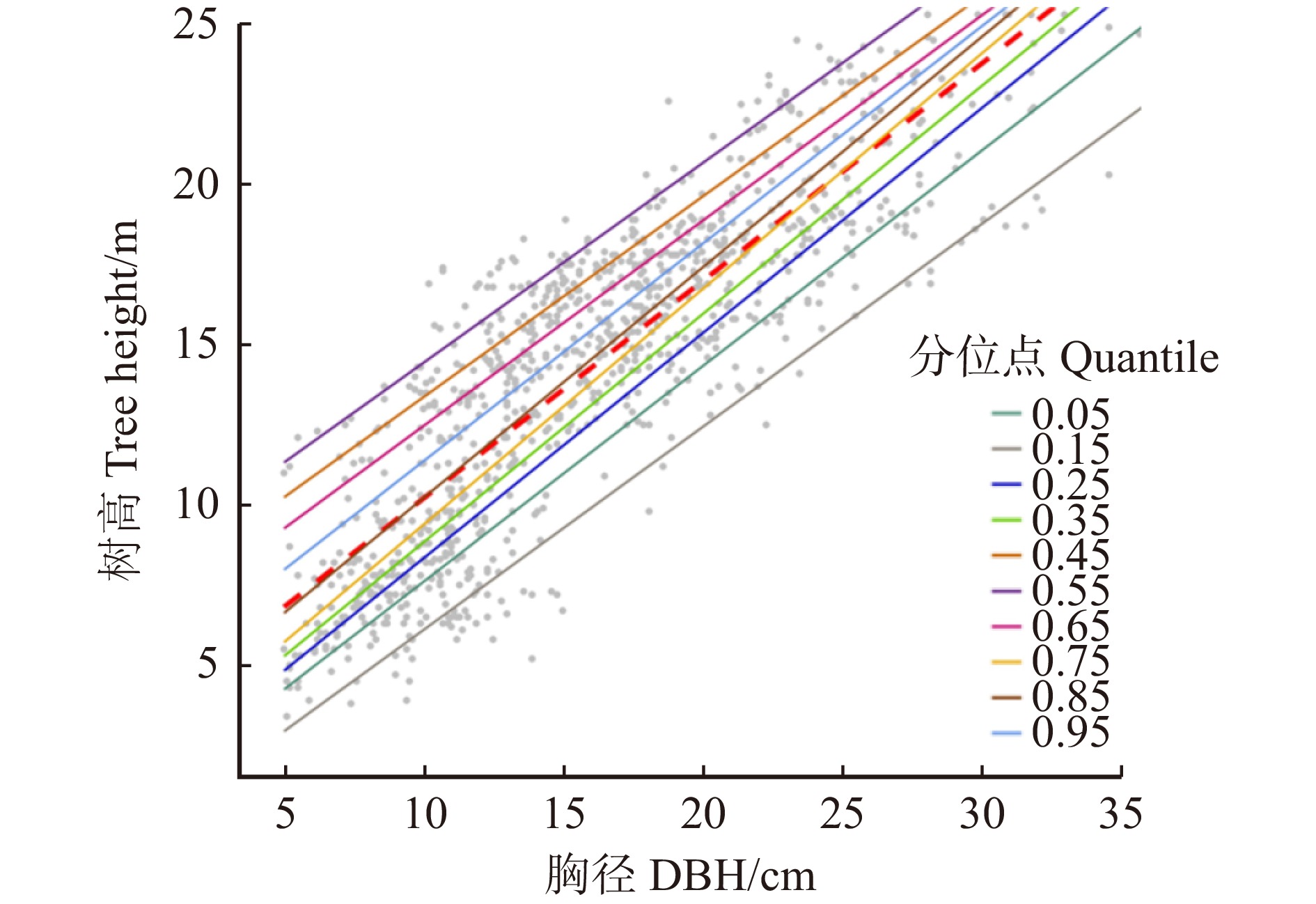
Table 5 Parameter estimation and evaluation of quantile regression model

模型 Model	分类 Classification	参数 Parameter			评价指标 Evaluation index
模型 Model	分类 Classification	a	b	c	RMSE/m	${R}_{\mathrm{a}\mathrm{d}\mathrm{j}}^{2}$	MSE/m
非线性混合效应模型 Nonlinear mixed effect model		18.958 1	4.020 3	0.153 6	1.720	0.878	2.957
分位数回归模型 Quantile regression model	$\tau =0.05$	17.033 8	19.501 0	0.195 5	4.820	0.103	22.067
	$\tau =0.15$	17.937 1	13.874 8	0.194 8	3.557	0.504	12.203
	$\tau =0.25$	18.356 9	13.427 1	0.204 4	3.044	0.641	8.835
	$\tau =0.35$	18.660 3	12.704 3	0.210 2	2.758	0.711	7.125
	$\tau =0.45$	18.926 2	12.574 2	0.216 9	2.632	0.736	6.496
	$\tau =0.55$	19.257 1	12.740 6	0.224 5	2.609	0.744	6.302
	$\tau =0.65$	20.877 4	8.122 7	0.187 5	2.742	0.716	6.980
	$\tau =0.75$	22.939 1	5.673 9	0.153 7	3.139	0.608	9.658
	$\tau =0.85$	26.212 8	4.076 8	0.116 7	3.793	0.431	13.997
	$\tau =0.95$	30.558 9	2.998 2	0.083 3	4.803	0.125	21.524

下载: 导出CSV

表 6 非线性分位数混合效应模型参数估计及评价

Table 6 Parameter estimation and evaluation of nonlinear quantile mixed effect model

项目 Item	参数 Parameter	估计值 Estimated value
参数估计值 Parameter estimate	${a}_{1}$	69.378 8
	${b}_{1}$	19.327 2
	${c}_{1}$	0.067 0
参数误差值 Parameter error value	$a\mathrm{_e}$	5.309 5
	$b\mathrm{_e}$	0.996 2
	$c_{\mathrm{e}}$	0.004 9
评价指标 Evaluating index	AIC	3 979.418
	BIC	4 028.293
	Loglik	−1 979.709
方差−协方差矩阵 Variance-covariance matrix	${\boldsymbol{z}}_{1}^{2}$	1 224.832 1
	${\boldsymbol{z}}_{12}$	230.232 2
	${\boldsymbol{z}}_{13}$	−4.681 7
	${\boldsymbol{z}}_{21}$	230.232 2
	${\boldsymbol{z}}_{2}^{2}$	69.963 7
	${\boldsymbol{z}}_{23}$	−0.542 4
	${\boldsymbol{z}}_{31}$	−4.681 7
	${\boldsymbol{z}}_{32}$	−0.542 4
	${\boldsymbol{z}}_{3}^{2}$	0.023 0
注： ${\boldsymbol{z}}_{1}^{2}$ 、 ${\boldsymbol{z}}_{2}^{2}、{\boldsymbol{z}}_{3}^{2}$ 为随机参数 ${u}_{1}$ 、 ${u}_{2}$ 、 ${u}_{3}$ 的方差； ${\boldsymbol{z}}_{12}$ 为随机参数 ${u}_{1}$ 和 ${u}_{2}$ 的协方差； ${\boldsymbol{z}}_{13}$ 为随机参数 ${u}_{1}$ 和 ${u}_{3}$ 的协方差； ${\boldsymbol{z}}_{21}$ 为随机参数 ${u}_{2}$ 和 ${u}_{1}$ 的协方差； ${\boldsymbol{z}}_{23}$ 为随机参数 ${u}_{2}$ 和 ${u}_{3}$ 的协方差； ${\boldsymbol{z}}_{31}$ 为随机参数 ${u}_{3}$ 和 ${u}_{1}$ 的协方差； ${\boldsymbol{z}}_{32}$ 为随机参数 ${u}_{3}$ 和 ${u}_{2}$ 的协方差。 Notes: ${\boldsymbol{z}}_{1}^{2}$ , ${\boldsymbol{z}}_{2}^{2},{\boldsymbol{z}}_{3}^{2}$ are the variance of random parameters u₁, u₂ and u₃; ${\boldsymbol{z}}_{12}$ are the covariance of random parameters u₁ and u₂; ${\boldsymbol{z}}_{13}$ is the covariance of random parameters u₁ and u₃; ${\boldsymbol{z}}_{21}$ is the covariance of random parameters u₂ and u₁; ${\boldsymbol{z}}_{23}$ is the covariance of random parameters u₂ and u₃; ${\boldsymbol{z}}_{31}$ is the covariance of random parameters u₃ and u₁; ${\boldsymbol{z}}_{32}$ is the covariance of random parameters u₃ and u₂.

下载: 导出CSV

表 7 各模型拟合结果

Table 7 Fitting results of each model

模型 Model	评价指标 Evaluating index			排名 Rank
模型 Model	AIC	BIC	Loglik	排名 Rank
基础模型 Basic model	4 631.666	4 651.216	−2 311.833	3
非线性混合效应模型 Nonlinear mixed effect model	3 953.986	3 988.199	−1 969.993	1
分位数模型 Quantile model	4 658.689	4 673.349	−2 326.345	4
非线性分位数混合效应模型 Nonlinear quantile mixed effect model	3 979.418	4 028.293	−1 979.709	2

下载: 导出CSV

表 8 各模型检验结果

Table 8 Validation results of each model

模型 Model	评价指标 Evaluation index			排名 Rank
模型 Model	RMSE/m	${R}_{\mathrm{a}\mathrm{d}\mathrm{j}}^{2}$	MSE/m	排名 Rank
基础模型 Basic model	2.205	0.813	4.861	3
非线性混合效应模型 Nonlinear mixed effect model	1.487	0.915	2.212	1
分位数回归模型 Quantile regression model	2.256	0.805	5.090	4
非线性分位数混合效应模型 Nonlinear quantile mixed effect model	1.558	0.907	2.427	2

下载: 导出CSV

参考文献(22)

[1]	杜志, 陈振雄, 李锐, 等. 气候敏感的杉木树高−胸径非线性混合效应模型研建[J]. 北京林业大学学报, 2023, 45(9): 52−61. doi: 10.12171/j.1000-1522.20230052 Du Z, Chen Z X, Li R, et al. Development of climate-sensitive nonlinear mixed-effects tree height-DBH model for Cunninghamia lanceolata[J]. Journal of Beijing Forestry University, 2023, 45(9): 52−61. doi: 10.12171/j.1000-1522.20230052
[2]	陈浩, 罗扬. 马尾松树高−胸径非线性混合效应模型构建[J]. 森林与环境学报, 2021, 41(4): 439−448. Chen H, Luo Y. Construction of nonlinear mixed effect height-diameter model for Pinus massoniana[J]. Journal of Forest and Environment, 2021, 41(4): 439−448.
[3]	沈子奕, 林杰. 基于哑变量回归和混合效应的杉树树高−胸径模型[J]. 济南大学学报(自然科学版), 2022, 36(1): 80−85. Shen Z Y, Lin J. Height-diameter at breast height model for Cunninghamia lanceolate based on dummy variable regression and mixed effects[J]. Journal of University of Jinan (Science and Technology), 2022, 36(1): 80−85.
[4]	梁瑞婷, 孙玉军, 李芸. 深度学习和传统方法模拟杉木树高−胸径模型比较[J]. 林业科学研究, 2021, 34(6): 65−72. Liang R T, Sun Y J, Li Y. Comparison of deep learning and traditional models to simulate the height-DBH relationship of Chinese fir[J]. Forest Research, 2021, 34(6): 65−72.
[5]	孙拥康, 汤景明, 王怡. 基于分位数回归的马尾松青冈栎混交林树高−胸径模型[J]. 中南林业科技大学学报, 2021, 41(12): 18−25. Sun Y K, Tang J M, Wang Y. Height-diameter model of Pinus massoniana and Cyclobalanopsis glauca mixed forest based on quantile regression[J]. Journal of Central South University of Forestry & Technology, 2021, 41(12): 18−25.
[6]	Misik T, Antal K, Kárász I, et al. Nonlinear height–diameter models for three woody, understory species in a temperate oak forest in Hungary[J]. Canadian Journal of Forest Research, 2015: 46(11): 1337−1342.
[7]	Huang S S, Titus S J, Wiens D P. Comparison of nonlinear height-diameter functions for major Alberta tree species[J]. Canadian Journal of Forest Research, 1992, 22(9): 1297−1304. doi: 10.1139/x92-172
[8]	佟艺玟, 陈东升, 冯健, 等. 基于线性分位数混合效应的辽东山区红松冠幅模型[J]. 应用生态学报, 2022, 33(9): 2321−2330. doi: 10.13287/j.1001-9332.202209.002 Tong Y W, Chen D S, Feng J, et al. Crown width model for planted Korean pine in eastern Liaoning mountains based on mixed effect linear quantile[J]. Chinese Journal of Applied Ecology, 2022, 33(9): 2321−2330. doi: 10.13287/j.1001-9332.202209.002
[9]	Raptis D I, Kazana V, Kechagioglou S, et al. Nonlinear quantile mixed-effects models for prediction of the maximum crown width of Fagus sylvatica L., Pinus nigra Arn. and Pinus brutia Ten[J/OL]. Forests, 2022, 13(4): 499. [2022−10−31]. https://doi.org/10.3390/f13040499.
[10]	李海奎, 法蕾. 基于分级的全国主要树种树高−胸径曲线模型[J]. 林业科学, 2011, 47(10): 83−90. doi: 10.11707/j.1001-7488.20111013 Li H K, Fa L. Height-diameter model for major tree species in China using the classified height method[J]. Scientia Silvae Sinicae, 2011, 47(10): 83−90. doi: 10.11707/j.1001-7488.20111013
[11]	Grégoire T G, Schabenberger O, Barrett J P. Linear modelling of irregularly spaced, unbalanced, longitudinal data from permanent-plot measurements[J]. Canadian Journal of Forest Research, 2011, 25(1): 137−156.
[12]	Pinheiro J C, Bates D M. Mixed-effects models in S and S-Plus[M]. New York: Springer, 2000.
[13]	Koenker R, Bassett G. Regression quantiles[J]. Econometrica, 1978, 46(1): 33−50. doi: 10.2307/1913643
[14]	田德超, 李凤日, 董利虎. 依据分位数回归建立的长白落叶松潜在最大冠幅预测模型[J]. 东北林业大学学报, 2019, 47(8): 41−46. doi: 10.3969/j.issn.1000-5382.2019.08.008 Tian D C, Li F R, Dong L H. Potential maximum crown width prediction model of Larix olgensis by quantile regression[J]. Journal of Northeast Forestry University, 2019, 47(8): 41−46. doi: 10.3969/j.issn.1000-5382.2019.08.008
[15]	Delyon B, Lavielle M, Moulines E. Convergence of a stochastic approximation version of EM algorithm[J]. The Annals of Statistics, 1999, 27(1): 94−128.
[16]	Akaike H. A new look at the statistical model identification[J]. IEEE Transactions on Automatic Control, 1974, 19(6): 716−723. doi: 10.1109/TAC.1974.1100705
[17]	王冬至, 张冬燕, 李永宁, 等. 基于贝叶斯法的针阔混交林树高与胸径混合效应模型[J]. 林业科学, 2019, 55(11): 85−94. doi: 10.11707/j.1001-7488.20191110 Wang D Z, Zhang D Y, Li Y N, et al. Height-diameter relationship for conifer mixed forest based on Bayesian nonlinear mixed-effects model[J]. Scientia Silvae Sinicae, 2019, 55(11): 85−94. doi: 10.11707/j.1001-7488.20191110
[18]	Xu H, Sun Y J, Wang X J, et al. Height-diameter models of Chinese fir ( Cunninghamia lanceolata) based on nonlinear mixed effects models in southeast China[J]. Advance Journal of Food Science and Technology, 2014, 6(4): 445−452. doi: 10.19026/ajfst.6.53
[19]	Sun Y X, Gao H L, Li F R. Using linear mixed-effects models with quantile regression to simulate the crown profile of planted Pinus sylvestris var. Mongolica trees[J/OL]. Forests, 2017, 8(11): 446 [2022−11−02]. https://doi.org/10.3390/f8110446.
[20]	邓祥鹏, 许芳泽, 赵善超, 等. 基于贝叶斯法的新疆天山云杉树高−胸径模型研究[J]. 北京林业大学学报, 2023, 45(1): 11−20. doi: 10.12171/j.1000-1522.20220318 Deng X P, Xu F Z, Zhao S C, et al. Tree height-DBH model for Picea schrenkiana in Tianshan Mountain, Xinjiang of northwestern China based on Bayesian method[J]. Journal of Beijing Forestry University, 2023, 45(1): 11−20. doi: 10.12171/j.1000-1522.20220318
[21]	Wang J. Bayesian quantile regression for parametric nonlinear mixed effects models[J]. Statistical Methods and Applications, 2012, 21(3): 279−295. doi: 10.1007/s10260-012-0190-7
[22]	Özçelik R, Cao Q V, Trincado G, et al. Predicting tree height from tree diameter and dominant height using mixed-effects and quantile regression models for two species in Turkey[J]. Forest Ecology and Management, 2018, 419: 240−248.

施引文献(9)

期刊类型引用(6)

1.	冯林艳，周火艳，赵晓迪. 乌兰布和沙漠两种植物的分布格局及其变化. 南京林业大学学报(自然科学版). 2024(01): 155-160 . 百度学术
2.	曾红，徐永艳，邵琳亚，闻永慧，夏小丽，汪琼. 4种植物叶片浸提液成分分析及其对珊瑚樱种子萌发的影响. 西南林业大学学报(自然科学). 2023(04): 39-46 . 百度学术
3.	马光宗，徐高峰，杨韶松，杨云海，张付斗，温丽娜，陶琼，申时才，叶敏. 甘薯提取物对3种杂草种子萌发和幼苗生长的化感作用. 西南农业学报. 2022(06): 1295-1302 . 百度学术
4.	路文杰，佛芒芒，肖毅，王永新，杜利霞，钟华，赵祥，董宽虎. 草地植物凋落物浸提液对根际微生物碳源利用的影响. 中国草地学报. 2021(06): 35-42 . 百度学术
5.	张林媚，刘姝玲，郭彩云. 立地条件对榆林沙区樟子松嫁接红松生长的影响. 林业科技通讯. 2021(11): 32-37 . 百度学术
6.	王方琳，尉秋实，柴成武，王理德，张德魁，王昱淇，王飞，胡小柯. 沙蒿（Artemisia desertorum）浸提液对自身种子萌发与幼苗生长的化感作用. 中国沙漠. 2021(06): 21-28 . 百度学术

其他类型引用(3)

资源附件(0)

图(4) / 表(8)

计量

文章访问数: 555
HTML全文浏览量: 135
PDF下载量: 73
被引次数: 9

1. 材料与方法
1.1 材　料
1.2 细胞壁微观形貌表征
1.3 化学成分表征
1.4 吸附热力学量测定
2. 结果与讨论
2.1 细胞壁结构
2.2 化学成分变化
2.3 吸附热力学分析
2.3.1 Hailwood-Horrobin模型拟合
2.3.2 吸湿平衡状态下的热力学量分析
2.3.2.1 微分吸着热
2.3.2.2 自由能变化
2.3.2.3 微分吸着熵
2.3.3 水分吸湿与解吸的比较
3. 结　　论

1. 材料与方法
1.1 材　料
1.2 细胞壁微观形貌表征
1.3 化学成分表征
1.4 吸附热力学量测定
2. 结果与讨论
2.1 细胞壁结构
2.2 化学成分变化
2.3 吸附热力学分析
2.3.1 Hailwood-Horrobin模型拟合
2.3.2 吸湿平衡状态下的热力学量分析
2.3.2.1 微分吸着热
2.3.2.2 自由能变化
2.3.2.3 微分吸着熵
2.3.3 水分吸湿与解吸的比较
3. 结　　论

参考文献(22)

施引文献

资源附件(0)

基于非线性分位数混合效应构建杉木树高曲线模型

作者简介: 王宝莹。主要研究方向：林业模型模拟。Email：wbying27@163.com 地址：100083 北京市海淀区清华东路35号北京林业大学林学院

责任作者: 孙玉军，博士，教授。主要研究方向：林业模型模拟。Email：sunyj@bjfu.edu.cn 地址：同上。

计量

出版历程