应压木细胞壁S<sub>2</sub>层的微纤丝螺旋角对其抗压韧性的影响

刘浩正; 王建山; 石广玉

doi:10.12171/j.1000-1522.20220506

应压木细胞壁S₂层的微纤丝螺旋角对其抗压韧性的影响

天津大学机械工程学院力学系，天津 300354

基金项目: 国家自然科学基金重点国际合作研究项目（1202010100）

详细信息

作者简介:
刘浩正。主要研究方向：生物力学。 Email：ysulhz@163.com　地址： 300354天津市津南区雅观路135号天津大学机械工程学院力学系

责任作者:
石广玉，教授，博士生导师。主要研究方向：固体力学和复合材料力学。Email：shi_guangyu@tju.edu.cn　地址：同上

中图分类号: S781.29；S781.1；O242.2
计量
- 文章访问数: 516
- HTML全文浏览量: 214
- PDF下载量: 92
出版历程
- 收稿日期: 2022-12-13
- 修回日期: 2023-02-28
- 录用日期: 2023-03-19
- 网络出版日期: 2023-03-20
- 发布日期: 2023-04-24

Effects of microfibril helix angle in the S₂ layer of compression wood cell wall on the compressive toughness of it

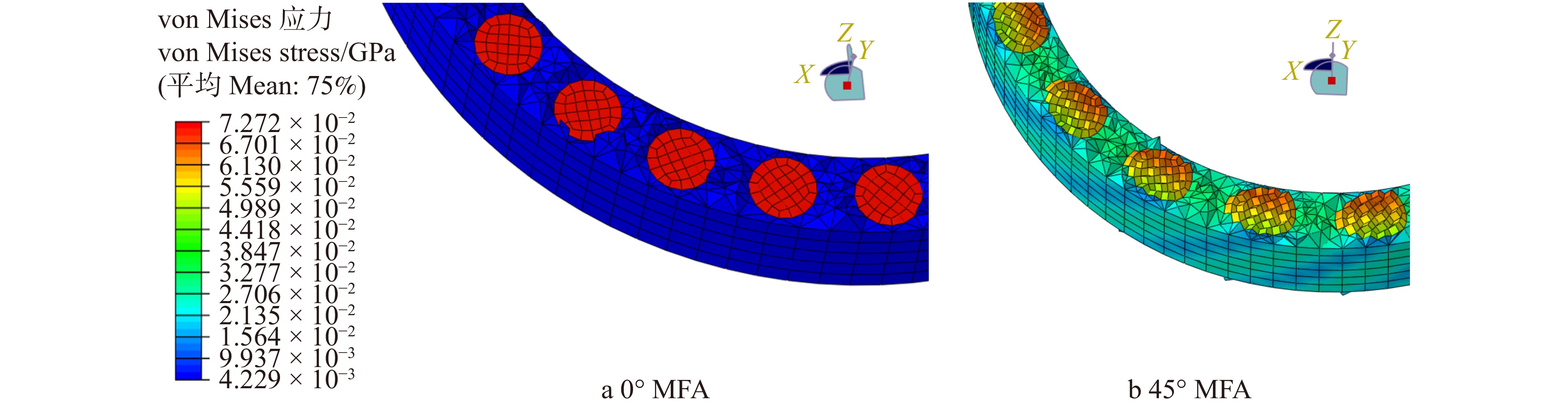
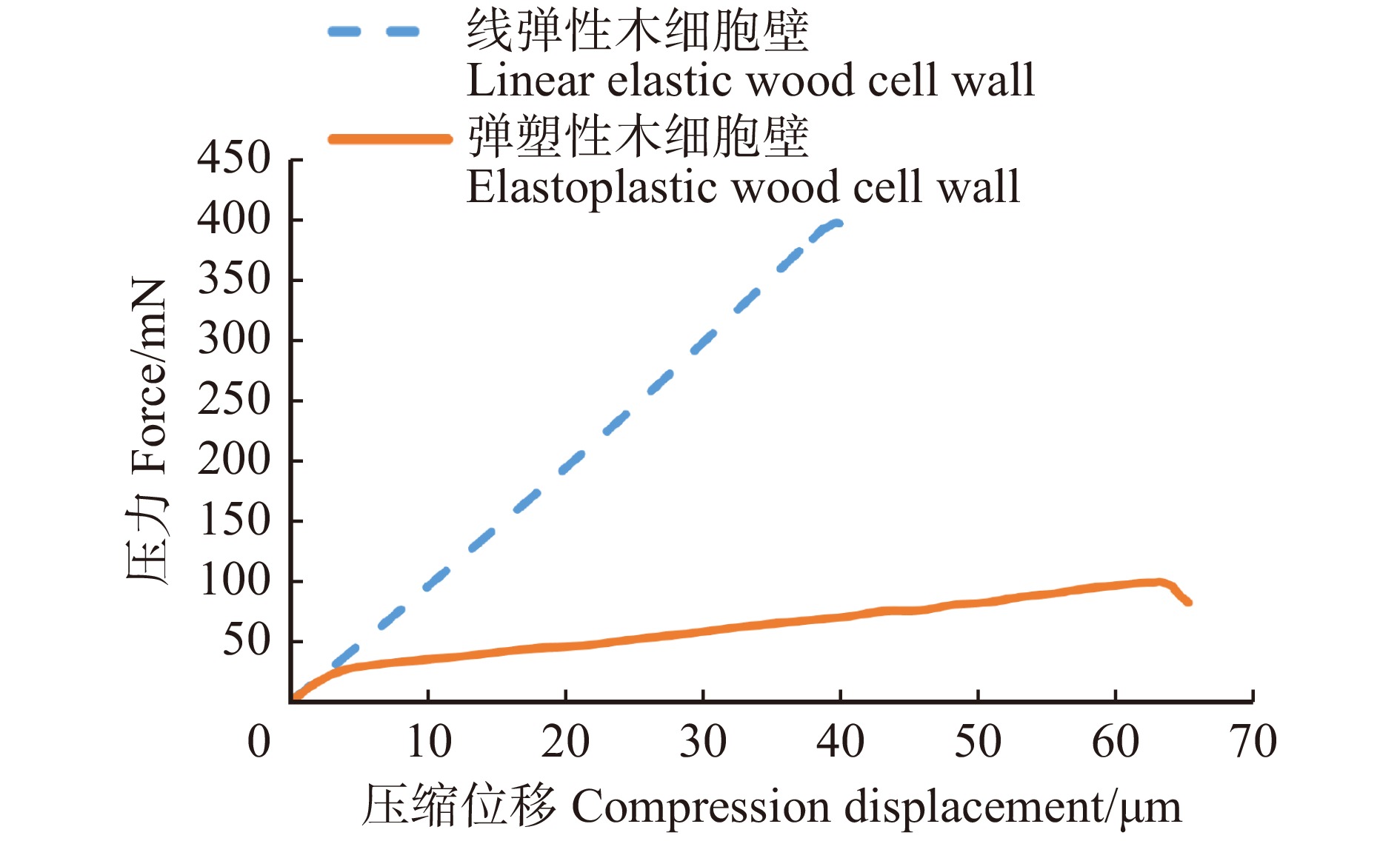
Department of Mechanics, School of Mechanical Engineering, Tianjin University, Tianjin 300354, China

摘要

摘要:
目的应压木细胞壁S₂层中的大微纤丝螺旋角(MFA)是树木管胞对力学环境适应性生长的结果，故它有其特别的力学性能。但目前人们还不了解S₂层中大MFA对其抗压性能的增韧机理。基于应压木细胞壁S₂层的超微结构建立复合材料力学模型，采用数值模拟方法研究应压木细胞壁S₂层中MFA对其抗压韧性的影响，可以探究其中的力学机理，并探索基于数值模型研究木细胞壁压缩韧性的建模与分析方法，进而为仿生材料设计奠定力学基础。
方法首先将云杉木细胞壁S₂层简化为连续微纤丝和基体组成的复合材料，并利用夹杂理论的自洽模型计算木细胞壁S₂层基体的等效弹性常数。然后利用HyperWorks建立木细胞壁的纤维增强复合材料有限元分析模型，用Abaqus模拟不同MFA的应压木和正常木细胞壁S₂层在压缩载荷下的力学行为，并用所得结果分析其MFA与抗压韧性的关系。在此基础上，对比是否考虑木细胞壁的S₁、S₃（或S₂L，指的是S₂层与S₁ 层之间木质素和半纤维素含量高的区域）和MP层（P和ML层）对其受压力学行为的影响，并分析在应压木细胞壁数值模型中考虑各组分材料塑性行为的重要性。
结果在压力作用下，当木细胞壁S₂层的MFA增大时，其临界屈曲位移增大，临界屈曲压力先减小再增大。45° MFA应压木细胞壁S₂层的临界压力与0° MFA正常木细胞壁S₂层相当，但前者的临界屈曲位移是后者的3.57倍，屈曲失稳前的应变能是后者的2.95倍。在相同压力下，45° MFA应压木细胞壁S₂层微纤丝的von Mises应力低于0° MFA正常木。由于单个应压木细胞壁S₂层中螺旋状微纤丝所具有的压−扭耦合变形受到周边管胞对扭转变形的约束，其抗压刚度和抗压韧性得到增强。应压木细胞壁中的S₁、S₂L和MP层对其受压屈曲有显著的约束作用，完整应压木细胞壁的临界压力比只考虑S₂层的临界压力增大37.6%。压力作用下木细胞壁的破坏模式为塑性屈曲，所以考虑木细胞壁各组分材料的塑性行为对准确地计算其抗压韧性十分重要，忽略其塑性行为会使其临界压力的计算结果增大2.97倍。
结论在受压状态下，应压木细胞壁S₂层中微纤丝的螺旋形貌改变了微纤丝与基体间的应力传递，使得S₂层的基体承受更多的压应力，木细胞壁的破坏模式变为局部塑性屈曲。所以在压力作用下，尽管应压木细胞壁的抗压刚度随S₂层MFA的增大而减小，但应压木细胞壁的临界屈曲位移随MFA的增大而显著地增大，从而增强了它的抗压韧性。当MFA为45°左右时，应压木细胞壁的抗压韧性最佳，此时不仅它的临界屈曲位移比正常木细胞壁S₂层高两倍多，且它的临界屈曲压力也略高于后者。
- 应压木 /
- 木细胞壁 /
- 微纤丝角（MFA） /
- 临界屈曲位移 /
- 抗压韧性 /
- 增韧机理 /
- 数值模拟
Abstract:
Objective The large microfibril helix angle (MFA) in the S₂ layer of the compression wood cell wall is the result of the adaptive growth of tree tracheids to the mechanical stimulation, so it has special mechanical functions. However, the toughening mechanism of large MFA in S₂ layer on the compressive properties of wood cell wall has not been understood yet by researchers. Based on a computational model of composite material for the ultrastructure of S₂ layer of the compression wood cell wall, the effects of MFA in S₂ layer on the compressive toughness of compression wood cell wall were simulated and the toughening mechanism was explored, and the method of modeling and analyzing the compressive toughness of the wood cell wall based on the numerical model was explored. The findings presented in this paper would provide useful guideline for the optimal design of biomimetic materials.
Method First, the S₂ layer of spruce wood cell wall was modeled as a composite cylinder composed of continuous microfibrils as well as matrix, and the equivalent elastic constants of the matrix of S₂ layer were calculated using the self-consistent model of inclusion theory. Then, the finite element analysis model of the fiber reinforced composite of wood cell wall was established by HyperWorks. The compressive mechanical behaviors of the S₂ layers of compression wood and normal wood with different MFA were simulated by Abaqus, and the relationship between MFA and the compressive toughness of S₂ layer was analyzed. On this basis, the compressive mechanical behaviors of wood cell wall with and without S₁, S₃ (or S₂L, it means the area between S₂ and S₁ layer with high lignin and hemicellulose content) and MP (P and ML) layers were investigated, and the importance of considering the plastic behavior of each constituent in the numerical model of compression wood cell wall was analyzed.
Result As the increasing of MFA in S₂ layer, the critical buckling displacement of S₂ layer of wood cell wall was increasing, and the critical buckling pressure was first decreasing and then increasing. The critical pressure of S₂ layer of compression wood cell wall with MFA of 45° was equivalent to that of normal wood cell wall with MFA = 0°, but the critical buckling displacement of the former was 3.57 times of the latter, and the strain energy before buckling was 2.95 times of the latter. Under the same pressure, the von Mises stress of microfibrils in the compression wood S₂ layer with MFA = 45° was lower than that in the normal wood S₂ layer with MFA = 0°. The compressive stiffness and compressive toughness of S₂ layer with large MFA were enhanced because the compression-torsion coupling of spiral microfibrils in S₂ layer of a single compression wood cell wall was constrained by its surrounding tracheids. S₁, S₂L and MP layers had significant restraint effect on the buckling of the compression wood cell wall, and the critical pressure of the compression wood cell wall with the consideration of all the layers in the wood cell wall was 37.6% larger than that of the model only considering the S₂ layer. The failure mode of wood cell wall under pressure was of plastic buckling, so it is very important to include the plastic behavior of each component of wood cell wall to accurately calculate its compressive toughness. Ignoring the plastic behavior of wood cell wall will cause the computed result of its critical pressure increased by 2.97 times.
Conclusion The spiral morphology of microfibrils in the S₂ layer of compression wood cell wall changes the stress transfer between the microfibrils and the matrix, which results in the matrix of the S₂ layer bearing more compressive stress and the failure mode of the wood cell wall becoming local plastic buckling. Although the compressive stiffness of compression wood cell wall decreases with the increase of MFA in S₂ layer, the critical buckling displacement of cell wall increases significantly with the increase of MFA, thereby the compressive toughness of S₂ layer is enhanced. When MFA is about 45°, the compressive toughness of S₂ layer of compression wood cell wall reaches the highest, not only its critical buckling displacement is more than twice that of S₂ layer of normal wood cell wall, but also its critical buckling pressure is slightly higher than the latter.
- compression wood /
- wood cell wall /
- microfibril angle (MFA) /
- critical buckling displacement /
- compressive toughness /
- toughening mechanism /
- numerical simulation

HTML全文

图 1 木细胞壁微结构

图片引自参考文献[20]和[21]。Pictures are cited from references [20] and [21].

Figure 1. Microstructure of wood cell wall

下载: 全尺寸图片幻灯片

图 2 正常木和应压木细胞壁几何模型

Figure 2. Geometric models of cell walls of normal and compression wood

下载: 全尺寸图片幻灯片

图 3 木细胞壁有限元网格

Figure 3. Finite element mesh of wood cell wall

下载: 全尺寸图片幻灯片

图 4 木细胞壁上端面载荷和外表面边界条件

Figure 4. Load on the upper face and boundary conditions on the outer surface of wood cell wall

下载: 全尺寸图片幻灯片

图 5 不同MFA木细胞壁S2层压力–位移曲线

Figure 5. Force-displacement curves of S2 layers of wood cell wall with different MFA

下载: 全尺寸图片幻灯片

图 6 0° MFA的正常木和45° MFA的应压木细胞壁S₂层压缩屈曲变形图

Figure 6. Compressive buckling deformation graphs of S₂ layers of normal wood cell wall at 0° MFA and compression wood cell wall at 45° MFA

下载: 全尺寸图片幻灯片

图 7 0° MFA正常木和45° MFA应压木细胞壁S₂层轴向压缩过程中的应变能

Figure 7. Strain energy during axial compression of S₂ layers of normal wood cell wall at 0° MFA and compression wood cell wall at 45° MFA

下载: 全尺寸图片幻灯片

图 8 0° MFA正常木和45° MFA应压木细胞壁S₂层微纤丝和基体von Mises应力分布云图

Figure 8. von Mises stress cloud diagrams of microfibrils and matrix of S₂ layers of normal wood cell wall at 0° MFA and compression wood cell wall at 45° MFA

下载: 全尺寸图片幻灯片

图 9 有无扭转约束的45° MFA应压木细胞壁S₂层压力–位移曲线

Figure 9. Force-displacement curves of S₂ layers of compression wood cell walls with and without torsional restraint at 45° MFA

下载: 全尺寸图片幻灯片

图 10 不同长径比的45° MFA应压木细胞壁S₂层应力–应变曲线

Figure 10. Stress-strain curves of S₂ layers of compression wood cell walls with different aspect ratios at 45° MFA

下载: 全尺寸图片幻灯片

图 11 不同水体积分数的45° MFA应压木细胞壁S₂层压力–位移曲线

Figure 11. Force-displacement curves of S₂ layers of compression wood cell walls with different water volume ratio at 45° MFA

下载: 全尺寸图片幻灯片

图 12 考虑所有层和只考虑S₂层的45°应压木细胞壁压力–位移曲线

Figure 12. Force-displacement curves of compression wood cell walls considering all layers and considering only S₂ layer at MFA 45°

下载: 全尺寸图片幻灯片

图 13 线弹性和弹塑性45°MFA应压木细胞壁压力–位移曲线

Figure 13. Force-displacement curves of compression wood cell walls at 45° MFA using linear elastic and elastoplastic models

下载: 全尺寸图片幻灯片

表 1 云杉木细胞壁各层尺寸

Table 1 Dimensions of layers in spruce wood cell wall

细胞壁层 Cell wall layer	厚度 Thickness/µm	占细胞壁厚度比例 Proportion to cell wall thickness/%
S₁	0.318	9.0
S₂	3.000	85.0
S₃	0.112	3.2
P	0.100	2.8

下载: 导出CSV

表 2 S₂层基体成分材料的力学参数及体积分数

Table 2 Mechanical parameters of matrix component materials of S₂ layer

成分 Component	体积模量 Bulk modulus/GPa	剪切模量 Shear modulus/GPa	在S₂层中体积分数 Volume fraction in S₂ layer/%
半纤维素 Hemicellulose	8.89	2.96	17.0
木质素 Lignin	5.00	2.30	28.0
水和提取物 Water and extract	2.30	0	14.0

下载: 导出CSV

表 3 微纤丝弹性常数

Table 3 Elastic constants of microfibril

部分 Component	${E}_{\mathrm{A}}$ /GPa	${E}_{\mathrm{T}}$ /GPa	${\mu }_{\mathrm{A}}$	${\mu }_{\mathrm{T}}$	${G}_{\mathrm{A}}$ /GPa
微纤丝 Microfibril	62.77	15.00	0.087	0.420	3.00
注： ${E}_{\mathrm{A}}$ 、 ${\mu }_{\mathrm{A}}$ 和 ${E}_{\mathrm{T}}\mathrm{、}{\mu }_{\mathrm{T}}$ 分别表示微纤丝轴向和横向的弹性模量、泊松比， ${G}_{\mathrm{A}}$ 为剪切模量。Notes: ${E}_{\mathrm{A}}$ , ${\mu }_{\mathrm{A}}$ and ${E}_{\mathrm{T}}, \;{\mu }_{\mathrm{T}}$ represent the axial and lateral elastic modulus and Poisson’s ratio of the microfibril, ${G}_{\mathrm{A}}$ is the shear modulus.

下载: 导出CSV

表 4 S₁、S₃、P和ML层的弹性常数

Table 4 Elastic constants of S₁, S₃, P and ML layers

细胞壁层 Cell wall layer	${E}_{\mathrm{c}}$ /GPa	${E}_{\mathrm{r}}$ /GPa	${\mu }_{\mathrm{c}}$	${\mu }_{\mathrm{r}}$	${G}_{\mathrm{c}}$ /GPa
S₁	25.64	8.74	0.226	0.035	2.88
S₃	23.88	8.11	0.232	0.036	2.70
ML + P	11.51	11.51	0.200	0.200	4.80
注：下标c和r分别表示木细胞壁的周向和径向。Note: subscripts c and r indicate the circumferential and radial directions of the wood cell wall.

下载: 导出CSV

表 5 微纤丝和基体塑性材料参数

Table 5 Plastic material parameters of microfibril and matrix

部分 Component	屈服应力 Yield stress/GPa	极限压应力 Ultimate compressive stress/GPa	极限塑性应变 Ultimate plastic strain
基体 Matrix	0.054	0.243	0.770
微纤丝 Microfibril	0.318	0.636	0.107

下载: 导出CSV

参考文献(32)

[1]	张胜龙, 刘京晶, 楼雄珍, 等. 杉木应压木木质部细胞形态特征及主要代谢成分表征[J]. 北京林业大学学报, 2015, 37(5): 126−133. doi: 10.13332/j.1000-1522.20140396 Zhang S L, Liu J J, Lou X Z, et al. Morphological characteristics of cells and main metabolic components in xylem of Cunninghamia lanceolata compression wood[J]. Journal of Beijing Forestry University, 2015, 37(5): 126−133. doi: 10.13332/j.1000-1522.20140396
[2]	Färber J, Lichtenegger H C, Reiterer A, et al. Cellulose microfibril angles in a spruce branch and mechanical implications[J]. Journal of Materials Science, 2001, 36(21): 5087−5092. doi: 10.1023/A:1012465005607
[3]	杜明秋, 钟珊丽, 林二培, 等. 杉木应压木形成中的显微特征及主要代谢成分变化[J]. 核农学报, 2022, 36(11): 2307−2315. doi: 10.11869/j.issn.100-8551.2022.11.2307 Du M Q, Zhong S L, Lin E P, et al. Anatomy characteristics and study of alterations of key metabolic components in Cunninghamia lanceolata during compression wood formationon wood formation[J]. Journal of Nuclear Agricultural Sciences, 2022, 36(11): 2307−2315. doi: 10.11869/j.issn.100-8551.2022.11.2307
[4]	Ruelle J. Morphology, anatomy and ultrastructure of reaction wood[M]. Berlin: Springer Berlin Heidelberg, 2013: 13−35.
[5]	李柬龙, 陈胜, 李海潮, 等. 轻木细胞壁超微结构与力学性能关系研究[J]. 北京林业大学学报, 2022, 44(2): 115−122. Li J L, Chen S, Li H C, et al. Relationship between cell wall ultrastructure and mechanical properties of balsa wood[J]. Journal of Beijing Forestry University, 2022, 44(2): 115−122.
[6]	Adusumalli R, Raghavan R, Ghisleni R, et al. Deformation and failure mechanism of secondary cell wall in spruce late wood[J]. Applied Physics A, 2010, 100(2): 447−452. doi: 10.1007/s00339-010-5847-1
[7]	张淑琴, 余雁, 费本华, 等. 杉木木材管胞纵向弹性模量的研究[J]. 北京林业大学学报, 2012, 34(6): 126−130. Zhang S Q, Yu Y, Fei B H, et al. Longitudinal modulus of elasticity of Chinese fir tracheids[J]. Journal of Beijing Forestry University, 2012, 34(6): 126−130.
[8]	余雁. 人工林杉木管胞的纵向力学性质及其主要影响因子研究[D]. 北京: 中国林业科学研究院, 2003. Yu Y. Longitudinal mechanical properties and its main influencing factors of tracheids of Chinese fir from plantation[D]. Beijing: Chinese Academy of Forestry, 2003.
[9]	孙海燕, 苏明垒, 吕建雄, 等. 细胞壁微纤丝角和结晶区对木材物理力学性能影响研究进展[J]. 西北农林科技大学学报(自然科学版), 2019, 47(5): 50−58. Sun H Y, Su M L, Lü J X, et al. Research progress on effect of microfibril angle and crystalline area in cell wall on wood physical and mechanical properties[J]. Journal of Northwest A&F University (Natural Science Edition), 2019, 47(5): 50−58.
[10]	Barrios A, Trincado G, Watt M S. Wood properties of juvenile and mature wood of Pinus radiata Don trees growing on contrasting sites in Chile[J]. Forest Science, 2017, 63(2): 184−191. doi: 10.5849/forsci.2016-060
[11]	蒋坤云, 陈丽华, 杨苑君, 等. 华北油松、落叶松根系抗拉强度与其微观结构的相关性研究[J]. 水土保持学报, 2013, 27(2): 8−12. doi: 10.13870/j.cnki.stbcxb.2013.02.053 Jiang K Y, Chen L H, Yang Y J, et al. Relationship between tensile strength and selected anatomical features of two different conifer species’ roots in North China[J]. Journal of Soil and Water Conservation, 2013, 27(2): 8−12. doi: 10.13870/j.cnki.stbcxb.2013.02.053
[12]	李新宇, 张明辉. 利用X射线衍射法探究木材含水率与结晶度的关系[J]. 东北林业大学学报, 2014, 42(2): 96−99. doi: 10.13759/j.cnki.dlxb.2014.02.023 Li X Y, Zang M H. Relationship of wood moisture content and the degree of crystallinity by X-Ray diffraction[J]. Journal of Northeast Forestry University, 2014, 42(2): 96−99. doi: 10.13759/j.cnki.dlxb.2014.02.023
[13]	Schwiedrzik J, Raghavan R, Rüggeberg M, et al. Identification of polymer matrix yield stress in the wood cell wall based on micropillar compression and micromechanical modelling[J]. Philosophical Magazine (Abingdon, England), 2016, 96(32-34): 3461−3478. doi: 10.1080/14786435.2016.1235292
[14]	赵彻. 异质材料与微结构耦合仿生设计及其3D打印[D]. 长春: 吉林大学, 2017. Zhao C. Biomimetic design and 3D printing of composite by coupling heterogeneous materials and microstructures[D]. Changchun: Jilin University, 2017.
[15]	Li X W, Yu H C, Qing H Q, et al. Helical fiber pull-out in biological materials[J]. Acta Mechanica Solida Sinica, 2016, 29(3): 245−256. doi: 10.1016/S0894-9166(16)30159-8
[16]	Gao Y, Li B, Wang J, et al. Fracture toughness analysis of helical fiber-reinforced biocomposites[J]. Journal of the Mechanics and Physics of Solids, 2021, 146: 104206. doi: 10.1016/j.jmps.2020.104206
[17]	Zhong W, Zhang Z, Chen X, et al. Multi-scale finite element simulation on large deformation behavior of wood under axial and transverse compression conditions[J]. Acta Mechanica Sinica, 2021, 37(7): 1136−1151. doi: 10.1007/s10409-021-01112-z
[18]	Oliveira P R, Ribeiro F S L M, Panzera T H, et al. Hybrid polymer composites made of sugarcane bagasse fibres and disposed rubber particles[J]. Polymers and Polymer Composites, 2021, 29(9): S1280−S1293.
[19]	苏骏, 钱维民, 郭锋, 等. 超低温对超高韧性水泥基复合材料抗压韧性影响试验[J]. 复合材料学报, 2021, 38(12): 4325−4336. doi: 10.13801/j.cnki.fhclxb.20210223.002 Su J, Qian W M, Guo F, et al. Experimental study on the influence of ultra-low temperature on compressive toughness of ultra high toughness cementitious composites[J]. Acta Materiae Compositae Sinica, 2021, 38(12): 4325−4336. doi: 10.13801/j.cnki.fhclxb.20210223.002
[20]	Deng Q, Li S, Chen Y. Mechanical properties and failure mechanism of wood cell wall layers[J]. Computational Materials Science, 2012, 62: 221−226. doi: 10.1016/j.commatsci.2012.05.050
[21]	Hofstetter K, Hellmich C, Eberhardsteiner J. Development and experimental validation of a continuum micromechanics model for the elasticity of wood[J]. European Journal of Mechanics-A/Solids, 2005, 24(6): 1030−1053. doi: 10.1016/j.euromechsol.2005.05.006
[22]	Salmén L. Micromechanical understanding of the cell-wall structure[J]. Comptes Rendus Biologies, 2004, 327(9−10): 873−880. doi: 10.1016/j.crvi.2004.03.010
[23]	刘宇. 基于木材的高强纤维素材料的构建与力学性能的研究[D]. 广州: 华南理工大学, 2019. Liu Y. Fabrication of super strong cellulose based materials from wood and their mechanical properties[D]. Guangzhou: South China University of Technology, 2019.
[24]	Marklund E, Varna J. Micromechanical modelling of wood fibre composites[J]. Plastics, Rubber & Composites, 2009, 38(2−4): 118−123.
[25]	Qing H, Mishnaevsky L. 3D multiscale micromechanical model of wood: from annual rings to microfibrils[J]. International Journal of Solids and Structures, 2010, 47(9): 1253−1267. doi: 10.1016/j.ijsolstr.2010.01.014
[26]	Jin K, Qin Z, Buehler M J. Molecular deformation mechanisms of the wood cell wall material[J]. Journal of the Mechanical Behavior of Biomedical Materials, 2015, 42: 198−206. doi: 10.1016/j.jmbbm.2014.11.010
[27]	Gangwar T, Schillinger D. Microimaging-informed continuum micromechanics accurately predicts macroscopic stiffness and strength properties of hierarchical plant culm materials[J]. Mechanics of Materials, 2019, 130: 39−57. doi: 10.1016/j.mechmat.2019.01.009
[28]	Hofstetter K, Hellmich C, Eberhardsteiner J. Micromechanical modeling of solid-type and plate-type deformation patterns within softwood materials: a review and an improved approach[J]. Holzforschung, 2007, 61(4): 343−351. doi: 10.1515/HF.2007.058
[29]	沈观林, 胡更开, 刘彬. 复合材料力学[M]. 北京: 清华大学出版社, 2013. Shen G L, Hu G K, Liu B. Mechanics of composite materials[M]. Beijing: Tsinghua University Press, 2013.
[30]	Fei G, Clemens M A, Michael C J. Thickness-dependent stiffness of wood: potential mechanisms and implications[J]. Holzforschung, 2020, 74(12): 1079−1087. doi: 10.1515/hf-2019-0311
[31]	Horbelt N, Dunlop J W C, Bertinetti L, et al. Effects of moisture and cellulose fibril angle on the tensile properties of native single Norway spruce wood fibres[J]. Wood Science and Technology, 2021, 55(5): 1305−1318. doi: 10.1007/s00226-021-01315-4
[32]	Ji Z, Ma J, Zhang Z, et al. Distribution of lignin and cellulose in compression wood tracheids of Pinus yunnanensis determined by fluorescence microscopy and confocal Raman microscopy[J]. Industrial Crops and Products, 2013, 47: 212−217. doi: 10.1016/j.indcrop.2013.03.006

施引文献(6)

期刊类型引用(4)

1.	张苗苗，罗于洋，王树森，张丽娜，马成功，于胜利，王景圆. 内蒙古旺业甸华北落叶松人工林空间结构分析及其优化. 西北林学院学报. 2024(01): 81-87+107 . 百度学术
2.	荆媛，魏爽，史文辉，马梓贺，王德宇，戎可. 天然次生林中小斑啄木鸟的取食偏好. 野生动物学报. 2024(01): 84-94 . 百度学术
3.	孙宇，刘盛，田佳歆，程福山，赵士博，王诗俊. 基于空间结构优化的长白落叶松人工林分间伐模型构建. 中南林业科技大学学报. 2023(01): 72-83 . 百度学术
4.	刘鑫，黄浪，卿东升，李建军. 基于Voronoi空间单元的林分空间结构智能优化研究. 林业资源管理. 2023(04): 27-35 . 百度学术

其他类型引用(2)

资源附件(0)

图(13) / 表(5)

计量

文章访问数: 516
HTML全文浏览量: 214
PDF下载量: 92
被引次数: 6

应压木细胞壁S2层的微纤丝螺旋角对其抗压韧性的影响

作者简介: 刘浩正。 主要研究方向：生物力学。 Email：ysulhz@163.com 地址： 300354天津市津南区雅观路135号天津大学机械工程学院力学系

责任作者: 石广玉，教授，博士生导师。主要研究方向：固体力学和复合材料力学。Email：shi_guangyu@tju.edu.cn 地址： 同上

计量

出版历程

Effects of microfibril helix angle in the S2 layer of compression wood cell wall on the compressive toughness of it

期刊类型引用(4)

其他类型引用(2)

计量

出版历程

目录

应压木细胞壁S₂层的微纤丝螺旋角对其抗压韧性的影响

作者简介:
刘浩正。主要研究方向：生物力学。 Email：ysulhz@163.com　地址： 300354天津市津南区雅观路135号天津大学机械工程学院力学系

责任作者:
石广玉，教授，博士生导师。主要研究方向：固体力学和复合材料力学。Email：shi_guangyu@tju.edu.cn　地址：同上

Effects of microfibril helix angle in the S₂ layer of compression wood cell wall on the compressive toughness of it