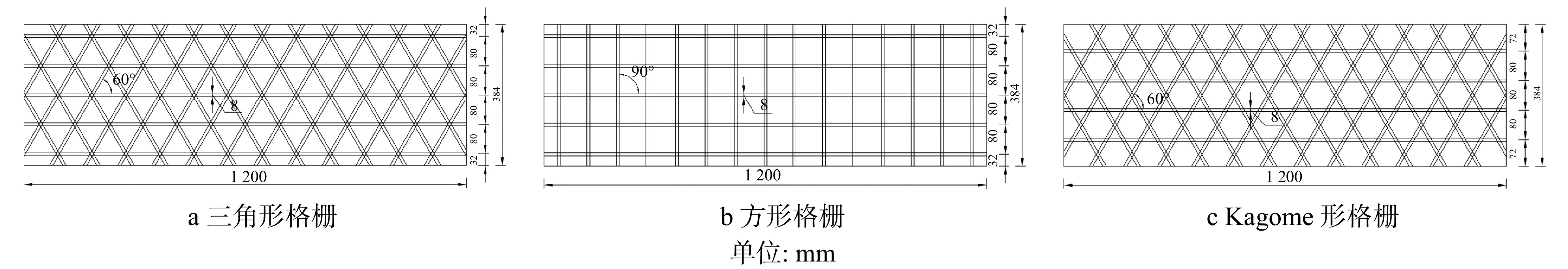
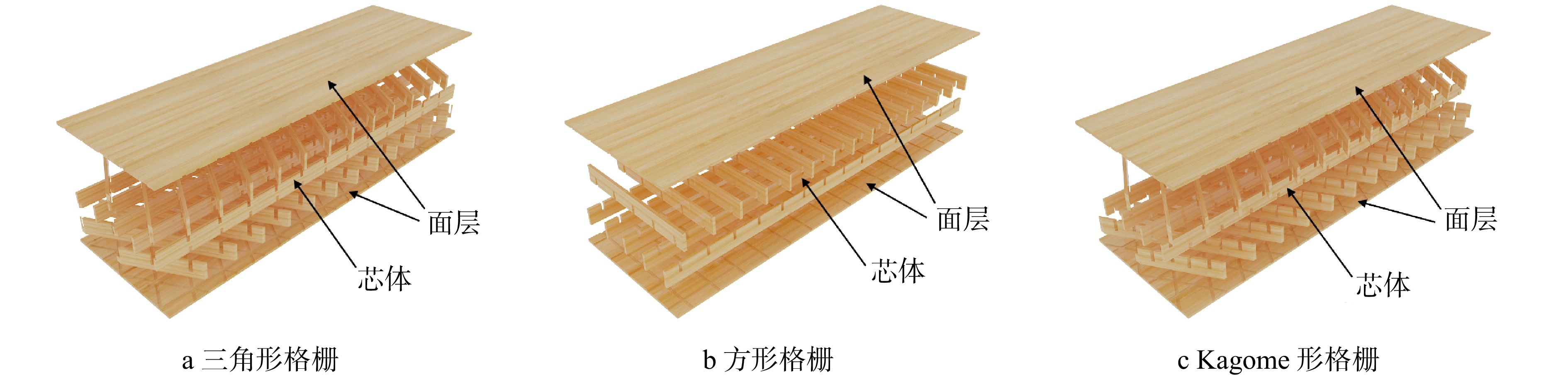
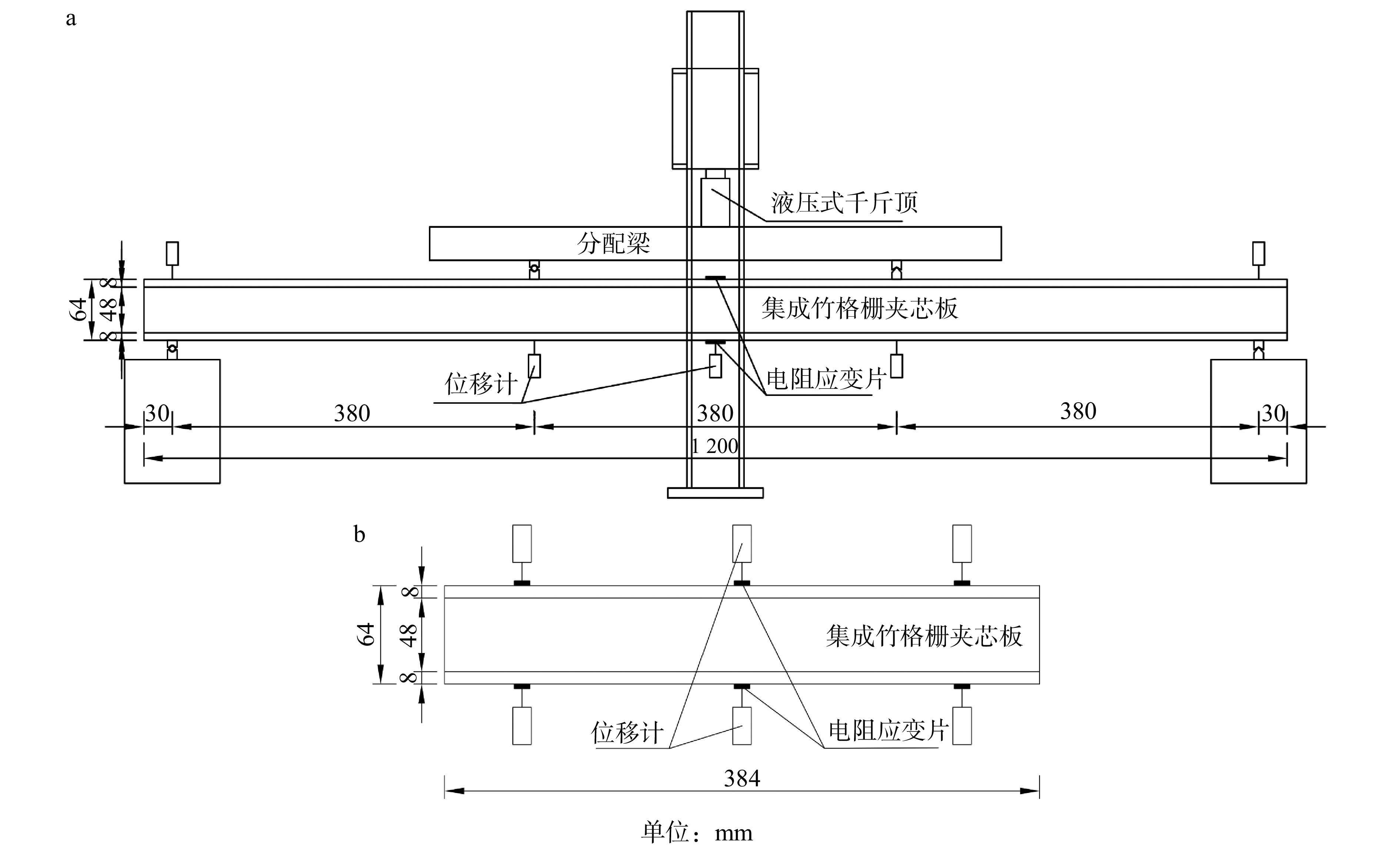
Bending performance of laminated bamboo sandwich panels with different lattice configurations
-
摘要:目的
针对集成竹利用效率不高，使用形式较为单一的问题，探索其轻质、高效特性的先进结构形式，这对推动竹材在工程领域的应用方面具有重要意义。本研究旨在探究不同构型格栅芯体对集成竹格栅夹芯板弯曲性能的影响，选出不同格栅构型中抗弯性能最优的结构，为其在实际工程的应用提供理论依据。
方法以集成竹为原材料，通过嵌锁法分别设计加工3种具有不同构型（三角形格栅、方形格栅和Kagome形格栅）的集成竹格栅夹芯板。对不同格栅芯体的集成竹格栅夹芯板进行四点弯曲试验，探讨夹芯板结构的受弯性能，分析夹芯板结构在受弯荷载下的破坏机理、跨中挠度、抗弯刚度和极限承载力的变化规律，并对3种不同芯体集成竹格栅夹芯板结构的比强度和比刚度进行对比。同时，采用有限元方法建立集成竹格栅夹芯板四点弯曲试验模型，并进行数值模拟分析。
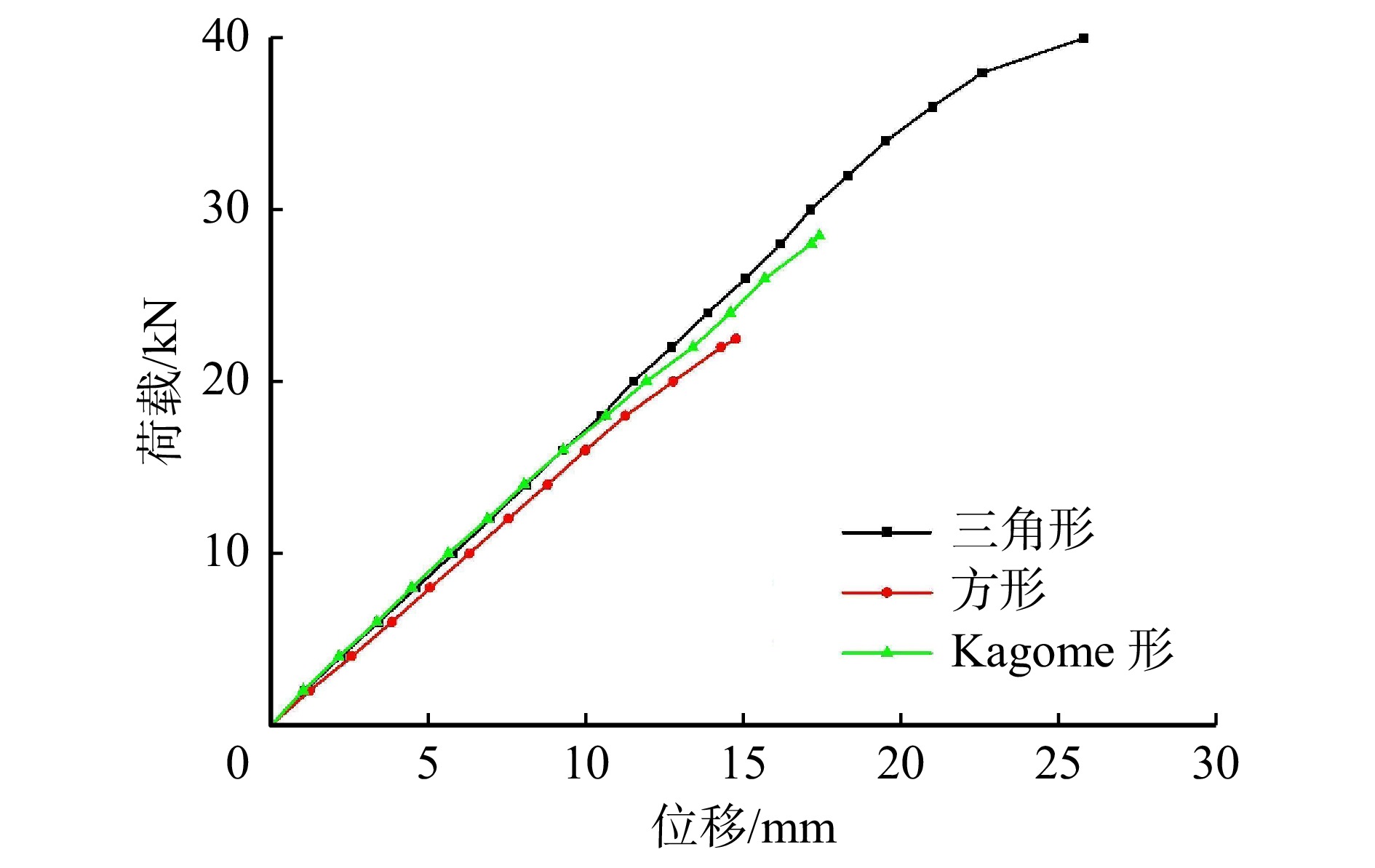
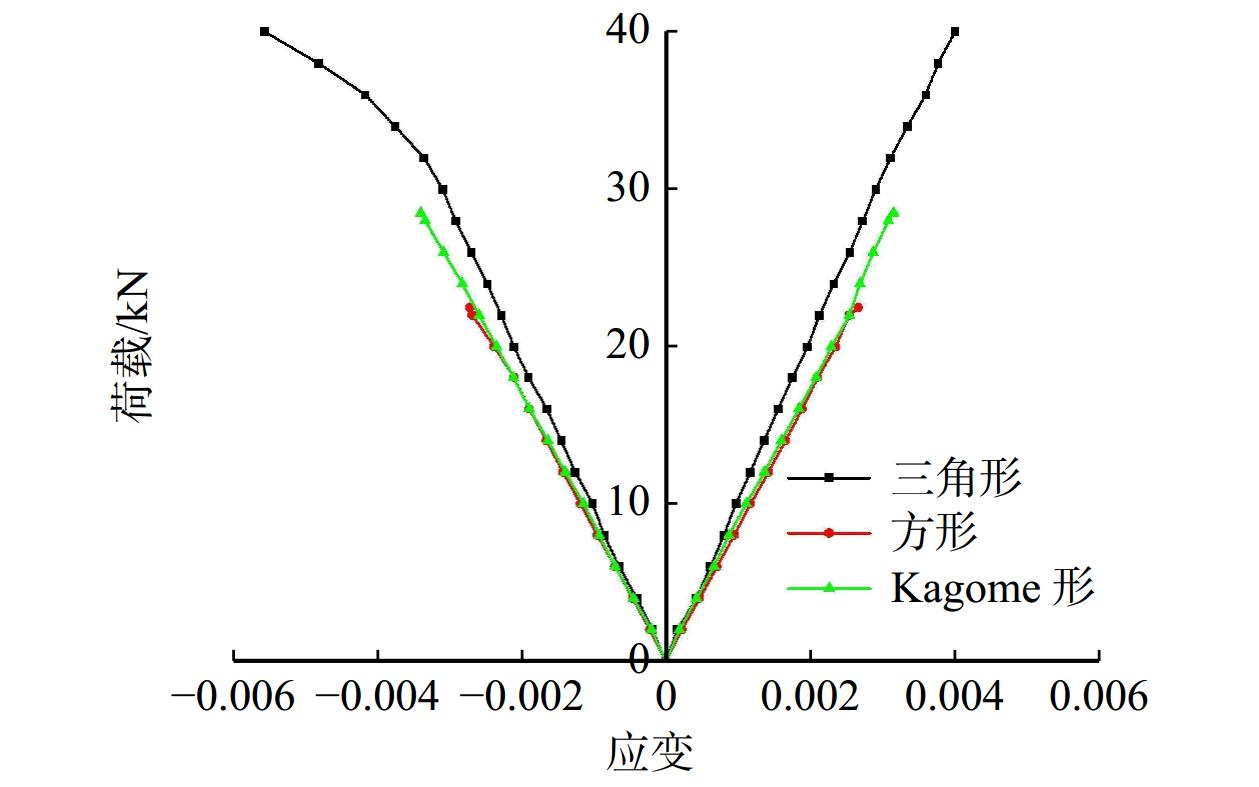
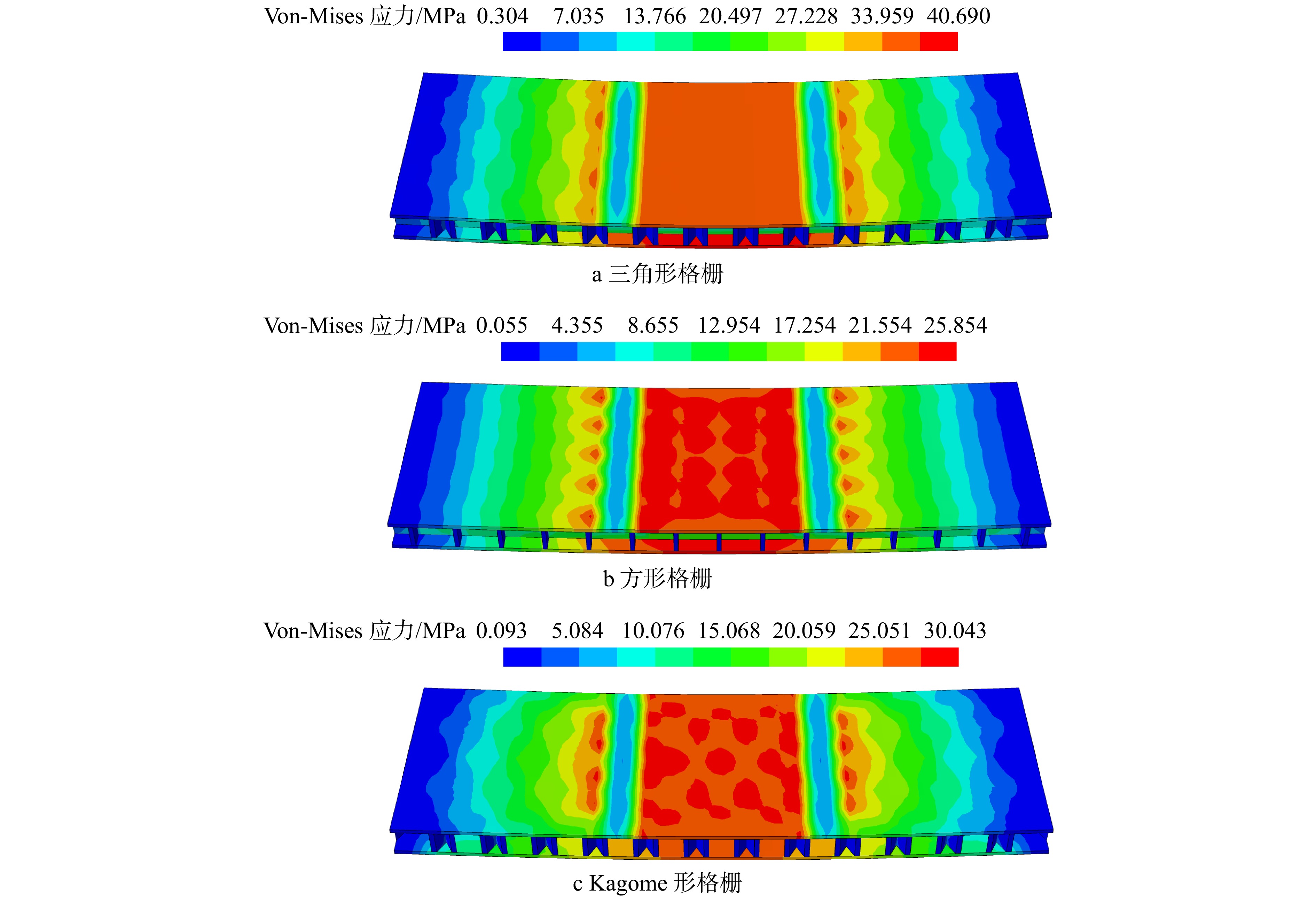
结果3种不同构型格栅芯体的集成竹格栅夹芯板结构在受弯加载过程中均表现为剪切破坏。三角形集成竹格栅夹芯板的承载能力最好，三角形和Kagome形格栅极限承载力分别为38.7 和27.5 kN，较极限承载力22.5 kN的方形格栅增加了71.9%和22.2%；同时三角形集成竹格栅夹芯板的比强度为133.5 kN·m/kg，较方形（94.6 kN·m/kg）和Kagome形（96.7 kN·m/kg）分别提高了41.2%和38.1%。有限元模型的模拟结果与试验结果较一致，能有效预测集成竹格栅夹芯板的弯曲性能。
结论3种格栅构型中三角形集成竹夹芯板弯曲性能最优，能够更好地发挥集成竹轻质高强的性能优势。研究结果可为竹材在工程领域的应用提供一种高效的结构形式及理论依据。
Abstract:ObjectiveIntegrated bamboo has low utilization efficiency and is used in a rather limited variety of forms in engineering applications. So exploring advanced structural forms that integrate the lightweight and high-efficiency characteristics of bamboo is of great significance in promoting the application of bamboo in the field of engineering. This study aims to investigate the impact of different lattice configurations on bending performance of laminated bamboo sandwich panels, select the structure with the best bending resistance among various lattice configurations, and provide a theoretical basis for its application in practical engineering.
MethodThree kinds of laminated bamboo sandwich panels with different lattice cores, namely triangular lattice, square lattice and Kagome lattice, were designed and processed with laminated bamboo as raw material by interlocking method in this study. A four-point bending test was carried out on laminated bamboo sandwich panels with different lattice cores, and the bending performance of sandwich panels was discussed. The failure mechanism of sandwich panels under bending load, as well as the variation law of mid-span deflection, bending stiffness and ultimate bearing capacity, were analyzed, and the specific strength and specific stiffness of laminated bamboo sandwich panels with three different core layers were compared. At the same time, a four-point bending test model of laminated bamboo sandwich panels was established by finite element method, and numerical simulation was carried out.
ResultThree kinds of sandwich panels with different lattice cores all showed shear failure during the bending loading, among which the triangular lattice sandwich panel had the best bearing capacity. Compared with square lattice sandwich panel with ultimate bearing capacity of 22.5 kN, the ultimate bearing capacity of sandwich panels with triangular lattice and Kagome lattice was 38.7 and 27.5 kN, increased by 71.9% and 22.2%, respectively. The specific strength of laminated bamboo sandwich panel with triangular lattice was 133.5 kN·m/kg, which was 41.2% and 38.1% higher than that of square lattice and Kagome lattice with specific strength of 94.6 and 96.7 kN·m/kg, respectively. The finite element model was in good agreement with the test results, which can effectively predict bending performance of laminated bamboo sandwich panels.
ConclusionAmong three kinds of lattice cores, the laminated bamboo triangular lattice sandwich panel has the best bending performance, which can better present the lightweight and high-strength advantages of laminated bamboo. The research results can provide an effective structural form and theoretical basis for the application of bamboo in the engineering field.
-
扦插繁殖是一种简便高效的林木种质资源保存方法。其操作简便、周期短、繁殖效率高，能够在林木繁殖过程中高度遗传并保持母株的优良特性，因此被广泛用于优质林木遗传资源（如珍稀长寿基因和抗逆基因）的保存[1−2]。目前，林木扦插生根机理的研究侧重于生理、生化等方面，揭示了插穗的营养水平、酶活性、内源激素变化等与生根的关系[3−4]。然而，对于抑制林木扦插生根的物质研究还鲜有报道。
扦插生根抑制物是指在植物扦插繁殖过程中，阻碍或抑制插穗根系形成和生长的化学物质，如激素（脱落酸、乙烯等）、代谢物（酚酸、类黄酮和生长抑制剂等）[5]。林木插穗中的抑制物含量受树种和树龄的影响[6]，如樱桃（Prunus spp.）插穗中的酚类物质（芦丁、香草酸、表儿茶素、咖啡酸和芥子酸）含量较高，导致扦插生根率低[7]。在巨桉（Eucalyptus grandis）插穗中发现了3种与单元酚相近的生根抑制物[8]。在落叶松（Larix kaempferi）扦插过程中，邻苯二酚、对羟基苯甲酸、儿茶酸、阿魏酸和没食子酸含量在难生根的无性系中均高于易生根无性系，且在扦插过程中逐渐减少，表明这些物质在生根过程中具有抑制作用[9]。
树龄的增长会导致抑制物含量的增加，这也是影响林木扦插生根能力的重要因素之一。如苯酚类和类黄酮含量随马尾松（Pinus massoniana）、紫杉（Taxus cuspidata）、核桃楸（Juglans mandshuric）树龄增长而逐渐积累，从而对扦插生根产生抑制作用[10−12]。有研究表明，通过使用酒精、高锰酸钾、硝酸银和抗酚剂等溶液处理，以及机械处理，可以去除插穗中的部分抑制物，从而提高其生根率[13−15]。
古树具有较强的气候和土壤适应能力，是林木用材、困难立地造林和园林美化等方面的优良种质资源[1−2]。侧柏（Platycladus orientalis）是我国重要的长寿命树种之一，在陕西、河南和山西等地分布着寿命长达数百年甚至数千年的侧柏古树[16−17]。然而，侧柏古树扦插繁殖中存在生根时间长、生根率低的问题[4]，这严重限制了古树的繁殖效率和数量，不利于古树优良基因保存和生态功能的发挥。目前，针对侧柏古树扦插过程中类黄酮和酚酸物质含量及其影响的研究仍较少。本文在3月份和6月份选取树龄为5、100、300和700 年生的侧柏母树的插穗进行扦插，研究不同树龄、不同扦插季节以及扦插过程中类黄酮和酚酸物质对侧柏生根的影响，为提高林木扦插生根率和保留古树优良种质资源提供理论依据。
1. 材料与方法
1.1 试验材料
选取中国林业科学研究院和北京植物园内树龄约为5、100、300和700年生（根据古树树龄记载）的侧柏母树，其中以5 年生侧柏作为对照试验材料。在3月份、6月份分别采集各林龄生长健壮、无病虫害的新梢。不同部位插穗试验采取5、100、300和700 年生侧柏古树的东、南、西、北的上和下8个方位的枝条，截成10 ~ 15 cm长的插穗，在离芽1.0 ~ 1.5 cm处的基部斜切。每个处理25个插穗，3次重复。插穗用质量浓度为1 000 mg/L的溶液（mNAA∶mIBA = 1∶1）浸泡处理1 min。使用轻基质网袋容器，基质选用体积比为2∶8的泥炭和珍珠岩的混合物。扦插试验在中国林业科学研究院内的全光照喷雾插床中进行，管理参照杜常健等[18]研究。
根据前期实验观察到的扦插过程中插穗基部的形态变化，本文将5、100、300和700年生侧柏母树的插穗中的3个不定根形成过程进行设定，扦插试验当天设为第0 天（S1）、愈伤组织形成期设为第45天（S2）和不定根形成期设为第90天（S3）。对每个重复随机选取插穗进行样本取材。拔出插穗后，用蒸馏水冲洗干净，滤纸擦干，迅速剥取插穗基部0.5 cm（除去木质部），剪碎混匀后保存。
1.2 试验方法
1.2.1 不同季节的不同树龄侧柏插穗的浸提液对白菜籽发芽的影响
在3月份、6月份分别取5、100、300和700年生侧柏的插穗。在S1阶段时采取除木质部外的组织0.2 g，液氮研磨成粉末，放入6 mL的80%甲醇提取液中，用超声波浸提2 h。将所得浸提液定容至3 mL，然后稀释至浸提液原浓度的0%（CK）、25%、50%、100%，分别导入培养皿，待溶液蒸干后加入2 mL蒸馏水，每个处理重复3次。在每个培养皿中放置50粒白菜种子，置于25 ℃光照培养箱中进行培养和观察，3 d后统计发芽率。
1.2.2 树龄对侧柏扦插生根的影响
在6月份分别开展5、100、300和700年生侧柏母树扦插试验，3个月后统计生根率和生根数，分别取平均值。
1.2.3 内源生根抑制物的测定
采用高效液相色谱法（HPLC）（Agilent 1100，美国Agilent公司）测定内源生根抑制物，包括类黄酮（芦丁和槲皮素）和酚酸物质（水杨酸、香豆酸、苯酚、阿魏酸、没食子酸、邻苯二酚）。
1.2.4 不同种类酚酸、类黄酮物质含量在不同季节的变化
在3月份和6月份，以母树树龄为5、100、300和700年生侧柏在S1阶段时插穗为试验材料，测定类黄酮（芦丁和槲皮素）和酚酸物质（水杨酸、香豆酸、苯酚、阿魏酸、没食子酸、邻苯二酚）的含量。
1.2.5 扦插过程中不同种类酚酸、类黄酮的含量的变化
在6月份进行扦插后，以母树树龄为5、100、300和700年生侧柏在S1、S2和S3阶段时插穗为试验材料，测定类黄酮（芦丁和槲皮素）和酚酸物质（水杨酸、香豆酸、苯酚、阿魏酸、没食子酸、邻苯二酚）的含量。
1.2.6 清除内源生根抑制物对扦插生根的影响
在6月份，选取100年生的侧柏插穗为试验材料，分别用0.1%硝酸银、0.1%醋酸、2%乙醇溶液进行20 h处理，分别用温水、洗洁精溶液处理3 h，用0.05%高锰酸钾溶液处理15 min。每个处理设25个插穗、3次重复。其他步骤同扦插试验。
2. 结果与分析
2.1 树龄对侧柏扦插生根的影响
本文研究了树龄对侧柏扦插生根率和生根数的影响。结果表明在6月份的扦插试验中，5年生侧柏扦插的生根率和生根数分别为83.5%和6.12；100、300和700年生侧柏扦插生根率分别为19.31%、13.54%和7.78%，生根数分别为3.21、2.84和2.24（图1）。5年生侧柏扦插的生根率和生根数显著高于100、300和700年生（P < 0.05）。随着树龄增加，生根能力显著下降，而100、300和700年生的扦插生根率和生根数没有显著差异（P > 0.05）。
![]()
图 1 树龄对侧柏扦插生根的影响不同小写字母代表处理之间在0.05水平存在显著性差异。下同。Different lowercase letters in the same column indicate significant differences at 0.05 level. Same as below.Figure 1. Effects of tree age on rooting of Platycladus orientalis cuttings2.2 不同季节和不同树龄侧柏插穗的浸提液对白菜籽发芽的影响
进一步探讨不同树龄和季节侧柏插穗浸提物对扦插生根的影响，研究结果表明除了CK外，3月份和6月份的侧柏插穗浸提液随着浓度的增加，白菜种子的发芽率均呈下降趋势。同时，随树龄增长，发芽率总体上呈现降低的趋势。尤其是浸提液占比为25%和50%时，来自300和700年生侧柏插穗的浸提液处理过的白菜籽的发芽率显著低于5年生侧柏插穗的浸提液处理过的白菜籽（P < 0.05）（表1，2）。总体来看，6月份的白菜籽的发芽率高于3月份，因此在6月份对侧柏古树进行扦插能够取得较好的效果。
表 1 3月份不同树龄侧柏不同插穗浸提液占比对白菜籽发芽率的影响Table 1. Effects of extraction solution with different proportions from Platycladus orientalis cuttings of different ages on germination rates of cabbage seeds in March% 树龄/a
Tree age/year0（CK） 25% 50% 100% 5 96.67 ± 1.33a 86.63 ± 7.05a 82.69 ± 6.37a 76.67 ± 6.66a 100 96.67 ± 1.35a 71.33 ± 7.23ab 70.00 ± 7.34ab 61.00 ± 7.22b 300 96.67 ± 1.38a 70.67 ± 6.87b 61.33 ± 6.59b 60.67 ± 6.13b 700 96.67 ± 1.33a 66.67 ± 7.15b 62.67 ± 6.78b 58.67 ± 5.93b 注：不同小写字母表示同一浸提液占比不同年龄间发芽率存在显著差异（P < 0.05）。下同。Notes:different lowercase letters indicate significant differences at the 0.05 level between cuttings of different ages under the same proportion of extraction solution. The same below. 表 2 6月份不同树龄侧柏不同插穗浸提液占比对白菜籽发芽率的影响Table 2. Effects of cabbage seeds treated with different proportion extraction solutions from cuttings propagated from P. orientalis at different ages on germination rates in June% 树龄/a
Tree age/year0（CK） 25% 50% 100% 5 96.60 ± 1.81a 88.15 ± 8.62a 83.47 ± 6.94a 78.67 ± 5.97a 100 96.60 ± 1.81a 73.33 ± 7.16b 72.00 ± 7.25ab 65.00 ± 6.26b 300 96.60 ± 1.81a 73.67 ± 7.10b 68.33 ± 5.92b 65.67 ± 6.29b 700 96.60 ± 1.81a 72.67 ± 7.13b 65.67 ± 6.97b 63.67 ± 6.16b 2.3 不同季节侧柏插穗类黄酮物质含量的变化
季节对林木酚酸含量有显著影响，本研究用HPLC对各类插穗内源抑制物进行分离，最终只检测出2种类黄酮物质：芦丁和槲皮素。3月份5、100、300、700年生侧柏插穗的芦丁含量显著高于6月份（P < 0.05），而6月份的槲皮素含量则显著低于3月份（P < 0.05），这表明季节对各树龄侧柏插穗的类黄酮含量影响显著（图2）。侧柏100、300、700年生侧柏插穗的芦丁含量在3月份和6月份都显著高于5年生侧柏（P < 0.05），5年生侧柏插穗槲皮素含量则显著高于100、300、700年生侧柏（P < 0.05），而100、300、700年生侧柏插穗之间的芦丁含量差异不显著（P > 0.05）。
![]()
图 2 不同树龄侧柏在不同季节类黄酮含量变化不同大写字母表示不同生根阶段在同一树龄的差异显著（P < 0.05）；不同小写字母表示不同树龄在同一生根阶段间差异显著（P < 0.05）。下同。Different capital letters indicate significant differences among varied rooting stages in same tree age (P < 0.05); different lowercase letters indicate significant differences among varied tree ages in same stage (P < 0.05). Same as below.Figure 2. Changes of flavonoids contents of P. orientalis at different ages in varied seasons2.4 不同季节侧柏插穗酚酸物质含量的变化
本研究检测出的酚酸物质包括香豆酸、阿魏酸、苯酚、水杨酸、没食子酸和邻苯二酚。结果发现3月份5、100、300、700年生侧柏插穗的香豆酸、水杨酸含量均高于6月份，特别是3月份700年生侧柏插穗的水杨酸、香豆酸含量较高，分别为40.47 和111.05 mg/g（图3）。3月份5、100、700年生侧柏插穗的阿魏酸、没食子酸、邻苯二酚含量均显著高于6月份（P < 0.05）。以上数据表明，3月份侧柏插穗的酚酸含量大多高于6月份，生长旺盛期的各种酚酸和类黄酮物质含量减少，说明6月份的枝条更适合侧柏古树扦插。5、100、300、700年生侧柏插穗香豆酸、水杨酸和邻苯二酚在3月份和6月份总体上呈现升高的趋势，这说明古树插穗的木质化程度较高，在不同季节中均具有较强的酚酸合成能力，可能与古树抗氧化和防御机制更强相关。
![]()
图 3 不同树龄侧柏在不同季节酚酸含量变化Figure 3. Changes of phenolic acid contents of P. orientalis at different ages in varied seasons2.5 扦插过程中不同种类酚酸、类黄酮的含量的变化
林木插穗中含有过量的酚酸和类黄酮对不定根形成具有抑制作用[19]。结果表明100、300、700年生侧柏插穗的芦丁含量在S1、S2和S3都显著高于5年生（P < 0.05），5、100、300、700年生侧柏扦插过程中芦丁含量随着生根阶段总体上呈上升趋势（图4），且在S3阶段达到峰值，分别为0.20、0.60、0.88 和0.90 mg/g，这表明芦丁对侧柏古树的生根具有抑制作用。而5年生侧柏插穗的槲皮素含量在S3时显著高于100、300 、700年生侧柏（P < 0.05），为1.49 mg/g。
![]()
图 4 不同树龄侧柏扦插过程中类黄酮含量变化S1为扦插试验当天，S2为愈伤组织形成期（第45天），S3为不定根形成期（第90天）。下同。S1 refers to the day of cutting experiment, S2 refers to callus formation period (the 45th day), and S3 refers to the adventitious root formation period (the 90th day). The same below.Figure 4. Changes of flavonoid content during rooting process of cuttings propagated from P. orientalis at different ages2.6 扦插过程中不同种类酚酸、类黄酮的含量的变化
不同树龄的酚酸含量在侧柏扦插生根过程的影响研究表明，300、700年生侧柏扦插过程中S2和S3的香豆酸含量显著高于5 年生侧柏插穗（图5）。100、300、700年生侧柏插穗的水杨酸、邻苯二酚含量随生根进程显著上升，并且在S1、S2和S3显著高于5 年生侧柏插穗（P < 0.05）。没食子酸的含量随树龄升高总体上呈现升高的趋势。除此之外，5 年生侧柏插穗的苯酚、阿魏酸含量在S1时显著高于100、300、700年生侧柏古树插穗。结果说明香豆酸、水杨酸、没食子酸和邻苯二酚）的含量随年龄增加总体上呈现增加趋势。
![]()
图 5 不同树龄侧柏扦插过程中酚酸含量变化Figure 5. Changes of phenolic acid content during rooting process of cuttings propagated from P. orientalis at different ages不同生根阶段的酚酸含量对侧柏扦插的影响研究结果表明，5、100、700年生侧柏插穗的香豆酸含量随生根进程（S1 ~ S3）显著上升（P < 0.05）。100、300、700年生侧柏插穗的阿魏酸、苯酚含量在S3显著高于S1和S2，而5年生侧柏插穗的苯酚含量在3个生根时期没有显著变化。S2和S3时5、100、300、700年生侧柏插穗的没食子酸含量显著高于S1时的含量；S3时5、100、300、700年生侧柏插穗的邻苯二酚含量显著高于S1、S3时的含量。结果表明酚酸物质含量随生根阶段（S1 ~ S3）的进展显著增加，尤其是在700年生插穗中的S3阶段，表明年龄较大的插穗在生根过程中会积累更多的酚类化合物。
2.7 清除内源生根抑制物对扦插生根的影响
清除100年生侧柏插穗的内源生根抑制物的试验结果显示，不同清除抑制物方式对扦插生根结果存在显著差异（P < 0.05）（表3）。使用0.1%硝酸银、清水、0.05%高锰酸钾处理100年生侧柏插穗能显著提高扦插生根率，生根率分别为26.55%、22.97%、23.63%，高于用0.1% 醋酸、2%乙醇、洗洁精处理后的生根率。此外，0.1%硝酸银和0.05%高锰酸钾处理100年生侧柏插穗能提高扦插生根数，生根数分别为5.13和5.44，高于其他方法处理后的生根率。0.1%硝酸银和0.05%高锰酸钾处理插穗后扦插生根效果较好，说明适合的方法能清除侧柏古树的内源抑制物，提高扦插生根率。
表 3 清除抑制物对100年生侧柏古树扦插生根的影响Table 3. Effects of removing inhibitors on rooting rates of cuttings of 100 years old ancient P. orientalis清除方式
Clear method处理时间
Treating time/h生根率
Rooting rate/%生根数
Rooting number最长根长
Max. root length/cm0.1% AgNO3 20 26.55 ± 2.66a 5.13 ± 0.48a 1.68 ± 0.22a 0.1% CH3COOH 20 19.25 ± 2.28b 2.52 ± 0.23c 1.24 ± 0.29b 2% EtOH 20 21.14 ± 3.24b 3.78 ± 0.39b 1.57 ± 0.19ab 水Water 3 22.97 ± 2.98ab 3.16 ± 0.46bc 1.52 ± 0.21ab 洗洁精Abluent 3 18.52 ± 2.72b 3.59 ± 0.77b 1.17 ± 0.26b 0.05% KMnO4 0.25 23.63 ± 2.91ab 5.44 ± 0.69a 1.95 ± 0.23a 注：不同小写字母表示不同清除方法之间存在显著差异（P < 0.05）。Note: different lowercase letters indicate significant differences at 0.05 level between removal methods. 3. 讨 论
林木的树龄和季节是影响扦插生根的重要因素[4]。本研究发现随树龄的增加，侧柏扦插生根率降低，这与马尾松、桉树等林木扦插生根的结果一致[10,20]。同时，插穗的浸提液抑制白菜种子发芽的作用增强，与沉水樟和核桃楸等林木的研究结果相似[5,12]。而侧柏插穗的水杨酸、香豆酸等酚酸物质在3月份的含量普遍高于6月份。因此，6月份较适合侧柏古树扦插，这与荷花玉兰（Magnolia grandiflora）扦插的最佳时间同期[6]。这主要是因为6月份插穗生长旺盛，营养物质（可溶性和淀粉）含量高，次生物质（酚酸和类黄酮）含量少，木质化程度低[9]。本研究根据树龄和季节对林木扦插生根的重要性，选择适宜的季节进行插穗扦插有助于提高生根率。
当插穗中的酚酸化合物含量较高时，扦插不定根的形成受阻[21]。随着树龄增长，侧柏古树（100、300、700年生）插穗的芦丁和水杨酸等酚酸和类黄酮物质含量升高，这表明古树扦插生根率低可能与侧柏古树插穗木质化程度高导致的酚酸和类黄酮物质积累有关[9]。牛樟（Cinnamomum kanehirae）插穗在木质素含量高时，不定根形成困难[22]。樱桃（Prunus spp.）插穗木质化程度高时，酚酸物质（芦丁、香草酸、表儿茶素、咖啡酸和芥子酸）含量也高，而扦插生根率却比较低，与本文的研究结果相似[7]。这些抑制物在树龄较大的侧柏插穗中的含量普遍较高，进一步说明了抑制物（酚酸和类黄酮）是导致古树难生根的关键因素之一。
酚酸类物质的积累与植物抵御胁迫有关，但也对扦插生根具有抑制作用。在生根的不同阶段（S1 ~ S3），各种酚类化合物（如水杨酸、没食子酸和邻苯二酚）的含量随生根的进程显著增加，700年生侧柏的插穗在S3阶段的酚酸含量显著高于其他年龄组。水杨酸作为逆境内源信号参与植物的抗性，是侧柏插穗在扦插过程中受到病菌侵染产生的应激反应物质。侧柏古树（100、300、700年生）的插穗在S3阶段水杨酸含量较高，使愈伤组织趋于成熟生长，再分化能力降低[23]。如巴拉圭冬青（Ilex paraguariensis）插穗在高光照胁迫下总酚含量升高，但是生根率却降低[24]。这主要是因为在胁迫条件下，插穗中的酚酸和类黄酮物质积累增加，这些物质不仅具有增加细胞壁厚度、提高细胞刚性的作用，还会抑制扦插不定根形成。因此，未来的研究可以重点关注通过调控类黄酮和酚酸物质的含量来提高林木插穗的生根能力。
有些酚酸和类黄酮物质可能对林木扦插生根起到促进作用。本研究显示，6月份5年生侧柏插穗中槲皮素和阿魏酸含量高，说明在幼嫩的植物组织中有些种类的酚酸含量高，这与苦丁茶（IIex kudingcha）嫩叶比老叶中的槲皮素含量高的结果相似[25]。5年生侧柏插穗在生根阶段槲皮素和邻苯二酚的含量增加，这可能是由于槲皮素可保护吲哚乙酸（IAA）免受羧化作用的伤害，从而促进生根。在Rosa bourboniana嫩枝扦插过程中，高浓度的酚类促进不定根发生[26]，这主要是因为酚酸在不定根形成过程中能提高插穗抗逆能力。蛇足石杉（Huperzia serrata）插穗中IAA、儿茶酚、绿原酸、阿魏酸的含量与PPO、POD的活性正比时促进扦插生根[27]。这些不同种类的酚酸和类黄酮物质对扦插生根的影响还需要进一步研究。
通过清除插穗中抑制物（如酚酸和类黄酮），能够提高侧柏扦插的生根率[28]。本研究发现，使用0.1%的硝酸银或0.05%的高锰酸钾溶液处理侧柏插穗，能有效促进不定根的形成。这与使用0.2%硝酸银溶液处理弗吉尼亚栎（Quercus vinginiana）插穗、3%乙醇与0.5%高锰酸钾的组合处理马尾松插穗能获得良好的生根效果相似[29]。因此，需要进一步筛选和优化清除插穗抑制物的对比试验，以获得较好的生根效果。
4. 结 论
综上所述，随着树龄的增长，5、100、300、700年生侧柏扦插生根的能力下降，表明树龄与扦插生根能力呈负相关关系。同时，侧柏插穗的浸提液抑制白菜种子发芽的作用增强。除了槲皮素和苯酚外，100、300、700年生侧柏的酚酸和类黄酮物质含量大都高于5年生侧柏。除了槲皮素和阿魏酸外，3月份的酚酸和类黄酮物质含量普遍高于6月份。随着生根阶段（S1 ~ S3）的推进，酚酸含量显著增加，尤其在700年树龄插穗的S3阶段，年龄较大的母树插穗在生根过程中会积累更多的酚类化合物。使用0.1%的硝酸银处理侧柏插穗后生根率和生根数量较高，而0.05%的高锰酸钾处理后插穗不定根的长度增长。本文通过研究不同树龄侧柏插穗的酚酸和类黄酮物质的作用以及清除方法，为提高林木的扦插生根率提供了理论依据。
-
![]()
图 2 嵌锁法加工集成竹格栅夹芯板示意图
Figure 2. Schematic diagram of laminated bamboo sandwich panel processed by interlocking method
![]()
图 3 嵌锁法加工集成竹格栅夹芯板加工流程图
Figure 3. Process flowchart of laminated bamboo sandwich panel processed by interlocking method
![]()
图 4 集成竹格栅夹芯板弯曲试验加载示意（a）与测点布置（b）
Figure 4. Loading diagram (a) and measuring point arrangement (b) of bending test for laminated bamboo sandwich panel
![]()
图 7 集成竹格栅夹芯板的四点抗弯试验破坏形态
Figure 7. Failure mode of four-point bending test for laminated bamboo sandwich panel
表 1 集成竹的密度和主要力学性能
Table 1 Density and main mechanical properties of laminated bamboo
材料 密度/（g·cm−3） 顺纹抗拉强度/MPa 顺纹抗压强度/MPa 顺纹剪切强度/MPa 集成竹 0.64 ± 0.02 114.5 ± 6.7 59.7 ± 4.0 18.9 ± 1.1 变异系数/% 2.5 5.8 6.7 6.0 
下载: 导出CSV
表 2 集成竹格栅夹芯板结构的几何参数
Table 2 Geometric parameters of laminated bamboo sandwich panel
试件构型 试件数量 夹芯板 竹板厚度/mm 芯体厚度/mm 面层厚度/mm 长格栅数量 短格栅数量 长度/mm 宽度/mm 厚度/mm 三角形格栅 3 1 200 384 64 8 48 8 5 方形格栅 3 1 200 384 64 8 48 8 5 15 Kagome形格栅 3 1 200 384 64 8 48 8 4
下载: 导出CSV
表 3 集成竹材料参数
Table 3 Material parameters of laminated bamboo
弹性模量/MPa 泊松比 剪切模量/MPa E11 E22 E33 μ12 μ13 μ23 G12 G13 G23 9 200 920 460 0.36 0.30 0.29 690 552 158 注：1、2、3分别表示试件跨度方向、试件截面宽度方向、试件截面高度方向。数据引自参考文献[19]。
下载: 导出CSV
表 4 极限荷载、跨中最大位移以及应变的试验和有限元模拟结果对比
Table 4 Comparison of experimental and finite element simulation results for ultimate load, maximum displacement at midspan and strain
试件构型 试验值 模拟值 相对误差/% Pu/kN Δu/mm εc × 106 εt × 106 Pu/kN Δu/mm εc × 106 εt × 106 Pu Δu εc εt 三角形格栅 38.7 25.1 −5 693.8 4 424.9 38.5 25.2 −5 865.7 4 235.3 0.5 0.4 −3.0 4.3 方形格栅 22.5 14.7 −2 575.8 2 580.3 23.5 15.3 −2 558.2 2 549.8 4.4 4.0 −0.7 1.2 Kagome形格栅 27.5 16.8 −3 142.4 3 124.5 27.0 17.0 −3 049.4 3 004.5 1.9 1.0 −3.0 3.8 注：试验值为同组试件弯曲试验下的平均值；Pu为极限荷载，Δu为跨中最大位移，εc为压应变，εt为拉应变。
下载: 导出CSV
表 5 集成竹格栅夹芯板刚度、强度性能
Table 5 Stiffness and strength properties of laminated bamboo sandwich panel
试件构型 相对密度/（g·cm−3） 抗弯刚度/（kN·m2） 抗弯强度/MPa 比刚度 × 103/（kN·m5·kg−1） 比强度/（kN·m·kg−1） 三角形格栅 0.30 45.7 ± 0.2 40.7 149.9 ± 5.0 133.5 方形格栅 0.26 40.6 ± 0.1 24.4 157.5 ± 2.1 94.6 Kagome形格栅 0.29 43.1 ± 0.2 28.5 146.1 ± 5.1 96.7 注：比刚度为结构的抗弯刚度与其相对密度的比值，比强度为结构的抗弯强度与其相对密度的比值。
下载: 导出CSV
-
[1] Flander K D, Rovers R. One laminated bamboo-frame house per hectare per year[J]. Construction and Building Materials, 2009, 23(1): 210−218. doi: 10.1016/j.conbuildmat.2008.01.004
[2] 卿彦. “双碳”战略目标下木竹基先进功能材料研究进展[J]. 中南林业科技大学学报, 2022, 42(12): 13−25. Qing Y. Advanced functional materials derived from natural wood and bamboo resources under the double carbon strategy in China[J]. Journal of Central South University of Forestry & Technology, 2022, 42(12): 13−25.
[3] 黄东梅, 周培国, 张齐生. 竹结构民宅的生命周期评价[J]. 北京林业大学学报, 2012, 34(5): 148−152. Huang D M, Zhou P G, Zhang Q S. Life cycle assessment of bamboo-constructed house[J]. Journal of Beijing Forestry University, 2012, 34(5): 148−152.
[4] Li Z H, Chen C J, Mi R Y, et al. A strong, tough, and scalable structural material from fast-growing bamboo[J]. Advanced Materials, 2020, 32(10): 1906308. doi: 10.1002/adma.201906308
[5] Lurie S A, Solyaev Y O, Volkov-Bogorodskiy D B, et al. Design of the corrugated-core sandwich panel for the arctic rescue vehicle[J]. Composite Structures, 2017, 160: 1007−1019. doi: 10.1016/j.compstruct.2016.10.123
[6] Hopkins P M, Norris T, Chen A. Creep behavior of insulated concrete sandwich panels with fiber-reinforced polymer shear connectors[J]. Composite Structures, 2017, 172: 137−146. doi: 10.1016/j.compstruct.2017.03.038
[7] 罗熠民, 郑毅, 李荣. 纤维增强复材混合芯层夹芯板受弯性能试验研究[J]. 工业建筑, 2020, 50(3): 167−171. Luo Y M, Zheng Y, Li R. Experimental research on flexural behavior of FRP sandwich panels with hybrid core[J]. Industrial Construction, 2020, 50(3): 167−171.
[8] Wang Z, Yang X L, Lai W G, et al. A VAM-based equivalent model for triangular honeycomb sandwich panels: comparison with numerical and experimental data[J]. Materials, 2022, 15(14): 4766. doi: 10.3390/ma15144766
[9] Manalo A C, Aravinthan T, Karunasena W. Flexural behaviour of glue-laminated fibre composite sandwich beams[J]. Composite Structures, 2010, 92(11): 2703−2711. doi: 10.1016/j.compstruct.2010.03.006
[10] Ashby M F. The properties of foams and lattices[J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2006, 364(1838): 15−30. doi: 10.1098/rsta.2005.1678
[11] 范华林, 金丰年, 方岱宁. 格栅结构力学性能研究进展[J]. 力学进展, 2008(1): 35−52. doi: 10.3321/j.issn:1000-0992.2008.01.002 Fan H L, Jin F N, Fang D N. Structural mechanics of lattice grids[J]. Advances in Mechanics, 2008(1): 35−52. doi: 10.3321/j.issn:1000-0992.2008.01.002
[12] 吴启凡, 方海, 高峰, 等. 复合材料格栅板受弯性能试验研究与数值分析[J]. 复合材料科学与工程, 2020(1): 12−20. doi: 10.3969/j.issn.1003-0999.2020.01.002 Wu Q F, Fang H, Gao F, et al. Experimental testing and numerical simulation on flexural behavior of composite grid panels[J]. Composites Science and Engineering, 2020(1): 12−20. doi: 10.3969/j.issn.1003-0999.2020.01.002
[13] 王亚楠, 梅志远, 李华东, 等. 格栅增强夹芯板弯曲刚度影响因素及规律研究[J]. 复合材料科学与工程, 2018(2): 47−51. doi: 10.3969/j.issn.1003-0999.2018.02.008 Wang Y N, Mei Z Y, Li H D, et al. Research on the influencing factors and laws about bending stiffness of grille reinforced sandwich panel[J]. Composites Science and Engineering, 2018(2): 47−51. doi: 10.3969/j.issn.1003-0999.2018.02.008
[14] Li J H, Hunt J F, Gong S Q, et al. Fatigue behavior of wood-fiber-based tri-axial engineered sandwich composite panels (ESCP)[J]. Holzforschung, 2016, 70(6): 567−575. doi: 10.1515/hf-2015-0091
[15] Wang Z G, Li Z D, Xiong W. Experimental investigation on bending behavior of honeycomb sandwich panel with ceramic tile face-sheet[J]. Composites Part B: Engineering, 2019, 164: 280−286. doi: 10.1016/j.compositesb.2018.10.077
[16] Fan H L, Meng F H, Yang W. Sandwich panels with Kagome lattice cores reinforced by carbon fibers[J]. Composite Structures, 2007, 81(4): 533−539. doi: 10.1016/j.compstruct.2006.09.011
[17] 国家标准化管理委员会. 无疵小试样木材物理力学性质试验方法 第2部分 取样方法和一般要求: GB/T 1927.2—2021[S]. 北京: 中国标准出版社, 2021. Standardization Administration. Test methods for physical and mechanical properties of small clear wood specimens (part 2): sampling methods and general requirements: GB/T 1927.2—2021[S]. Beijing: Standards Press of China, 2021.
[18] 中华人民共和国住房和城乡建设部. 木结构试验方法标准: GB/T 50329—2012[S]. 北京: 中国建筑工业出版社, 2012. Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for test methods of timber structures: GB/T 50329—2012[S]. Beijing: China Architecture & Building Press, 2012.
[19] 朱其伟. 竹木复合梁层间剪切与弯剪性能试验研究[D]. 南京: 南京林业大学, 2023. Zhu Q W. Experimental study on Interlaminar shear and bending shear properties of bamboo-wood composite beams[D]. Nanjing: Nanjing Forestry University, 2023.
[20] 徐秉业, 刘信声, 沈新普. 应用弹塑性力学 [M]. 北京: 清华大学出版社, 2017. Xu B Y, Liu X S, Shen X P. Applied elastoplastic mechanics [M]. Beijing: Tsinghua University Press, 2017.
[21] 王智丰, 李贤军, 易锦, 等. 大跨胶合木拱桥人致振动及其优化控制[J]. 土木工程学报, 2021, 54(4): 79−94. Wang Z F, Li X J, Yi J, et al. Human-induced vibration and optimal control of long-span glulam arch bridges[J]. China Civil Engineering Journal, 2021, 54(4): 79−94.
-
期刊类型引用(14)
1. 姬新颖，唐佳莉，李敖，郑旭，王红霞，张俊佩. 盐胁迫下不同基因型核桃实生幼苗生长及生理响应. 林业科学. 2024(02): 65-77 . 
百度学术
2. 孙叶烁，田海英，姚玉涛，丁守鹏，张国新，韩民利. 咸水胁迫对草莓生长·品质的影响及耐盐综合评价. 安徽农业科学. 2023(02): 47-50 . 百度学术
3. 纪发达. 一种抗逆型复合调节剂的制备及其应用. 化肥设计. 2023(02): 8-10 . 百度学术
4. 高铖铖，燕丽萍，吴德军，王因花. 基于SSR标记的白蜡群体遗传多样性和群体结构分析. 中南林业科技大学学报. 2023(06): 69-78 . 百度学术
5. 王介华，睢金凯，崔令军，石开明. AMF对盐胁迫下桢楠生长和生理特性的影响. 中南林业科技大学学报. 2023(06): 51-58 . 百度学术
6. 程俊森，王溢，李永泉，魏尚霖，李超楠，姜维，黄润生. 基于Label-free技术的高州油茶铝胁迫蛋白质组学研究. 中南林业科技大学学报. 2023(08): 169-181 . 百度学术
7. 刘京，马洁怡，张金池，马仕林，王宇浩，聂晖，曹鹏翔. 不同土壤改良措施对苏北盐碱地薄壳山核桃生长的影响. 东北林业大学学报. 2022(07): 11-16 . 百度学术
8. 刘翠兰，王开芳，吴德军，燕丽萍，李善文，王芳，任飞，王因花. 滨海盐碱胁迫下白蜡无性系生长及生理特性的响应. 中国农学通报. 2022(35): 7-16 . 百度学术
9. 李寿冰，赵钰琪，曹帮华，袁洪振，耿颖，陈炳卓，张家昌. 盐碱胁迫对2种地被竹叶绿素含量的影响. 世界竹藤通讯. 2022(S1): 35-43 . 百度学术
10. 崔令军，刘瑜霞，林健，石开明. 丛枝菌根真菌对盐胁迫下桢楠光合生理的影响. 南京林业大学学报(自然科学版). 2021(01): 101-106 . 百度学术
11. 巩志勇，辛建华，商小雨，郭晓明，王延平. 盐碱胁迫下香椿幼苗光合及抗逆生理特性. 西北植物学报. 2021(07): 1199-1209 . 百度学术
12. 范馨月，张华，赵继业，杜子沫，丛日晨. 绒毛白蜡耐盐碱响应机制的研究进展. 中国农学通报. 2021(28): 28-34 . 百度学术
13. 缪李飞，于晓晶，张秋悦，封超年. 4个杜梨半同胞家系苗期耐盐性分析. 南京林业大学学报(自然科学版). 2020(05): 157-166 . 百度学术
14. 赵宝泉，邢锦城，温祝桂，徐海东，王进左，李玉明，董静，洪立洲. 林木盐胁迫响应机制研究进展. 现代农业科技. 2020(21): 159-165 . 百度学术
其他类型引用(11)
计量
- 文章访问数: 226
- HTML全文浏览量: 17
- PDF下载量: 42
- 被引次数: 25
