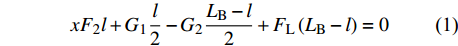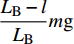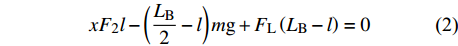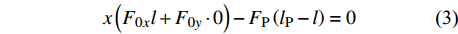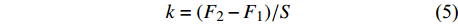Analysis and selection of gas spring of closet-type 0−90° foldable bed
-
摘要:目的壁柜式翻转床是现代小户型实现一房两用功能的重要形式,而其气弹簧的选择以“试”为主,缺乏理论支撑和实践指导。为了减少试错试验时间和提高产品设计的可靠性,拟从理论着手研究壁柜式翻转床的气弹簧设计和选型。方法以壁柜式翻转床为研究对象,根据其实际工况设定床体的极限工作条件;在床体极限工作条件下,运用理论力学中的静力学和力矩平衡原理,结合气弹簧运动特性曲线和技术标准对床体和气弹簧机构进行受力分析,完成气弹簧的选型;再运用两圆相交的几何方法得到气弹簧机构在床体的安装位置;最后对任意翻转角度下的床体进行受力分析,以CAD作为辅助测量工具,模拟床体运动曲线并分析其运动规律。结果构建了翻转床气弹簧机构分析计算理论,从使用和收纳两个极限状态着手,建立了气弹簧最小伸展力、行程和安装位置的确立方法;并进一步对任意翻转角度下的床体进行受力分析计算,得出翻转床的运动规律以及翻转床悬停角度的分析方法。并以市场常见床体尺寸(宽900 mm、长1 900 mm)、固定柜体目标深度300 mm为例,代入本方法求得气弹簧最小伸展力为428 N,行程为190 mm,弹性系数为1.06,得出悬停范围为18° ~ 24°。结论本研究构建的分析方法和结果可为家具行业的壁柜式翻转床设计、选型和性能分析提供理论支撑和实践指导。对于气弹簧机构尺寸和安装位置的确定,可以进一步通过有限元分析方法对壁柜侧板进行受力分析,进而优化力臂选择。Abstract:ObjectiveThe foldable bed is an important technology of the small apartment to realize the dual-use function. The selection of gas spring is mainly based on " wrong-trial”, which is lacking theoretical support and practical guidance. In order to shorten the production cycle and improve product reliability, it is especially important to conduct a theory research.MethodIn this study, the foldable bed was taken as the research object, and its limit working conditions were set according to the actual working conditions. Based on statics and torque balance principle in theoretical mechanics, combined with the gas spring motion characteristic curve and its technical standards, the force analysis of the foldable bed and its gas spring was conducted under the limit working conditions of the foldable bed to complete the selection of the gas spring. The optimal install location of the gas spring on the bed was obtained using two intersecting circles. The force analysis of the bed at random foldable angles was analyzed and its motion curve was simulated via CAD-aided measuring tools.Result This study set up the analysis and calculation theory of the gas spring of the foldable bed. Starting with two limit states of the use and storage, the theory to obtain minimum extension force, stroke and installation position of the gas spring was established; furthermore, the foldable bed at random foldable angle was analyzed and calculated. The motion law and the analysis method of the hover angle were obtained. This study took the common bed size (900 mm wide, 1 900 mm long) , 300 mm depth as an example, the minimum extension force, the stroke, the elastic coefficient, and the range of hover scope of the gas spring were 428 N, 190 mm, 1.06, and 18°−24°.Conclusion The analytical methods and results constructed in this study can provide theoretical support and practical guidance for the design, selection and performance analysis of foldable bed in the furniture industry. For the determination of the size and installation position of the gas spring, the force analysis of the sideboard of the foldable bed can be further carried out by means of finite element analysis, so as to optimize the selection of the force arm of the gas spring.
-
Keywords:
- foldable bed /
- gas spring /
- force analysis /
- motion analysis
-
现如今城市居住空间紧张,小户型的比例在逐渐加大。中国政府出台规定建筑面积90平方米以下户型占比必须达到70%以上[1],这预示着更多的家庭面临功能空间不足的情况,就有大量家庭有一房两用的需求,即一个房间除了卧室的主要功能外,还可通过功能家具实现客厅或书房等功能,而其中翻转床则是实现睡眠–休闲、学习空间转换的功能家具的典型代表产品。
虽然翻转床有着可观的市场需求,但对于其力学性能的检测还是依赖整体破坏性试验为主要手段,目前其设计和分析尚缺乏科学的理论指导[2]。20世纪90年代气弹簧作为新型支撑出现,张琦等[3]对气弹簧的力学性能进行了计算分析;王殿武[4]研究了气弹簧力学特性并将其运用到汽车尾盖上;刘迎林等[5]对全塑车身后备箱气撑杆进行运动仿真并验证其安装位置;王定虎[6]运用力矩平衡原理和理想气体方程对汽车背门撑杆的选择及布置进行校核。截止目前,气弹簧的研究主要集中在汽车领域,而鲜有在家具领域内的研究,为了弥补翻转床气弹簧机构设计和性能分析的理论欠缺,本文从实际应用需求出发,运用静力学和力矩平衡原理对气弹簧机构进行结构分析计算和选型。
1. 研究方法
1.1 翻转床气弹簧机构概况
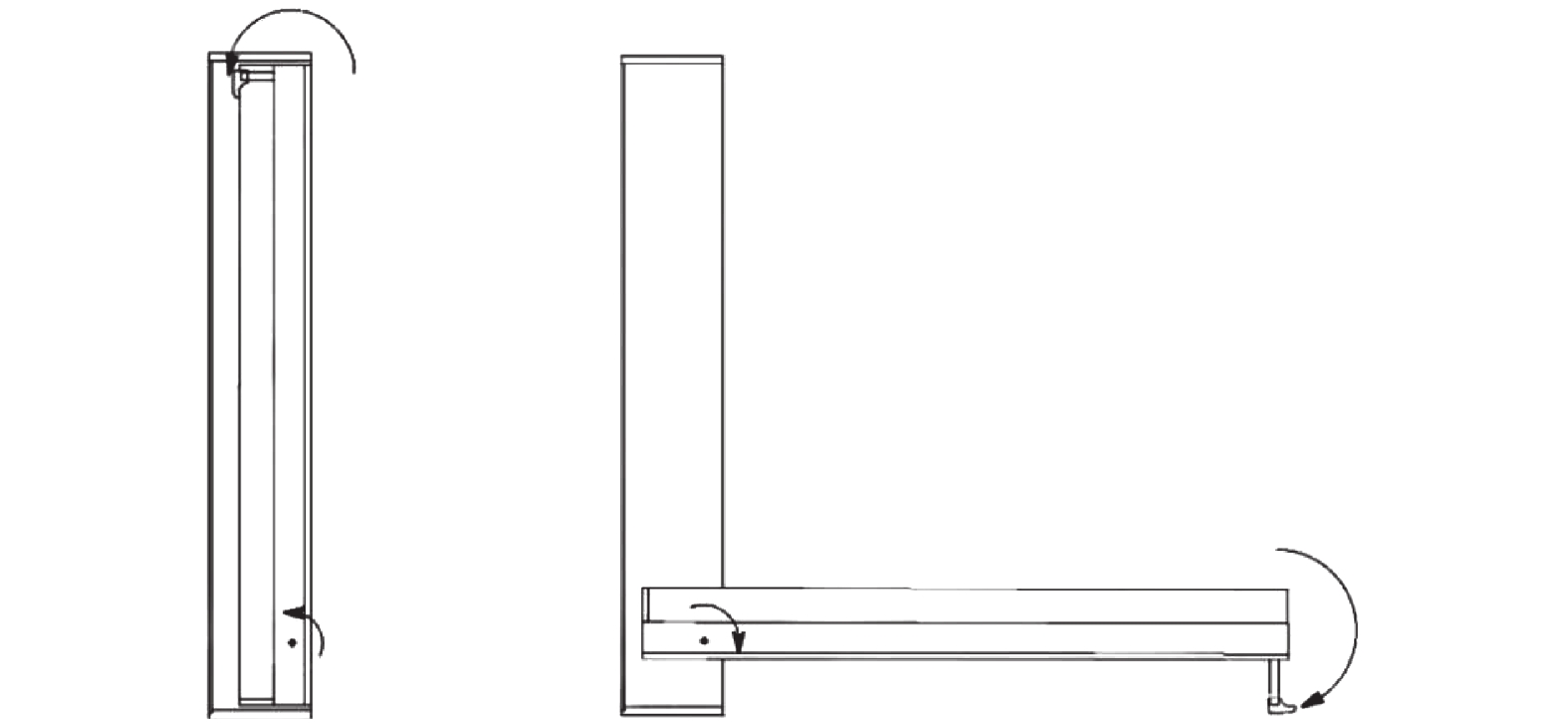
翻转床床体翻转的目的是实现床体的收纳,以便满足房间睡眠–休闲、学习空间转换的用户功能需求。根据使用场景分析,翻转床运动功能示意图如图1所示。因为翻转床的床体框架、床板、床垫和床上用品等零部件加起来质量较大,如仅凭借人手部力量支撑则翻转困难,且在操作过程中存在砸到人的风险,所以实际翻转床产品均需要借助辅助结构实现翻转和随停的功能。由于气弹簧具有支撑、缓冲的作用,因此恰好适用于翻转床的运动功能需求。
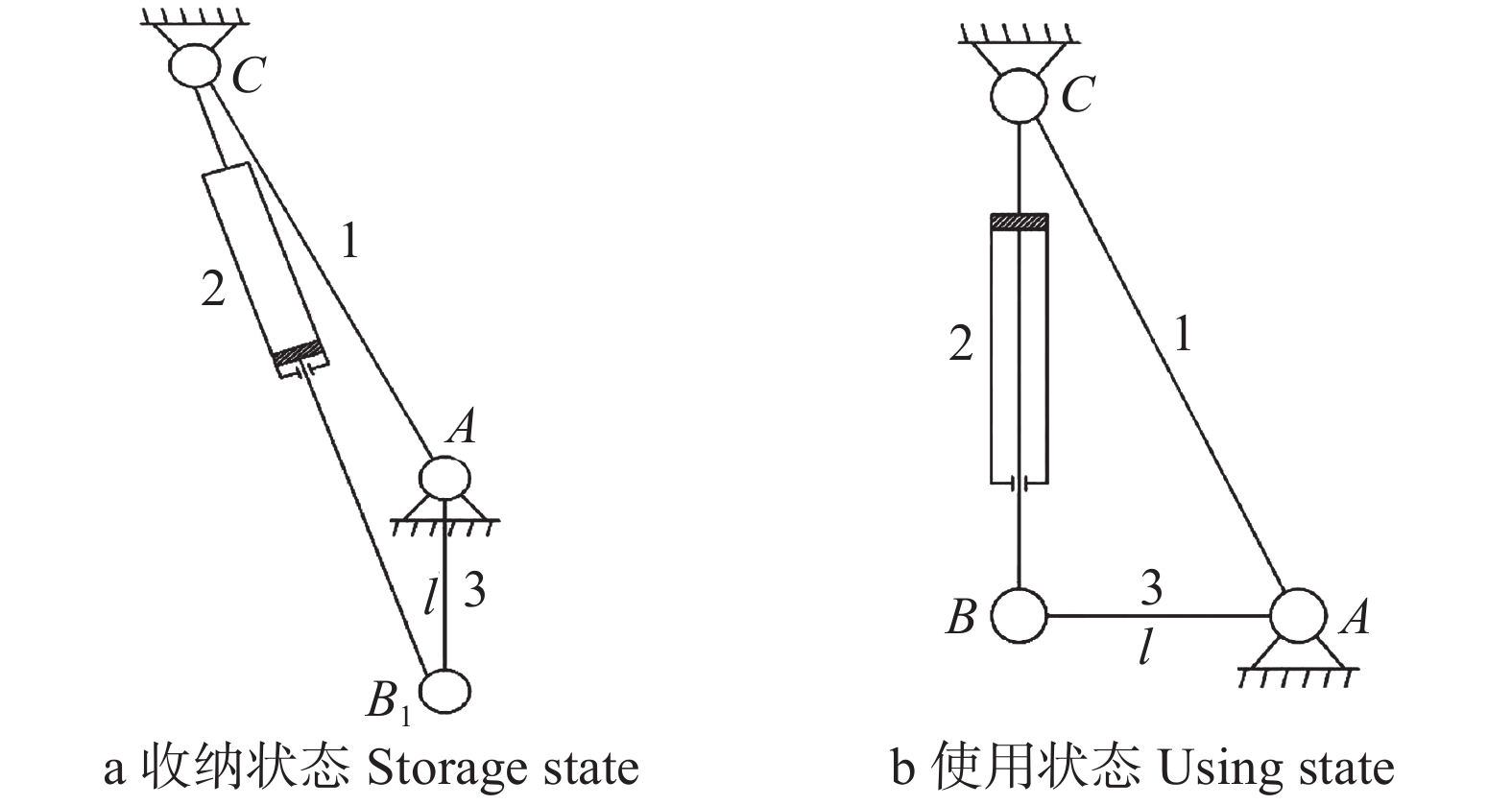
壁柜式翻转床的翻转功能主要由气弹簧机构实现,分析翻转床的运动本质就是分析气弹簧机构。壁柜式翻转床结构和气弹簧机构简图如图2所示,翻转床左右两侧具有相同连杆结构,其中A点为翻转床的翻转中心,由螺栓将床体翻转框架和固定柜体铰接;BC为气弹簧,气弹簧两端分别和固定柜体及床体翻转框架铰接;床体翻转存在两个极限状态,即收纳状态(图2a)和使用状态(图2b),处于收纳状态时气弹簧处在伸展状态,即B1C,处于使用状态时气弹簧处在压缩状态,即BC。
![]() 图 2 翻转床结构和气弹簧机构简图1. 固定柜体;2. 气弹簧;3. 床体翻转框架;A. 翻转中心;B. 气弹簧压缩末端;B1. 气弹簧伸展末端;C. 气弹簧固定端; l. A点到床头距离。 1, fixed cabinet; 2, gas spring; 3, rotate frame; A, rotation center; B, compression end of gas spring; B1, stretching end of gas spring; C, fixed end of gas spring; l, the distance from A point to the head of the bed.Figure 2. Diagram of foldable bed structure and gas spring mechanism
图 2 翻转床结构和气弹簧机构简图1. 固定柜体;2. 气弹簧;3. 床体翻转框架;A. 翻转中心;B. 气弹簧压缩末端;B1. 气弹簧伸展末端;C. 气弹簧固定端; l. A点到床头距离。 1, fixed cabinet; 2, gas spring; 3, rotate frame; A, rotation center; B, compression end of gas spring; B1, stretching end of gas spring; C, fixed end of gas spring; l, the distance from A point to the head of the bed.Figure 2. Diagram of foldable bed structure and gas spring mechanism1.2 气弹簧计算方法
根据国家标准GB 25751—2010压缩气弹簧技术条件、GB/T 1805—2001弹簧术语和JB/T 10418—2004气弹簧设计计算为依据,对气弹簧特性进行研究。对极限位置的床体进行平面力系的简化,并结合力矩平衡原理对床体和气弹簧机构进行受力分析。运用有限元的优化思路对气弹簧安装位置进行列表格寻最优解。运用静力学知识分析床体运动规律。
2. 结果与分析
2.1 气弹簧机构分析计算和选型
如图2所示,翻转床在收纳时气弹簧处于伸展过程,气弹簧的伸展力辅助床体的上翻过程。床体完全收纳进柜体时,此时床体重力矢量经过翻转中心A,在无外力情况下床体静止不动。翻转床展开过程中气弹簧处于压缩过程,气弹簧的压缩力为床体下翻过程提供缓冲力。
图3为气弹簧展开长度与压缩、伸展过程曲线示意图,其中F1为最小伸展力,F2为最大伸展力,F3为最小压缩力,F4为最大压缩力,S为气弹簧的行程,t为端头长度,结合图2气弹簧初始长度BC = S + t,展开长度L = B1C = S + t + S = 2S + t,t的取值一般为10 mm。
![]() 图 3 气弹簧展开长度与压缩、伸展过程曲线示意图d. 活塞杆直径;D1. 缸筒内径;D2. 缸筒外径;S. 行程;L. 伸展长度;t. 端头长度;F0. 启动力;F1. 最小伸展力;F2. 最大伸展力;F3. 最小压缩力;F4. 最大压缩力;Fa. 公称力a;Fb. 公称力b;C. 采力点。图引自文献[7]。d, piston rod diameter; D1, cylinder inner diameter; D2, cylinder outer diameter; S, stroke; L, extended length; t, end length; F0, star-up force; F1, minimum extension force; F2, maximum extension force; F3, minimum compress force; F4, maximum compress force; Fa, nominal force a; Fb, nominal force b; C, measuring point. Diagram is cited from reference [7].Figure 3. Diagram of expansion length of gas spring and curve of compression and stretching process
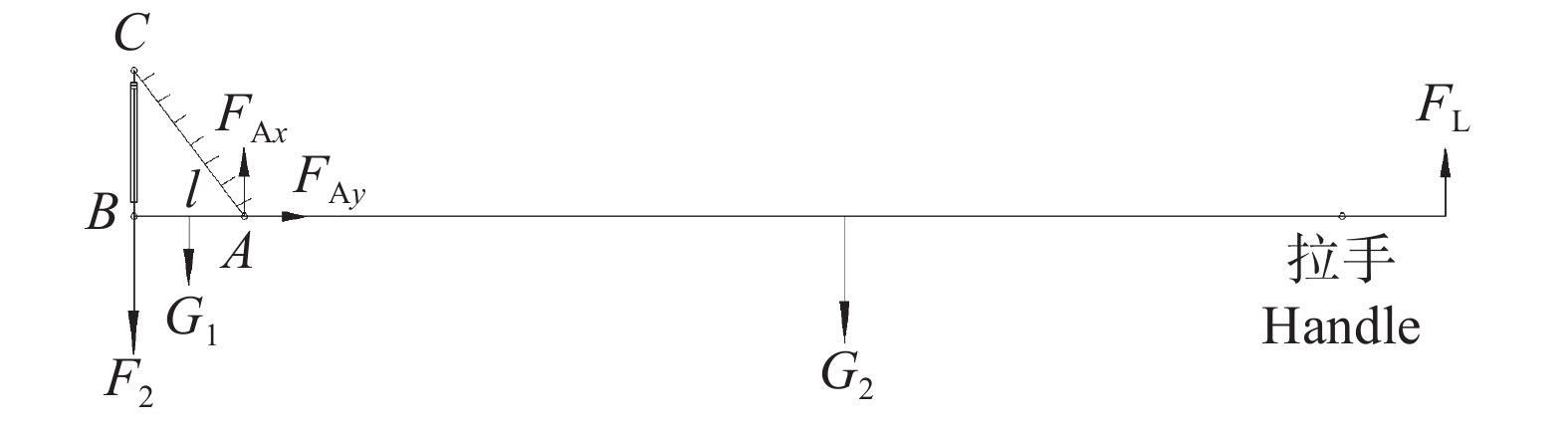
图 3 气弹簧展开长度与压缩、伸展过程曲线示意图d. 活塞杆直径;D1. 缸筒内径;D2. 缸筒外径;S. 行程;L. 伸展长度;t. 端头长度;F0. 启动力;F1. 最小伸展力;F2. 最大伸展力;F3. 最小压缩力;F4. 最大压缩力;Fa. 公称力a;Fb. 公称力b;C. 采力点。图引自文献[7]。d, piston rod diameter; D1, cylinder inner diameter; D2, cylinder outer diameter; S, stroke; L, extended length; t, end length; F0, star-up force; F1, minimum extension force; F2, maximum extension force; F3, minimum compress force; F4, maximum compress force; Fa, nominal force a; Fb, nominal force b; C, measuring point. Diagram is cited from reference [7].Figure 3. Diagram of expansion length of gas spring and curve of compression and stretching process气弹簧的选型需要的参数为气弹簧的伸展长度L和行程S以及气弹簧最小伸展力F1[8]。现以翻转床两个极限位置进行受力分析。使用状态下,当人手抬起床的边沿时以A点为旋转中心,能够将床体抬起。此时受力分析如图4所示。
![]() 图 4 使用状态手抬床体时床体受力分析简图A. 翻转中心;B. 气弹簧压缩末端;C. 气弹簧固定端;F2. 气弹簧最大伸展力;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;l. A点到床头距离;FL. 手对床体的抬力;A, rotation center;B, compression end of gas spring;C, fixed end of gas spring;F2, maximum extension force;G1, bed weight on the left side of point A;G2, bed weight on the right side of point A;FAx, x component of point A;FAy, y component of point A;l, A point to the head of the bed;FL, hand lift on the bed.Figure 4. Diagram of foldable bed force analysis when hand up the bed in using state
图 4 使用状态手抬床体时床体受力分析简图A. 翻转中心;B. 气弹簧压缩末端;C. 气弹簧固定端;F2. 气弹簧最大伸展力;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;l. A点到床头距离;FL. 手对床体的抬力;A, rotation center;B, compression end of gas spring;C, fixed end of gas spring;F2, maximum extension force;G1, bed weight on the left side of point A;G2, bed weight on the right side of point A;FAx, x component of point A;FAy, y component of point A;l, A point to the head of the bed;FL, hand lift on the bed.Figure 4. Diagram of foldable bed force analysis when hand up the bed in using state由力矩平衡可得:
xF2l+G1l2−G2LB−l2+FL(LB−l)=0 (1) 式中:F2为气弹簧最大伸展力,单位N;x为气弹簧个数;FL为手对床体的抬力,单位N;LB为床体总长,单位mm;G1为A点左侧床体质量与重力加速度之积,单位N;G2为A点右侧床体质量与重力加速度之积,单位N;l为A点到床头距离,单位mm。
设床体和床垫总质量为m,
G1=lLBmg ,G2= LB−lLBmg ,则可将式(1)简化得:xF2l−(LB2−l)mg+FL(LB−l)=0 (2) 式中:F2为气弹簧最大伸展力,单位N;x为气弹簧个数;FL为手对床体的抬力,单位N;LB为床体总长,单位mm;l为A点到床头距离,单位mm;m为床体和床垫总质量,单位kg;g为重力加速度,单位N/kg。
收纳状态下,拉手与A、B1点视作在同一竖直线上。拉动床体时,人手拉动拉手的力矩能够平衡气弹簧对A点的弹力矩,此时受力分析简图如图5所示。
![]() 图 5 收纳状态手拉床体时床体受力分析简图A. 翻转中心;B1. 气弹簧伸展末端;C. 气弹簧固定端;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;F0. 启动力;F0x. F0沿x轴方向分力;F0y. F0沿y轴方向分力;l. A点到床头距离;θ. F0与垂直方向夹角;FP. 手对拉手拉力。A, rotation center;B1, stretching end of gas spring;C, fixed end of gas spring;FAx, x component of point A;FAy, y component of point A; F0, star-up force; F0x, x component of F0; F0y. y component of F0; l, A point to the head of the bed; θ, angle of F0 with vertical direction; FP, hand pull on the handle.Figure 5. Diagram of foldable bed force analysis when hand drag the bed in storage state
图 5 收纳状态手拉床体时床体受力分析简图A. 翻转中心;B1. 气弹簧伸展末端;C. 气弹簧固定端;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;F0. 启动力;F0x. F0沿x轴方向分力;F0y. F0沿y轴方向分力;l. A点到床头距离;θ. F0与垂直方向夹角;FP. 手对拉手拉力。A, rotation center;B1, stretching end of gas spring;C, fixed end of gas spring;FAx, x component of point A;FAy, y component of point A; F0, star-up force; F0x, x component of F0; F0y. y component of F0; l, A point to the head of the bed; θ, angle of F0 with vertical direction; FP, hand pull on the handle.Figure 5. Diagram of foldable bed force analysis when hand drag the bed in storage state因为F0y与A点在水平方向上没有距离,所以F0在y轴方向上的力矩为0。由力矩平衡可得:
x(F0xl+F0y⋅0)−FP(lP−l)=0 (3) 式中:F0为气弹簧启动力,单位N;lP为拉手高度,单位mm;FP为手对拉手拉力,单位N;l为A点到床头距离,单位mm;x为气弹簧个数;F0x为F0沿x轴方向分力,单位N;F0y为F0沿y轴方向分力,单位N。
图5中,依据压缩气弹簧技术条件,气弹簧启动力F0略大于气弹簧最小压缩力F3,取F3值近似为F0值,由三角函数可知:
F0=F0xsinθ=F3 (4) 式中:θ为F0与垂直方向夹角,单位°;F0为气弹簧启动力,单位N;F0x为F0沿x轴方向分力,单位N;F3为气弹簧最小压缩力,单位N。
在符合人机工程的情况下,使lP尽量大可以加大手拉开床体的力矩,减小手部力量,取lP = 1 750 mm。依据人机工程学,为使得操作力比较恰当,收纳床体时推荐的操作力范围为50 ~ 80 N[9],此处取手对床体的抬力FL = 80 N,手拉拉手的力FP = 80 N。
F1与F3之间有一段由于摩擦力产生的差值,依据GB25751—2010其计算公式为Fr =(F3 − F1)/2,即动态摩擦力Fr是最小压缩力和最小伸展力之差的平均值[10]。气弹簧摩擦力所产生的阻力与杆的运动方向相反,其与标称力值(图样及产品上标注的力,包括F1、Fa、
F3⋯⋯ )极限偏差应符合下表1的规定。表 1 标称力值极限偏差与动态摩擦力Table 1. Nominal force limit deviation and dynamic friction标称力值 Nominal force 标称力值的极限偏差 Nominal force limit deviation 最大动态摩擦力 Maximum dynamic friction ≤ 100 + 15 − 5 25 101 ~ 200 + 20 − 10 30 201 ~ 400 + 30 − 15 40 401 ~ 600 + 40 − 20 60 601 ~ 800 + 50 − 25 80 801 ~ 1 000 + 60 − 30 100 1 001 ~ 1 200 + 70 − 35 130 > 1 200 + 80 − 40 150 注:表1引自文献[7]。Note: Tab.1 is cited from reference [7]. F1与F2的关系可由弹性系数求得。弹性系数k表示的是单位压缩力变化的弹簧常数[10],单位为N/mm,行程S的单位是mm。伸展阶段气弹簧弹性系数公式[11]为:
k=(F2−F1)/S (5) 其中,k的大小可由厂家进行调节,其具体值可通过实验得出。一般商家提供的气弹簧的弹性系数k介于1.05和1.8之间,弹性系数越小意味着制造难度越高。
以市场常见的床体规格为准,此处选取宽900 mm、长1 900 mm、质量为25 kg的床垫。选取匹配的床体框架结构的材质为钢,其质量约为25 kg。刨花板密度为650 kg/m3,则18 mm(厚) × 900 mm(宽) × 1 900 mm(长)的床板质量为20 kg。则床体总重力为:(25 + 25 + 20) × 9.8 = 686 N。如固定柜体目标深度为300 mm,为了保证A、C两点安装位置距离柜体板前后两边有足够的距离保证强度,则取l = 160 mm。
依据式(2),xF2 = 2 517.1 N,选取气弹簧个数x = 4,则F2 = 629.3 N。
由图4、图5可知:取l为160 mm时,以B、B1为圆心,以(S + 10)、(2S + 10)为半径作圆,作交点可得C点安装位置。并结合式(3)、式(4)、表1以及k的计算方程,可将相关参数整理成表2。
表 2 气弹簧相关参数及安装位置与行程S的关系Table 2. Relationship between gas spring stroke and relevant parameters and installation positionS/mm θ/° F3/N Fr/N F1/N k 170 18 643.2 180 20 581.1 60 461.1 0.934 190 23 508.0 40 428.0 1.059 200 26 453.4 40 373.4 1.280 注:S为行程;θ为F0与垂直方向夹角;F3为最小压缩力;Fr为最大动态摩擦力;F1为最小伸展力;k为气弹簧弹性系数。表3同此。Notes:S, stroke; θ, angle of F0 with vertical direction; F3, minimum compress force; Fr, maximum dynamic friction; F1, minimum extension force; k, gas spring modulus coefficient. Same as Tab.3. 由表2可知:θ角越大,k则越大,气弹簧的制作难度越小。为减小安装宽度,选择S为190 mm,F1 = 428 N作为最小伸展力的气弹簧,则要求厂家提供的气弹簧弹性系数k为1.06。参考表3可知F1和S的参数符合设计要求。
表 3 气弹簧活塞杆直径与最小伸展力大小选择范围推荐表Table 3. Recommended table of minimum extension force range and stroke range of gas spring序号
No.活塞杆
直径 Diameter of piston rod/mm最小伸展力
Minimum extension force (F1)/N行程范围
Stroke range/mm推荐范围
Recommended
range可选范围
Optional
range1 6 50 ~ 250 50 ~ 350 50 ~ 400 2 8 200 ~ 450 100 ~ 700 100 ~ 700 3 10 300 ~ 700 100 ~ 1 200 150 ~ 1 100 4 12 450 ~ 1 000 150 ~ 1 500 150 ~ 1 600 5 14 600 ~ 1 400 200 ~ 2 500 1 600 ~ 2 200 6 20 1 250 ~ 3 100 1 000 ~ 5 200 2 200 ~ 4 500 注:表3引自文献[12]。Note: Tab.3 is cited from reference [12]. 此时C点的安装位置如图6所示,BC = S + 10 = 200 mm,B1C = 2S + 10 = 390 mm。如果床体总质量加大,可以适当改变固定柜体深度以加大l的取值,使气弹簧机构获得更大的力臂。
2.2 翻转床运动规律分析
翻转床旋转到任意角度时的受力图如图7所示。取气弹簧对床体弹力FB与矩心A点的力臂为a,G1与矩心A点的力臂为b,G2与矩心A点的力臂为c。矩心A点右侧力矩减去左侧合力矩可列式:
![]() 图 7 任意位置下床架受力图A. 翻转中心;B′. 在β旋转角度下的床尾位置;C. 气弹簧固定端;β. 床体翻转框架翻转角度;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;a. FB对矩心A点的力臂;b. G1对矩心A点的力臂;c. G2对矩心A点的力臂。A, rotation center; B′, bed tail position at β rotation angle; C, fixed end of gas spring; β, flip angle of rotate frame; G1, bed mass on the left side of point A multiply gravity acceleration; G2, bed mass on the right side of point A multiply gravity acceleration; a, FB force arm to point A; b, G1 force arm to point A; c, G2 force arm to point A.Figure 7. Diagram of foldable bed force at arbitrary degree
图 7 任意位置下床架受力图A. 翻转中心;B′. 在β旋转角度下的床尾位置;C. 气弹簧固定端;β. 床体翻转框架翻转角度;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;a. FB对矩心A点的力臂;b. G1对矩心A点的力臂;c. G2对矩心A点的力臂。A, rotation center; B′, bed tail position at β rotation angle; C, fixed end of gas spring; β, flip angle of rotate frame; G1, bed mass on the left side of point A multiply gravity acceleration; G2, bed mass on the right side of point A multiply gravity acceleration; a, FB force arm to point A; b, G1 force arm to point A; c, G2 force arm to point A.Figure 7. Diagram of foldable bed force at arbitrary degreeMA=MA(xFB)+MA(G1)−MA(G2)=xFBa+G1b−G2c (6) 式中:MA为合力对A点的力矩,单位N;x为气弹簧个数;FB为气弹簧对床体弹力,单位N;G1为A点左侧床体重力,单位N;G2为A点右侧床体重力,单位N;a为FB对矩心A点的力臂,单位mm;b为G1对矩心A点的力臂,单位mm;c为G2对矩心A点的力臂,单位mm;MA(xFB)为单边气弹簧对A点力矩,单位N·mm;MA(G1)为G1对A点力矩,单位N·mm;MA(G2)为G2对A点力矩,单位N·mm。
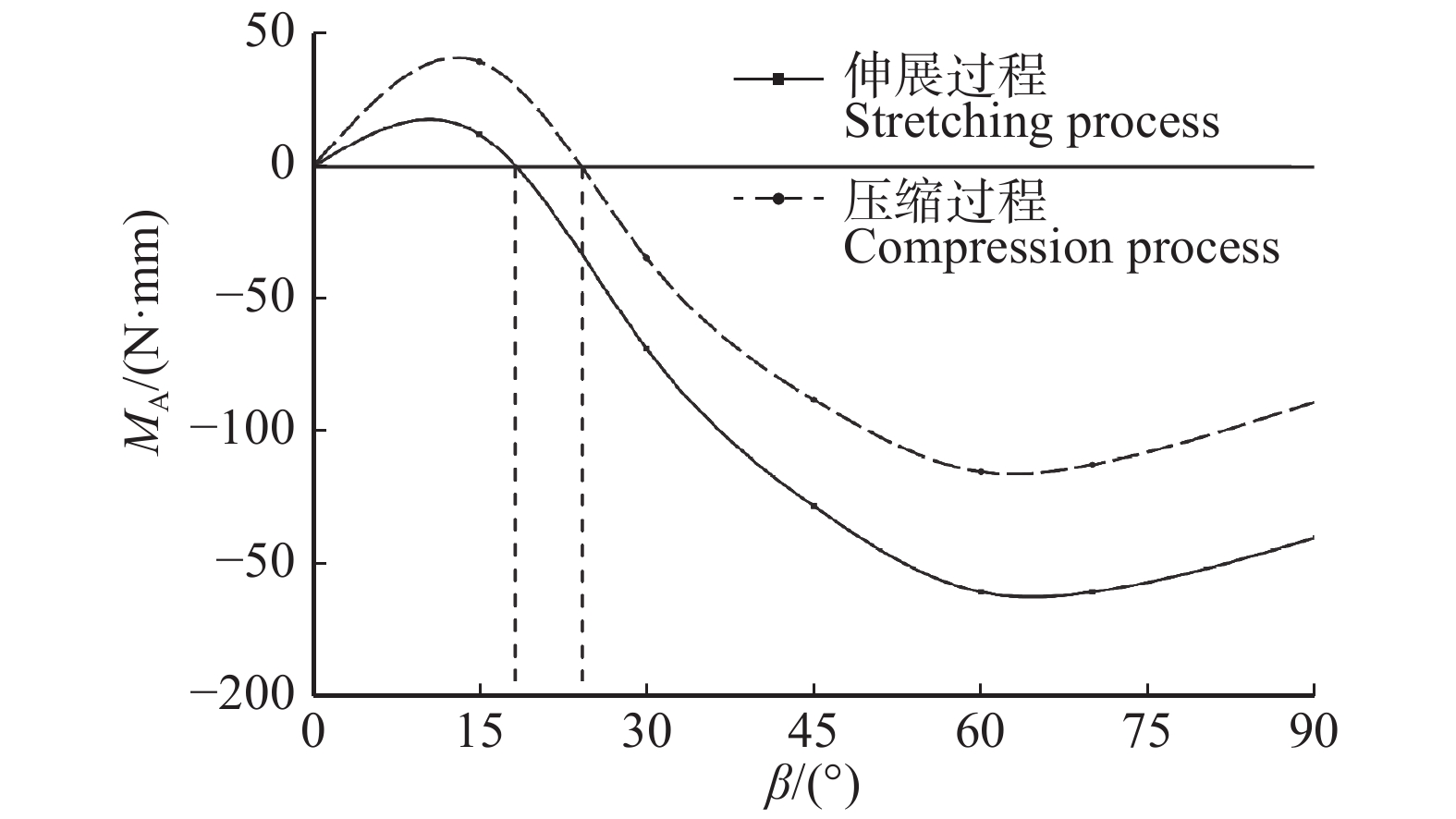
气弹簧压缩和伸展两个过程曲线中任意点的值可以用伸展长度和k值求出,在不同旋转角度β下分别量取a、b、c的值代入式(6),并作出β与MA的关系曲线如图8所示。图8两条曲线为分别代入了弹簧伸展过程力值和压缩过程力值后的曲线。由图 8可知:床体在打开18°以内会弹回收纳状态;18° ~ 24°之间床体可悬停;大于24°以后,A点右侧力矩大于A点左侧合力矩。
3. 结 论
基于静力学和力矩平衡原理完成了翻转床两个极限位置的受力分析,构建了翻转床气弹簧分析计算理论,运用该理论能够通过翻转床的床身质量和尺寸得到气弹簧的最小伸展力、行程和弹性系数,从而完成气弹簧选型;运用CAD工具做两圆相交的几何法得出气弹簧安装位置的确立方法;基于力矩平衡原理构建合力矩和翻转角度β的关系式,得出翻转床悬停范围。设定床体尺寸宽900 mm、长1 900 m、固定柜体目标深度300 mm,则可得气弹簧最小伸展力为428 N,行程为190 mm,弹性系数为1.06,悬停角度范围为18° ~ 24°,翻转角大于24°后则为自由下翻。本文构建的分析方法和结果可为家具行业的壁柜式翻转床设计、选型和性能分析提供理论支撑和实践指导。
-
图 2 翻转床结构和气弹簧机构简图
1. 固定柜体;2. 气弹簧;3. 床体翻转框架;A. 翻转中心;B. 气弹簧压缩末端;B1. 气弹簧伸展末端;C. 气弹簧固定端; l. A点到床头距离。 1, fixed cabinet; 2, gas spring; 3, rotate frame; A, rotation center; B, compression end of gas spring; B1, stretching end of gas spring; C, fixed end of gas spring; l, the distance from A point to the head of the bed.
Figure 2. Diagram of foldable bed structure and gas spring mechanism
图 3 气弹簧展开长度与压缩、伸展过程曲线示意图
d. 活塞杆直径;D1. 缸筒内径;D2. 缸筒外径;S. 行程;L. 伸展长度;t. 端头长度;F0. 启动力;F1. 最小伸展力;F2. 最大伸展力;F3. 最小压缩力;F4. 最大压缩力;Fa. 公称力a;Fb. 公称力b;C. 采力点。图引自文献[7]。d, piston rod diameter; D1, cylinder inner diameter; D2, cylinder outer diameter; S, stroke; L, extended length; t, end length; F0, star-up force; F1, minimum extension force; F2, maximum extension force; F3, minimum compress force; F4, maximum compress force; Fa, nominal force a; Fb, nominal force b; C, measuring point. Diagram is cited from reference [7].
Figure 3. Diagram of expansion length of gas spring and curve of compression and stretching process
图 4 使用状态手抬床体时床体受力分析简图
A. 翻转中心;B. 气弹簧压缩末端;C. 气弹簧固定端;F2. 气弹簧最大伸展力;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;l. A点到床头距离;FL. 手对床体的抬力;A, rotation center;B, compression end of gas spring;C, fixed end of gas spring;F2, maximum extension force;G1, bed weight on the left side of point A;G2, bed weight on the right side of point A;FAx, x component of point A;FAy, y component of point A;l, A point to the head of the bed;FL, hand lift on the bed.
Figure 4. Diagram of foldable bed force analysis when hand up the bed in using state
图 5 收纳状态手拉床体时床体受力分析简图
A. 翻转中心;B1. 气弹簧伸展末端;C. 气弹簧固定端;FAx. A点沿x轴方向分力;FAy. A点沿y轴方向分力;F0. 启动力;F0x. F0沿x轴方向分力;F0y. F0沿y轴方向分力;l. A点到床头距离;θ. F0与垂直方向夹角;FP. 手对拉手拉力。A, rotation center;B1, stretching end of gas spring;C, fixed end of gas spring;FAx, x component of point A;FAy, y component of point A; F0, star-up force; F0x, x component of F0; F0y. y component of F0; l, A point to the head of the bed; θ, angle of F0 with vertical direction; FP, hand pull on the handle.
Figure 5. Diagram of foldable bed force analysis when hand drag the bed in storage state
图 7 任意位置下床架受力图
A. 翻转中心;B′. 在β旋转角度下的床尾位置;C. 气弹簧固定端;β. 床体翻转框架翻转角度;G1. A点左侧床体质量与重力加速度之积;G2. A点右侧床体质量与重力加速度之积;a. FB对矩心A点的力臂;b. G1对矩心A点的力臂;c. G2对矩心A点的力臂。A, rotation center; B′, bed tail position at β rotation angle; C, fixed end of gas spring; β, flip angle of rotate frame; G1, bed mass on the left side of point A multiply gravity acceleration; G2, bed mass on the right side of point A multiply gravity acceleration; a, FB force arm to point A; b, G1 force arm to point A; c, G2 force arm to point A.
Figure 7. Diagram of foldable bed force at arbitrary degree
表 1 标称力值极限偏差与动态摩擦力
Table 1 Nominal force limit deviation and dynamic friction
标称力值 Nominal force 标称力值的极限偏差 Nominal force limit deviation 最大动态摩擦力 Maximum dynamic friction ≤ 100 + 15 − 5 25 101 ~ 200 + 20 − 10 30 201 ~ 400 + 30 − 15 40 401 ~ 600 + 40 − 20 60 601 ~ 800 + 50 − 25 80 801 ~ 1 000 + 60 − 30 100 1 001 ~ 1 200 + 70 − 35 130 > 1 200 + 80 − 40 150 注:表1引自文献[7]。Note: Tab.1 is cited from reference [7]. 表 2 气弹簧相关参数及安装位置与行程S的关系
Table 2 Relationship between gas spring stroke and relevant parameters and installation position
S/mm θ/° F3/N Fr/N F1/N k 170 18 643.2 180 20 581.1 60 461.1 0.934 190 23 508.0 40 428.0 1.059 200 26 453.4 40 373.4 1.280 注:S为行程;θ为F0与垂直方向夹角;F3为最小压缩力;Fr为最大动态摩擦力;F1为最小伸展力;k为气弹簧弹性系数。表3同此。Notes:S, stroke; θ, angle of F0 with vertical direction; F3, minimum compress force; Fr, maximum dynamic friction; F1, minimum extension force; k, gas spring modulus coefficient. Same as Tab.3. 表 3 气弹簧活塞杆直径与最小伸展力大小选择范围推荐表
Table 3 Recommended table of minimum extension force range and stroke range of gas spring
序号
No.活塞杆
直径 Diameter of piston rod/mm最小伸展力
Minimum extension force (F1)/N行程范围
Stroke range/mm推荐范围
Recommended
range可选范围
Optional
range1 6 50 ~ 250 50 ~ 350 50 ~ 400 2 8 200 ~ 450 100 ~ 700 100 ~ 700 3 10 300 ~ 700 100 ~ 1 200 150 ~ 1 100 4 12 450 ~ 1 000 150 ~ 1 500 150 ~ 1 600 5 14 600 ~ 1 400 200 ~ 2 500 1 600 ~ 2 200 6 20 1 250 ~ 3 100 1 000 ~ 5 200 2 200 ~ 4 500 注:表3引自文献[12]。Note: Tab.3 is cited from reference [12]. -
[1] 中华人民共和国建设部. 关于落实新建住房结构比例要求的若干意见[J]. 中国勘察设计, 2006(12):6−7. Ministry of Construction. Some opinions on the implementation of the requirements on the proportion of newly-built housing structure[J]. China Engineering & Consulting, 2006(12): 6−7.
[2] 张帆. 基于有限元法的实木框架式家具结构力学研究[D]. 北京: 北京林业大学, 2012. Zhang F. Research on structural mechanics of solid wood framework furniture based on finite element method[D]. Beijing: Beijing Forestry University, 2012.
[3] 张琦, 葛思擘. 新型支承−气弹簧的结构与特性分析[J]. 机械科学与技术, 1995, 14(6):85−89. Zhang Q, Ge S B. Analysis of structure and characteristics of a new type of supporting-air spring[J]. Mechanical Science and Technology for Aerospace Engineering, 1995, 14(6): 85−89.
[4] 王殿武. 四连杆铰链气弹簧助力式开启机构的设计[J]. 汽车技术, 1999(5):7−9. Wang D W. Design of four-link hinge air-spring assisted opening mechanism[J]. Automobile Technology, 1999(5): 7−9.
[5] 刘迎林, 张有忱, 秦柳, 等. 全塑车身后备箱气撑杆运动仿真[J]. 塑料, 2015, 44(2):104−107. doi: 10.3969/j.issn.1001-3539.2015.02.025 Liu Y L, Zhang Y C, Qin L, et al. Movtion simulation of all plastic body trunk pneumatic spring[J]. Plastics, 2015, 44(2): 104−107. doi: 10.3969/j.issn.1001-3539.2015.02.025
[6] 王定虎. 汽车背门气撑杆布置校核研究[J]. 内燃机与配件, 2018(22):99−101. doi: 10.3969/j.issn.1674-957X.2018.22.051 Wang D H. Research on the layout check of gas strut on the back door of automobile[J]. Internal Combustion Engine & Parts, 2018(22): 99−101. doi: 10.3969/j.issn.1674-957X.2018.22.051
[7] 国家质检总局(CN−GB). 压缩气弹簧技术条件: GB 25751—2010[S]. 北京: 中国标准出版社, 2010. National Bureau of Quality Inspection (CN−GB). Compression gas spring technical specification: GB 25751—2010[S]. Beijing: Standards Press of China, 2010.
[8] 李亮辉. 浅析工程机械用气弹簧的设计选型[J]. 工程机械, 2013, 44(6):37−39. doi: 10.3969/j.issn.1000-1212.2013.06.009 Li L H. Design and selection of gas spring for construction machinery[J]. Construction Machinery and Equipment, 2013, 44(6): 37−39. doi: 10.3969/j.issn.1000-1212.2013.06.009
[9] 谭能堃, 王治杰. SUV掀背门气撑杆布置与优化[J]. 科技传播, 2013, 5(7):94−95. Tan N K, Wang Z J. Layout and optimization of pneumatic brace for SUV hatchback door[J]. Public Communication of Science & Technology, 2013, 5(7): 94−95.
[10] 国家质量技术监督局. 弹簧术语: GB/T 1805—2001[S]. 北京: 中国标准出版社, 2001. The State Bureau of Quality and Technical Supervision. Nomenclature of springs: GB/T 1805—2001[S]. Beijing: Standards Press of China, 2001.
[11] 蒲蕾, 杨明华. 气弹簧在汽车后背门上的优化布置[J]. 汽车工程师, 2016(2):47−50. doi: 10.3969/j.issn.1674-6546.2016.02.010 Pu L, Yang M H. Optimal arrangement of air spring on the back door of automobile[J]. Auto Engineer, 2016(2): 47−50. doi: 10.3969/j.issn.1674-6546.2016.02.010
[12] 行业标准−机械(CN−JB). 气弹簧设计计算: JB/T 10418—2004[S]. 北京: 中国标准出版社, 2004. Industry standard−Machinery (CN−JB). Gas springs specification: JB/T 10418—2004[S]. Beijing: Standards Press of China, 2004.
-
期刊类型引用(3)
1. 郭家伟,赵民,王佐,王小甜. 辅助站立坐便器设计与试验. 机械设计与制造. 2024(04): 95-99 .  百度学术
百度学术
2. 水东莉. 独轮手推车式挖坑机结构设计. 辽宁农业职业技术学院学报. 2020(06): 1-3 .  百度学术
百度学术
3. 林锐航,李晓增,欧雪冰,李新兵,杜莹,何国山. 壁柜式翻转床五金结构特点分析. 林业和草原机械. 2020(06): 17-22 .  百度学术
百度学术
其他类型引用(0)



 下载:
下载: